Phase Noise and Jitter Simulation

Posted on Edited on In

Inspection of Phase Noise

How to Identify the Source of Phase Jitter through Phase Noise Plots [https://www.sitime.com/company/newsroom/blog/how-identify-source-phase-jitter-through-phase-noise-plots]

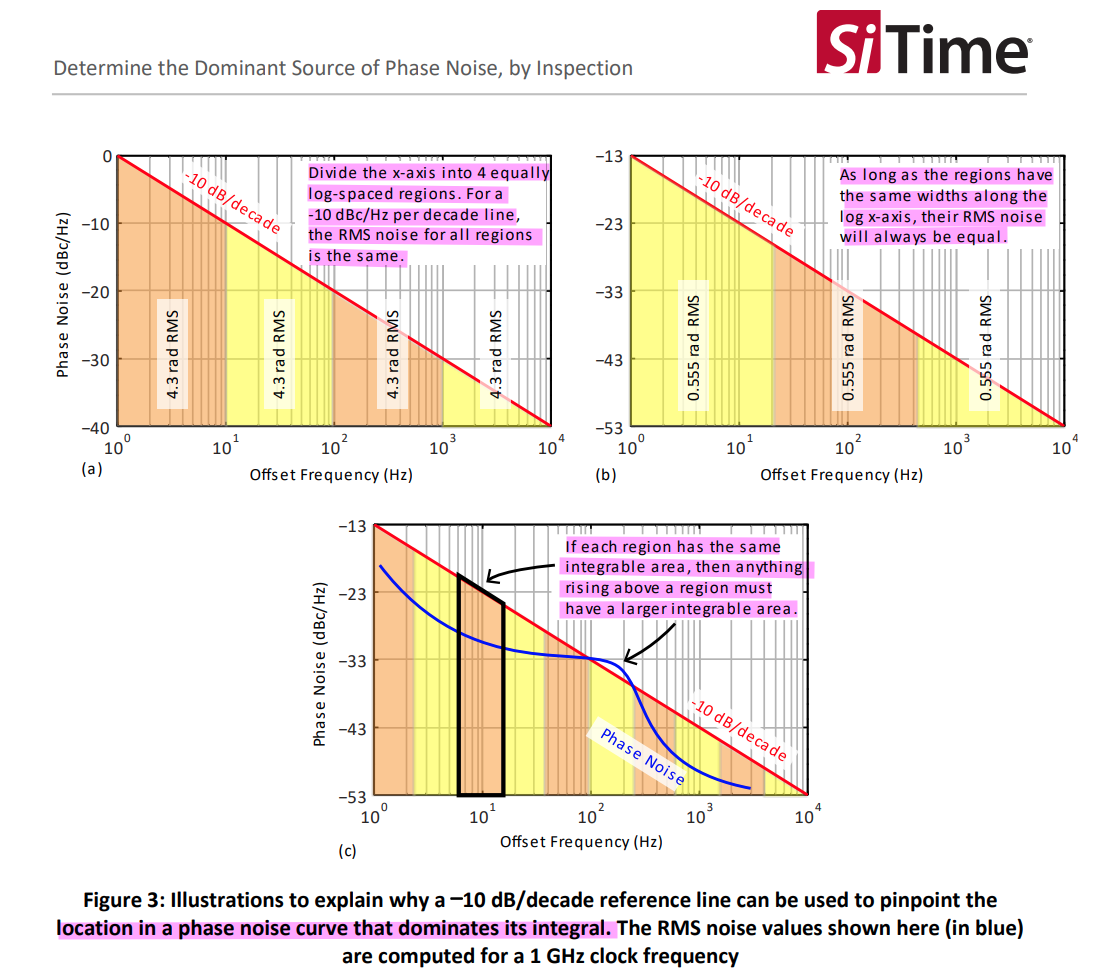
AN10072 Determine the Dominant Source of Phase Noise, by Inspection [https://www.sitime.com/support/resource-library/application-notes/an10072-determine-dominant-source-phase-noise-inspection]

4-minute Clinic: Determine the Dominant Source of Jitter by Inspection of Phase Noise Plot [https://youtu.be/2elHk3v45Pk]

a -10 dB/decade reference line can be used to pinpoint the location in a phase noise curve that dominates its integral

image-20250720154715877

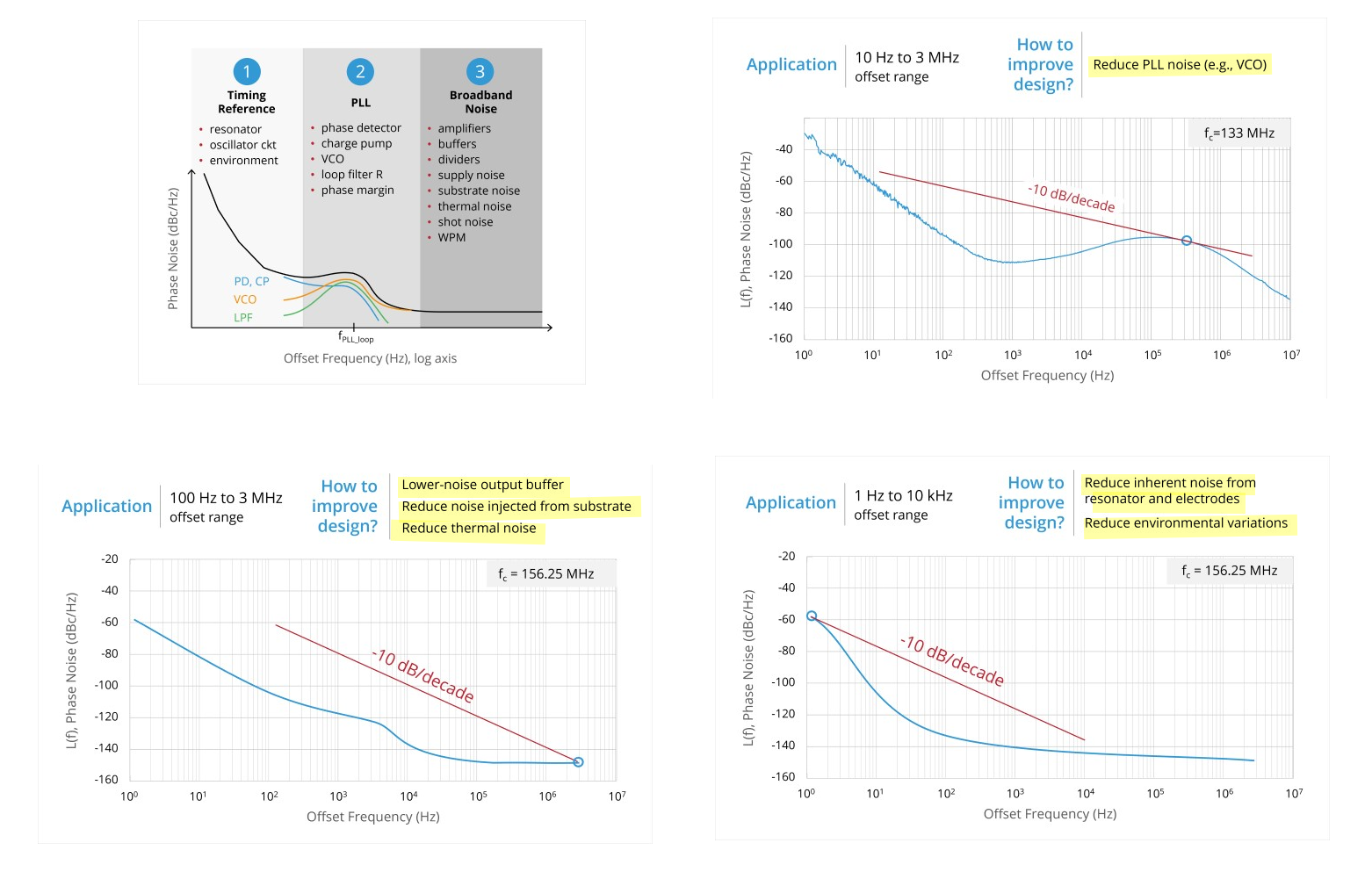
image-20250720155328050

Reference-Clock Phase Noise in PLL

Gary Giust. How to Evaluate Reference-Clock Phase Noise in High-Speed Serial Links [expanded version], [compact version]

G. Richmond, "Refclk Fanout Best Practices for 8GT/s and 16GT/s Systems," PCI-SIG Developers Conference, June 7, 2017

Knowing how input phase noise aliases when sampled by a PLL

image-20250719113300286

An alternate view of phase noise aliasing during the sampling process

  • Instead of mirroring the jitter-transfer function located below \(F_S/2\) across spectral boundaries located at integer multiples of \(F_S/2\) (i.e. 50 MHz) as shown in Figure 2 (a)
  • we could alternatively fold the portion of the Raw Data curve located above \(F_S/2\) across these spectrum boundaries to appear below \(F_S/2\) as shown in Figure 2 (b)

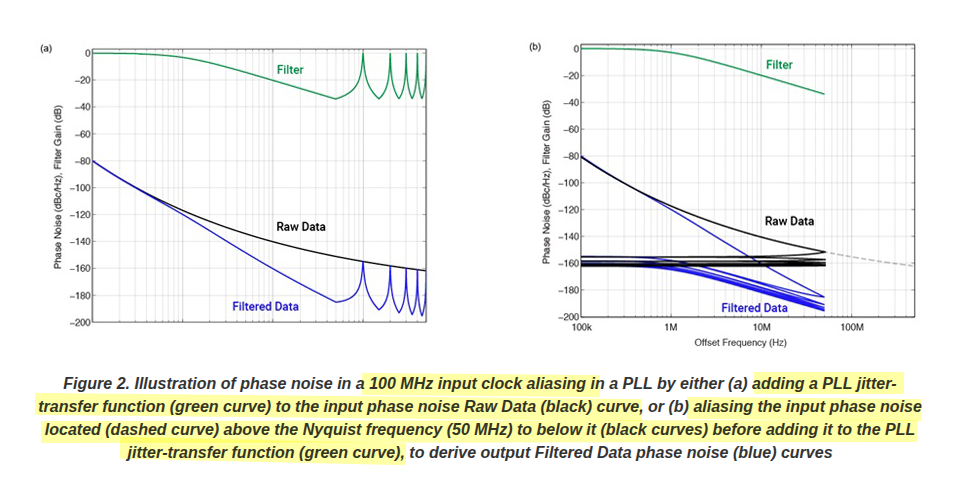
image-20250719120321878

Integrating the combined area under each Filtered Data curve shown in Figure 2 (b) is mathematically equivalent to integrating the entire Filtered Data curve shown in Figure 2 (a)

image-20250720075655909

Phase Noise Analyzer vs TIE jitter using Real-time Oscilloscope

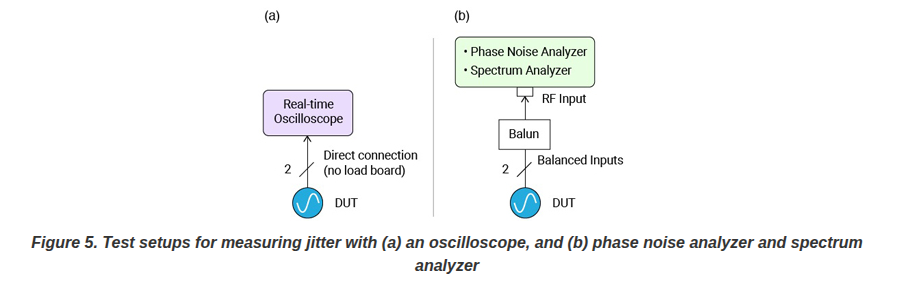
image-20250720083138045

Since an oscilloscope observes jitter similar to a real system, we regard its result as the gold standard against which other methods may be judged

Flat Phase Noise Extension to twice the clock frequency

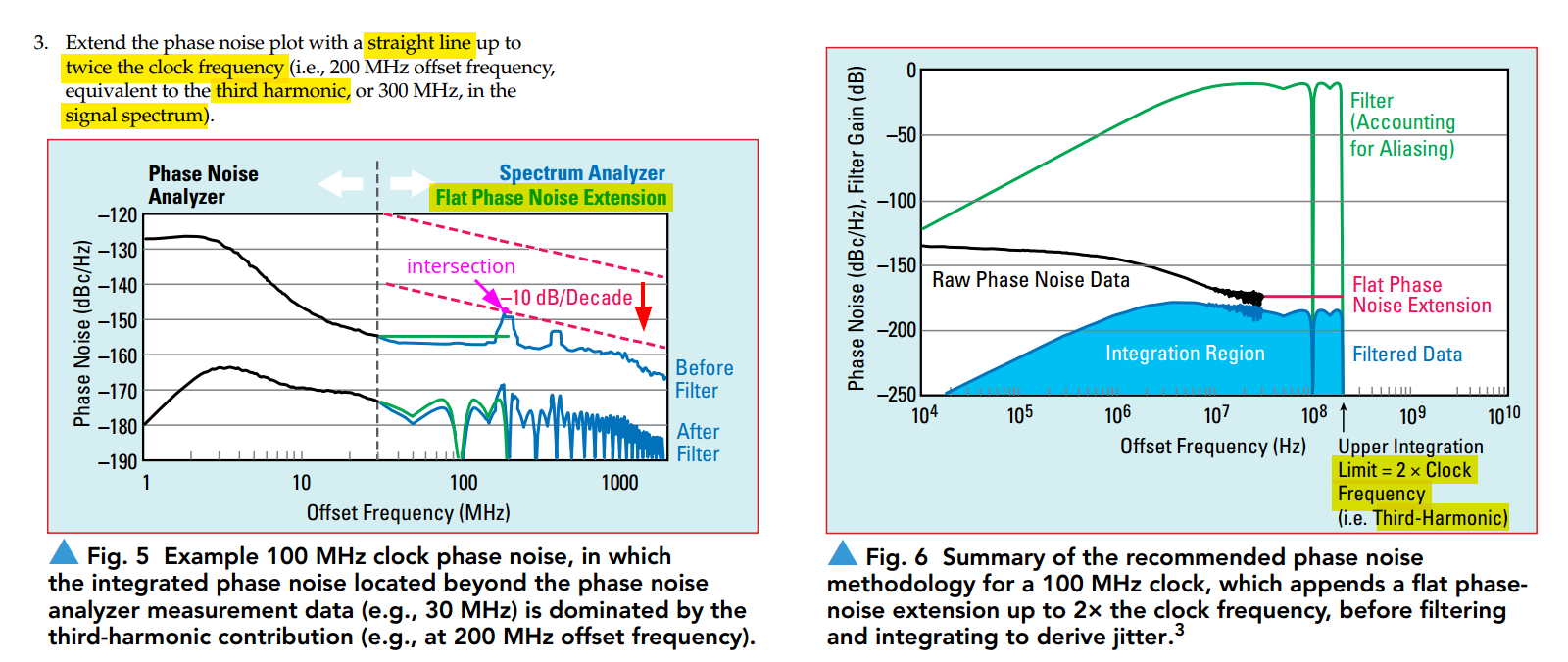
image-20250720090755239

Phase Noise Aliasing & Integration Limits

These two types of measurements deliver the same rms jitter of \(f_{CK}\)

  • both rising and falling: integrated from \(-f_{CK}\) to \(+f_{CK}\)
  • only the rising (or falling) edges: integrated from \(-f_{CK}/2\) to \(+f_{CK}/2\)

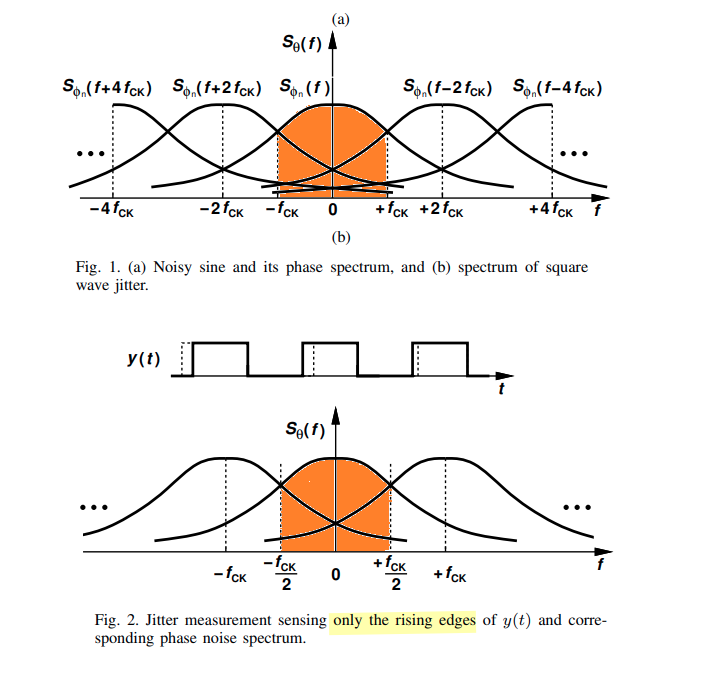
image-20250524074831161

image-20250523221143537

temporal autocorrelation and Wiener-Khinchin theorem is more appropriate to arise rms value

Y. Zhao and B. Razavi, "Phase Noise Integration Limits for Jitter Calculation,"[https://www.seas.ucla.edu/brweb/papers/Conferences/YZ_ISCAS_22.pdf]

G. Giust, "Phase Noise Aliases as TIE Jitter," Signal Integrity Journal, July 23, 2018 [https://www.signalintegrityjournal.com/articles/912-phase-noise-aliases-as-tie-jitter]

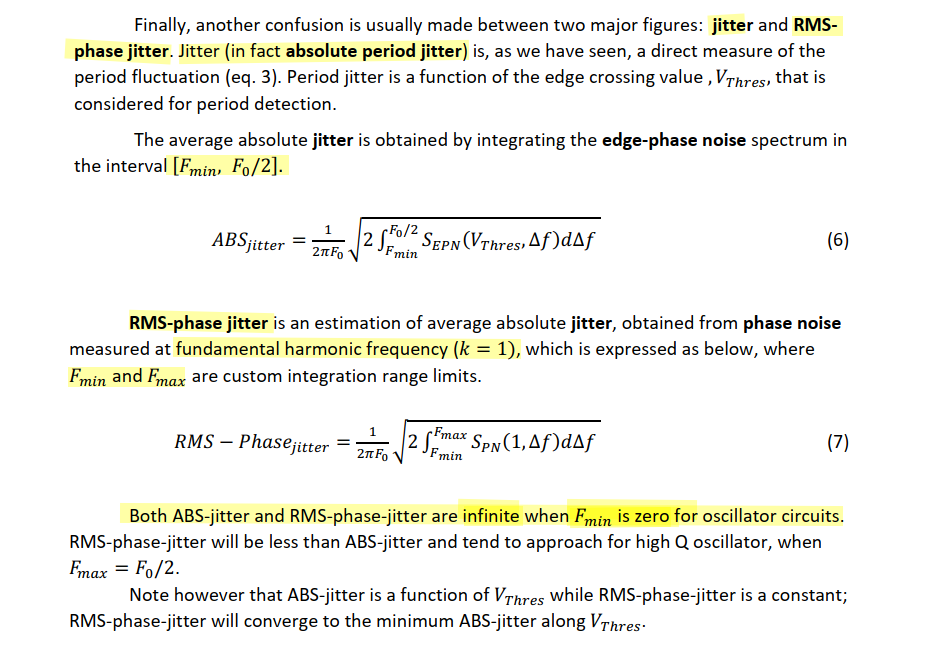
Jitter and Edge phase noise

[https://community.cadence.com/cadence_technology_forums/f/custom-ic-design/56929/how-to-derive-edge-phase-noise-from-output-noise-in-sampled-pnoise-simulation/1388888]

Shawn Logan, Summary of Study of Cadence Sampled Phase Noise and Jitter Definitions with a Comparison to Conventional Time Interval Error (TIE) for a Driven Circuit [www.dropbox.com/s/3m531dl4fl7bwbr/jee_computation_example_sml_032823v1p0.pdf]

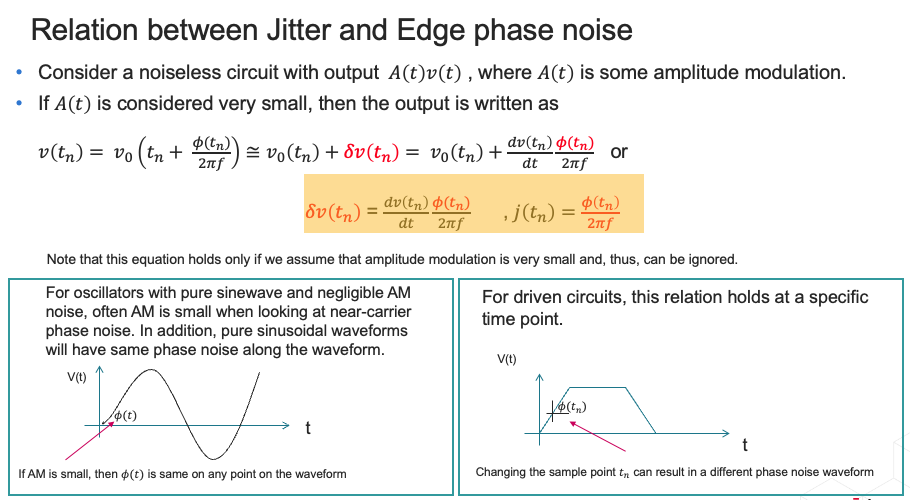
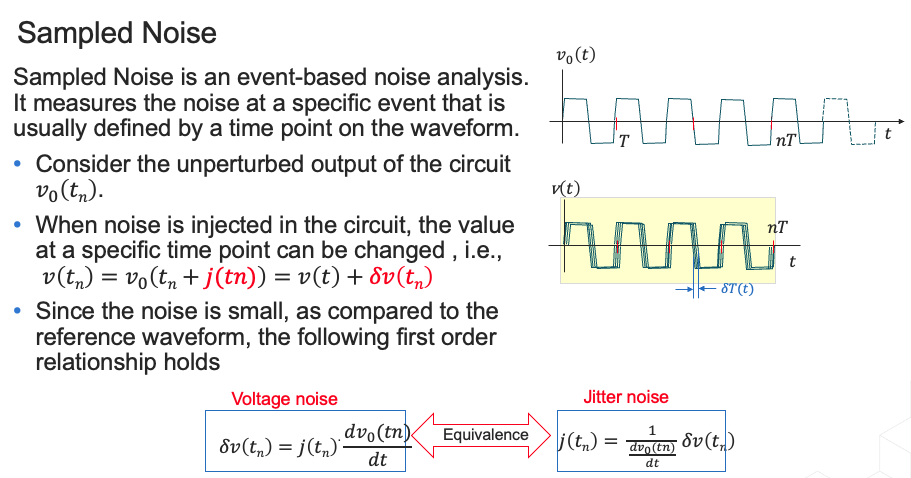
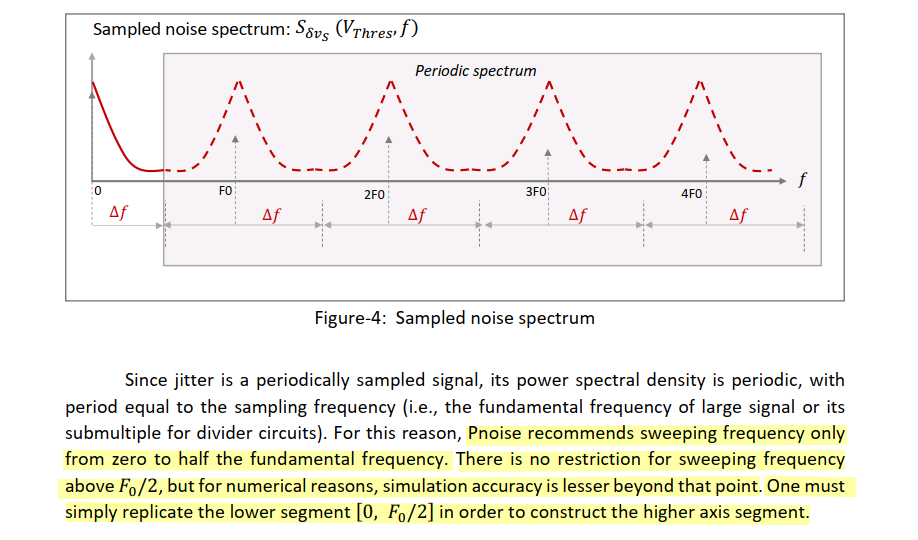
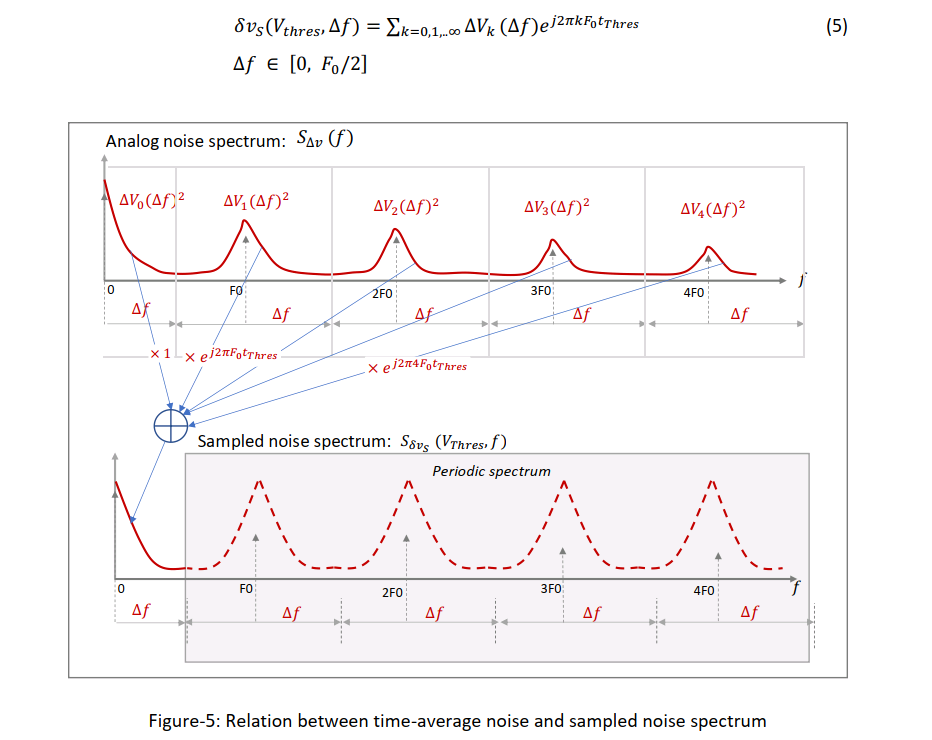
timeaverage noise (phase-noise) & sampled noise (edge-phase noise or jitter) spectrum

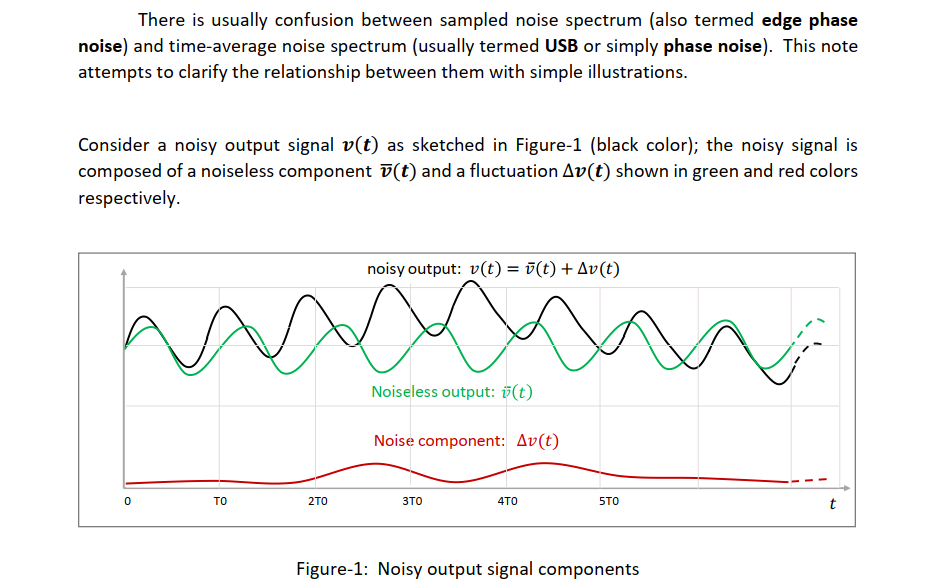
image-20250530203348622

Time-average noise analysis

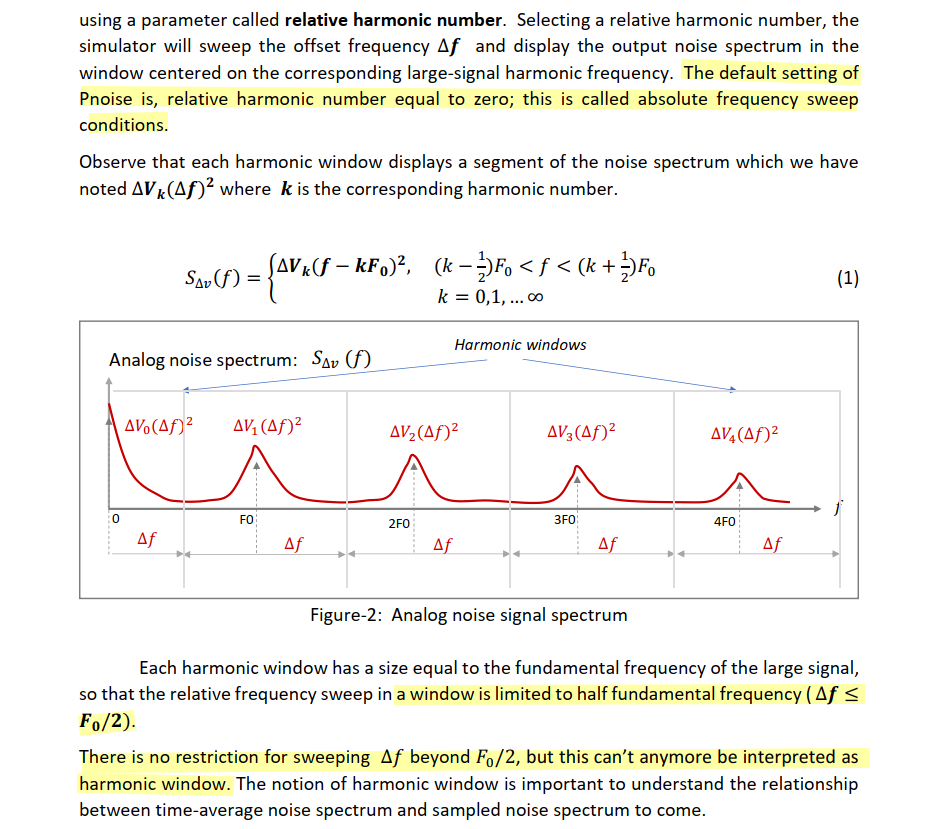
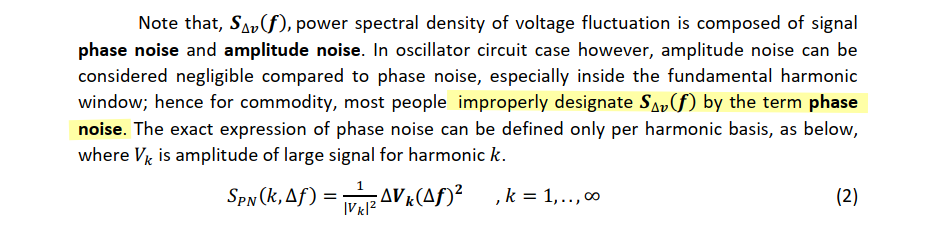
image-20250530203720538image-20250530204820891

\(S_{\Delta f}(f)\) between \([\Delta f, F_0/2]\) may be less than that of other harmonic window

Sampled noise analysis

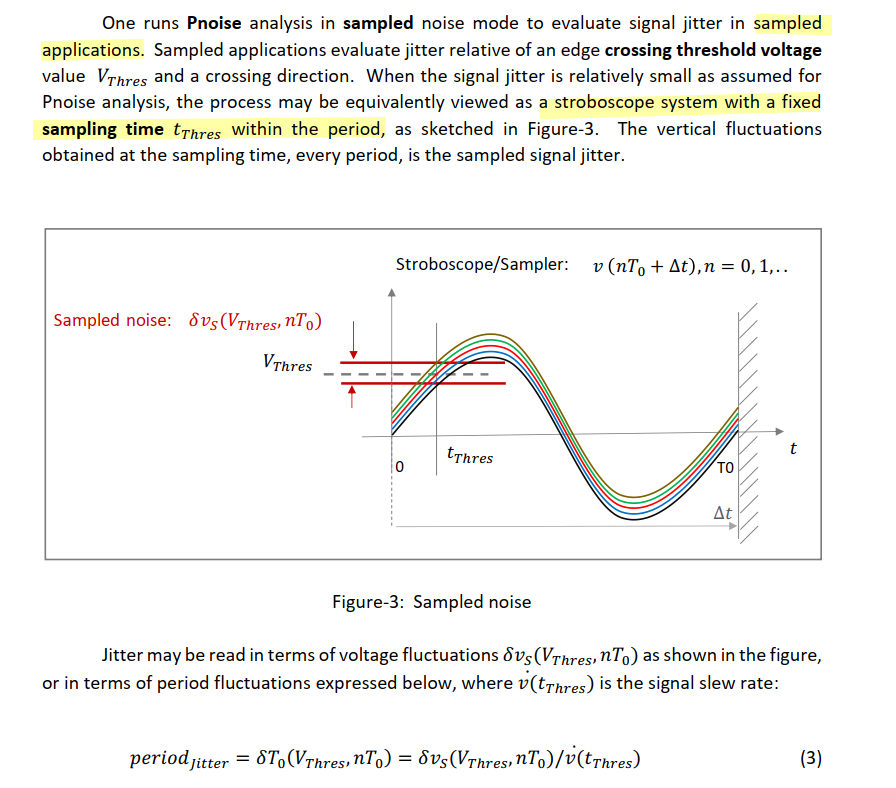
image-20250530204030405

image-20250530204222221

correlation

image-20250530205849830

image-20250530205919247

image-20250530210543667

VCO Phase Noise

pnoise - timeaverage

  1. Direct Plot/Pnoise/Phase Noise or

    image-20220511112856934

  2. manually calculate by definition

    image-20220511112806122

  3. output noise with unit dBc

    Direct Plot/Pnoise/Output Noise Units:dBc/Hz and Noise convention: SSB

    image-20220511113248984

The above method 2 and 3 only apply to timeaveage pnoise simulation,

pnoise - sampled(jitter)/Edge Crossing

EdgePhaseNoise.drawio

Direct Plot/Pnoise/Edge Phase Noise or

image-20220515214901120

Another way, the following equation can also be used for sampled(jitter)/Edge Crossing

1
PhaseNoise(dBc/Hz) = dB20( OutputNoise(V/sqrt(Hz)) / slopeCrossing / Tper*twoPi ) - dB10(2)

where dB10(2) is used to obtain SSB from DSB

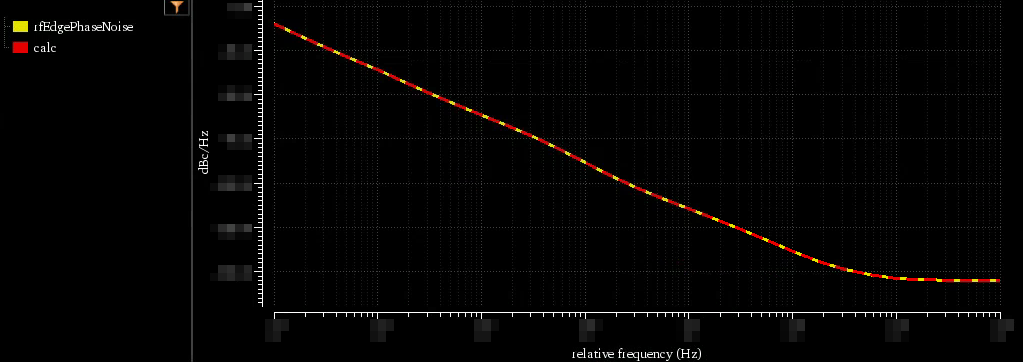
image-20220511150337318

Output Noise of sampled(jitter) pnoise

The last section's Output Noise (V**2/Hz) can be obtained by transient noise simulation

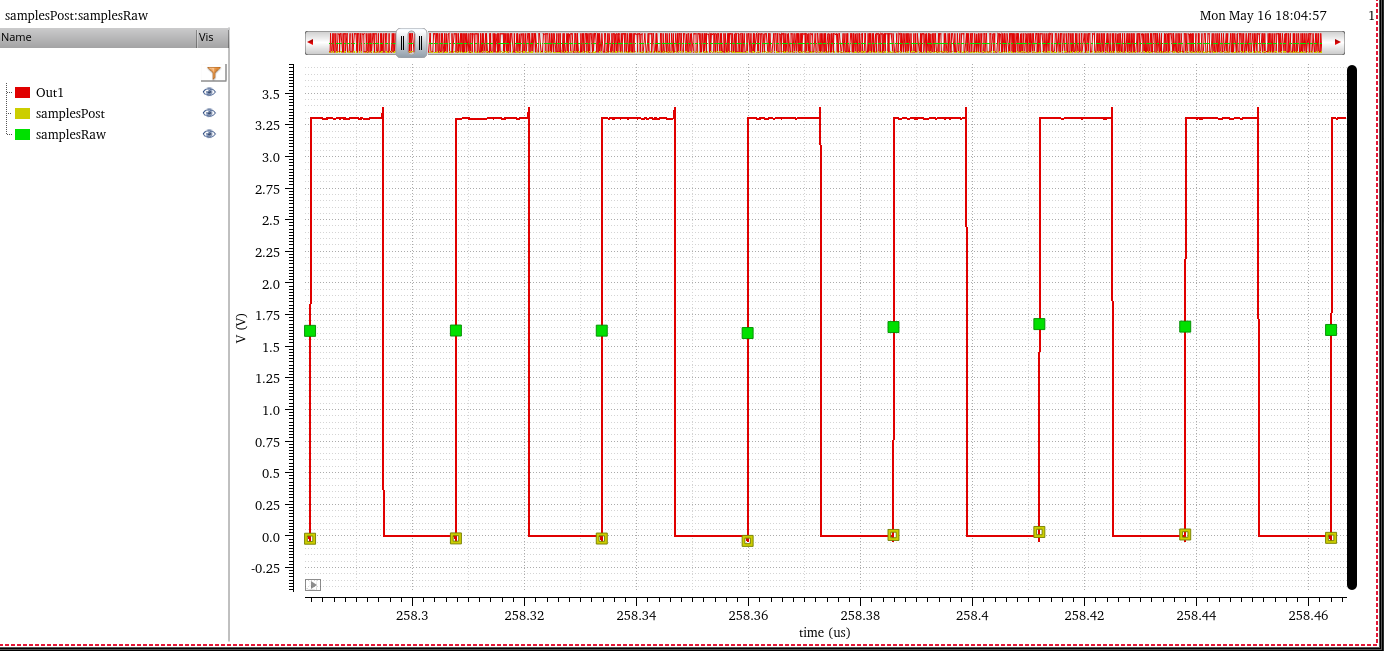
The idea is that sample waveform with ideal clock, subtract DC offset, then fft(psd)

  • samplesRaw = sample(wv)
  • samplePost = samplesRaw - average(samplesRaw)
  • Output Noise (V**2/Hz) = psd(samplePost)

image-20220516184543148

image-20220516184844296

Expression:

image-20220516185506348

The computation cost is typically very high, and the accuracy is lesser as compared to PSS/Pnoise

Pnoise Sampled(jitter): Sampled Phase Option

  • Identical to noisetype=timedomain in old GUI
  • Use model:
    • Sampleds Per Period: number of ponits
    • Add Specific Points: specific time point, still time points

image-20220712085426461

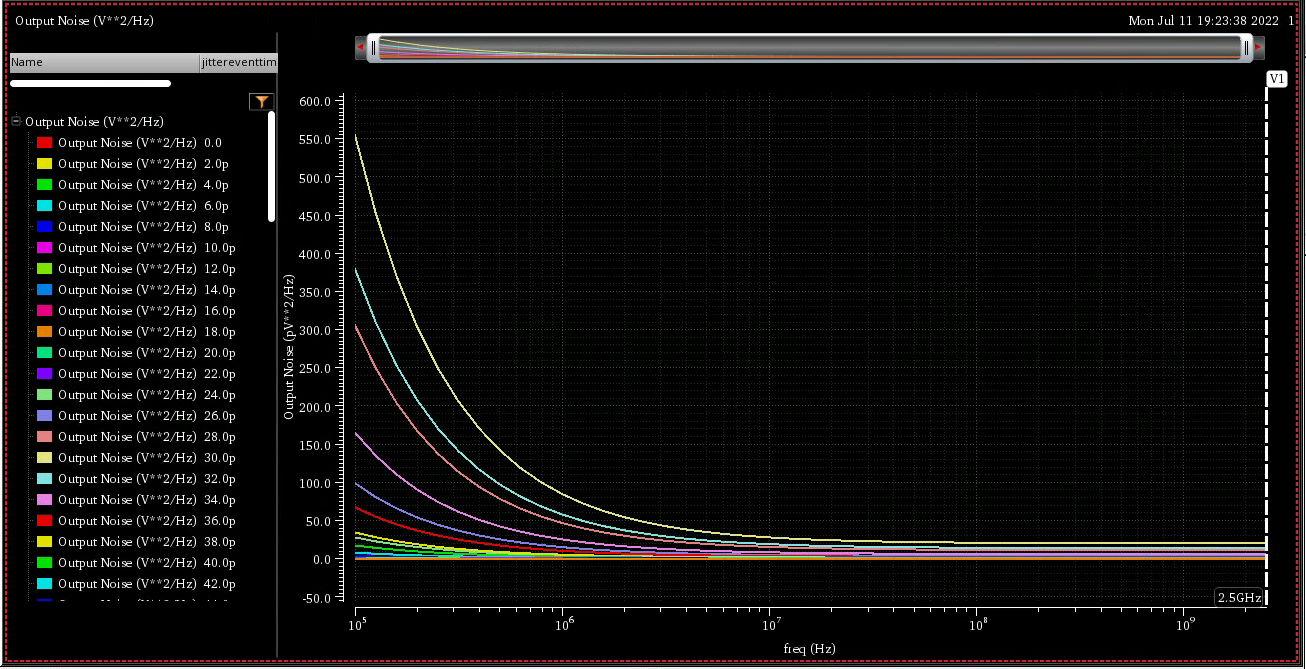
image-20220712085836315

image-20220712090011204

pss beat freq = 5GHz

pnoise sweeptype: absolute, from 100k to 2.5GHz

Transient noise

phase noise from transient noise analysis

  1. The Phase Noise function is now available in the Direct Plot form (Results-Direct Plot-Main Form) after Transient Analysis is run
    • Absolute jitter Method
    • Direct Power Spectral Density Method
  2. PN phase noise function
    • Absolute jitter Method
    • Direct Power Spectral Density Method

Absolute jitter Method: Phase noise is defined as the power spectral density of the absolute jitter of an input waveform

and absolute jitter method is the default method

In below discussion, we only think about the absolute jitter method

PSD and Phase Noise

  • phase noise is single-sideband
  • psd is double-sideband
  • Then the ratio is 2

By PSS_Pnoise

jee

1
rfEdgePhaseNoise(?result "pnoise_sample_pm0" ?eventList 'nil) + 10 * log10(2)

convert single-sideband phase noise to psd by multiplying 2 or 10 * log10(2)

By trannoise PN function

1
PN(clip(VT("/Out1") 2.60417e-08 0.000400052) "rising" 1.65 ?Tnom (1 / 3.84e+07) ?windowName "Rectangular" ?smooth 1 ?windowSize 15000 ?detrending "None" ?cohGain 1 ?methodType "absJitter")

double-sideband psd

By trannoise psd and abs_jitter function

1
dB10(psd(abs_jitter(clip(VT("/Out1") 2.60417e-08 0.000400052) "rising" 1.65 ?Tnom (1 / 3.84e+07)) 2.60417e-08 0.000400052 15360 ?windowName "Rectangular" ?smooth 1 ?windowSize 15000 ?detrending "None" ?cohGain 1))

double-sideband psd

abs_jitter Y-Unit default is rad

Comparison

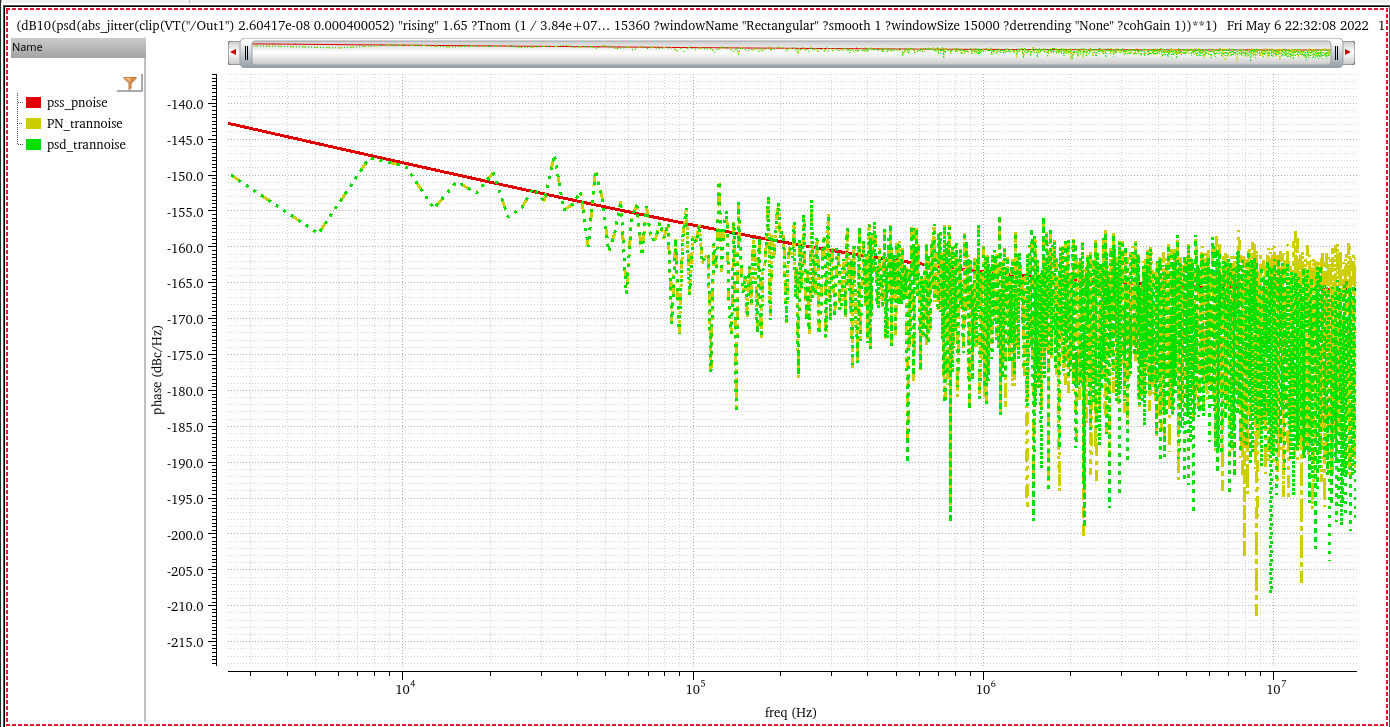
image-20220506225324377

PN's result is same with psd's

RMS value

  • build the abs_jitter function with seconds as the Y axis and add the stddev function to determine the Jee jitter value
  • or integrate psd

The RMS \(x_{\text{RMS}}\) of a discrete domain signal \(x(n)\) is given by \[ x_{\text{RMS}}=\sqrt{\frac{1}{N}\sum_{n=0}^{N-1}|x(n)|^2} \] Inserting Parseval's theorem given by \[ \sum_{n=0}^{N-1}|x(n)|^2=\frac{1}{N}\sum_{n=0}^{N-1}|X(k)|^2 \] allows for computing the RMS from the spectrum \(X(k)\) as \[ x_{\text{RMS}}=\sqrt{\frac{1}{N^2}\sum_{n=0}^{N-1}|X(k)|^2} \]

Remarks

Cadence Spectre's PN function may call abs_jitter and psd function under the hood.

Phase Noise in vsource

Suppose pnoise result of one block is shown as below, and the result is stimulus of following block

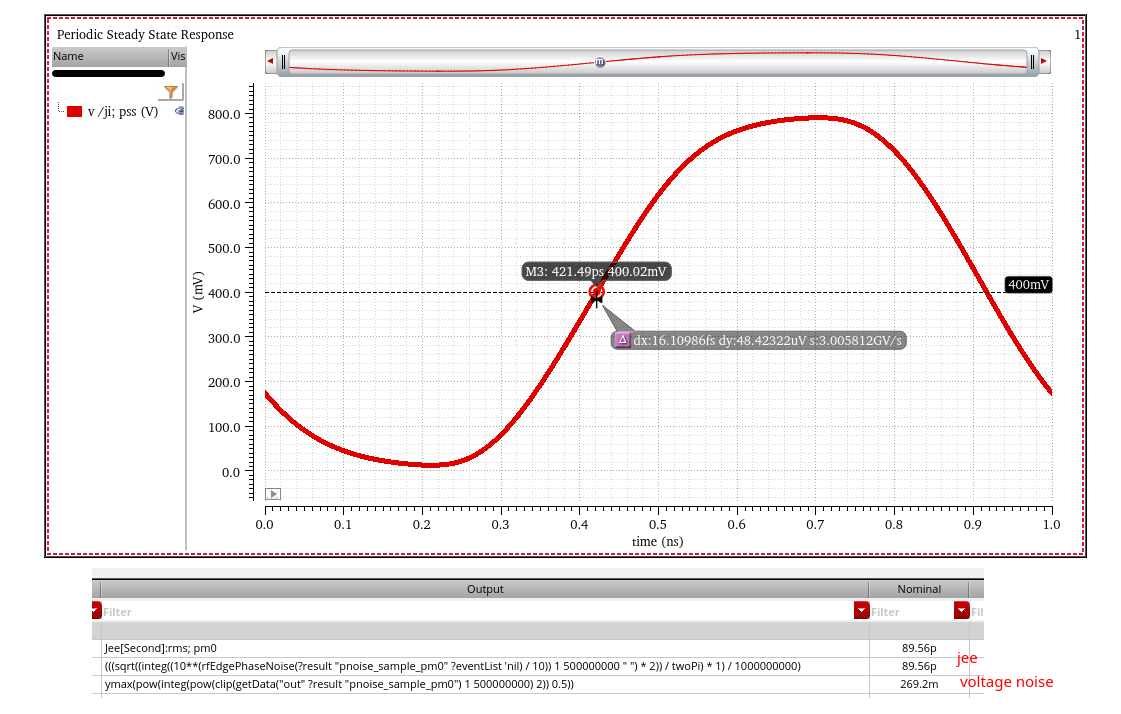
image-20250929215408888

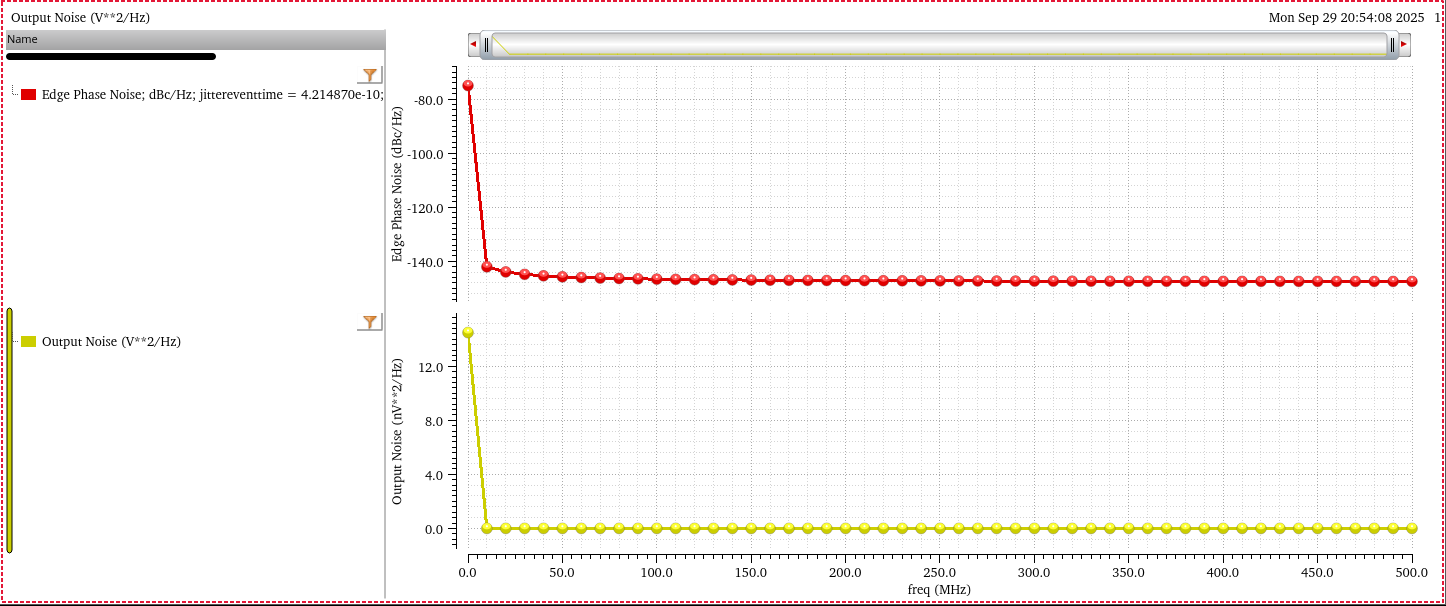
image-20250929215620135

First export Output Noise and Edge Phase Noise， then select noiseModelType and noisefile respectively

image-20250929224438491

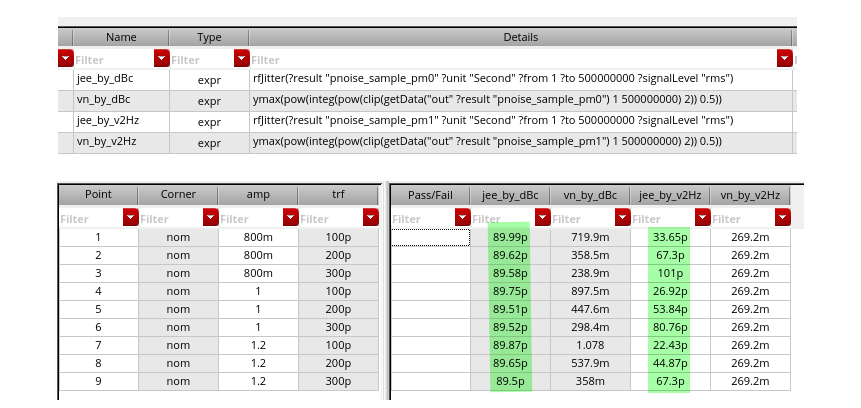
image-20250929221228963

Under vsource (Source type: pulse) with different amplitude & rising/falling time, simulation result demonstrate that Edge Phase Noise(dBc) maintain jitter or phase noise by tweaking voltage noise at edge under the hoods, however Noise Voltage(V^2/Hz) maintain voltage noise

In the conclusion, Edge Phase Noise(dBc) is preferred for phase noise evaluation

notice:

@(#)$CDS: spectre version 21.1.0 64bit 12/01/2023 07:24 (csvcm36c-1) $

@(#)$CDS: virtuoso version ICADVM20.1-64b 10/11/2023 09:26 (cpgbld01) $

SSB Phase Noise (dBc)

image-20250930185936617

image-20250930190216011

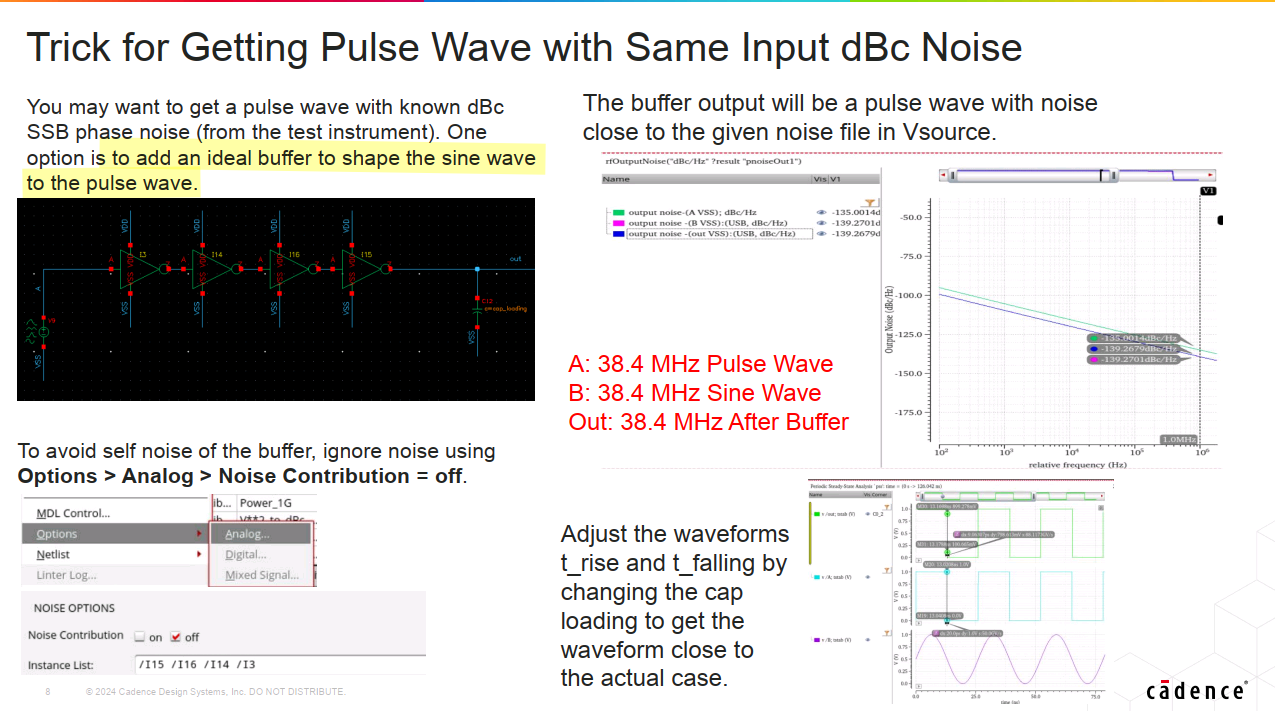
image-20250930190841282

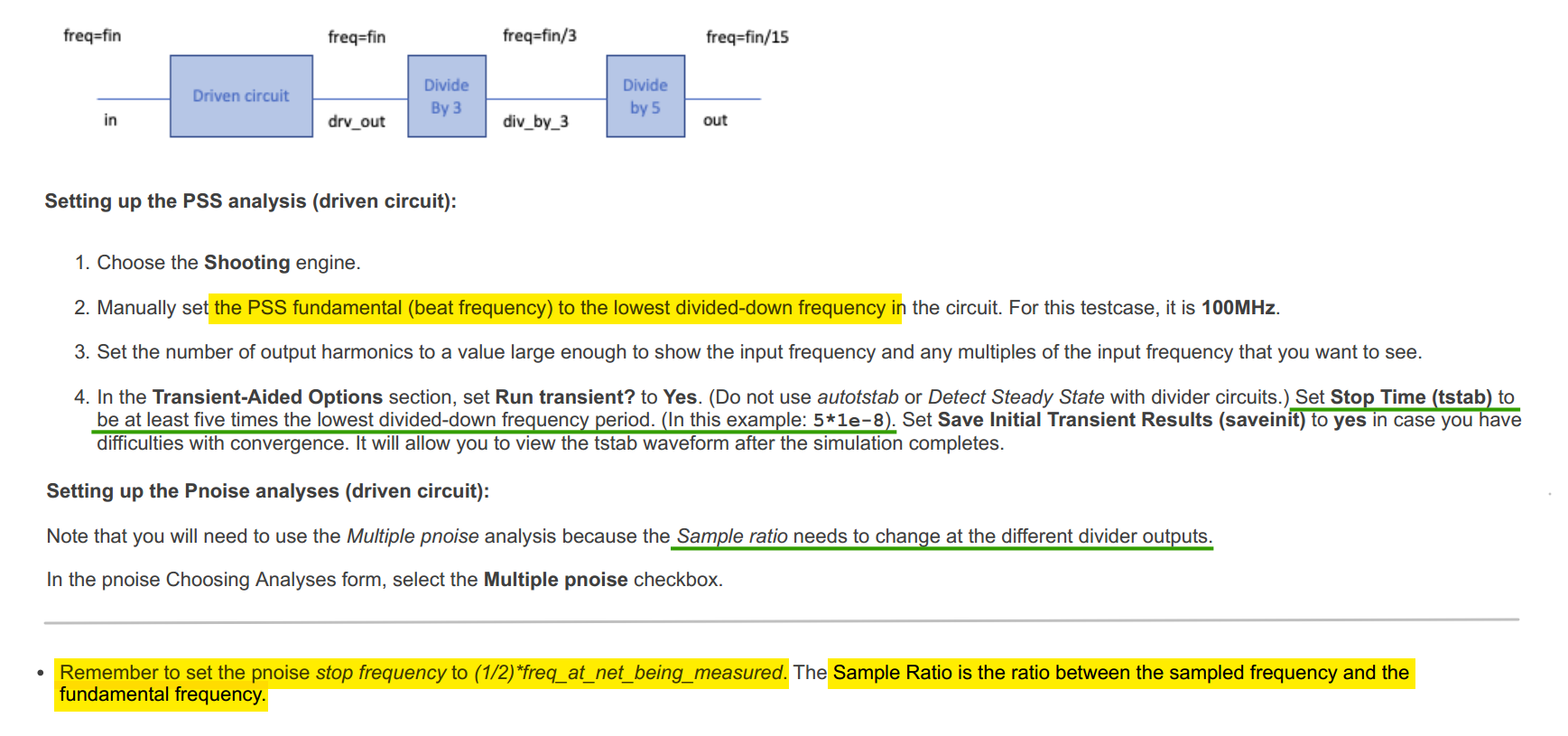
Divider PN simulation

Cadence Support. "How to set up pss/pnoise when simulating a driven circuit or a VCO, both containing dividers"

image-20250930200829603

reference

Article (11514536) Title: How to obtain a phase noise plot from a transient noise analysis URL: https://support.cadence.com/apex/ArticleAttachmentPortal?id=a1Od0000000nb1CEAQ

Article (20500632) Title: How to simulate Random and Deterministic Jitters URL: https://support.cadence.com/apex/ArticleAttachmentPortal?id=a1O3w000009fiXeEAI

Cadence, Application Note: Understanding the relations between time-average noise (phase-noise) and sampled noise (edge-phase noise or jitter) in Pnoise analysis

Tutorial on Scaling of the Discrete Fourier Transform and the Implied Physical Units of the Spectra of Time-Discrete Signals Jens Ahrens, Carl Andersson, Patrik Höstmad, Wolfgang Kropp URL: https://appliedacousticschalmers.github.io/scaling-of-the-dft/AES2020_eBrief/

Tawna, "Modeling Oscillators with Arbitrary Phase Noise Profiles"[https://community.cadence.com/cadence_blogs_8/b/rf/posts/modeling-oscillators-with-arbitrary-phase-noise-profiles]

—, "How to Specify Phase Noise as an Instance Parameter in Spectre Sources (e.g. vsource, isource, Port)" [https://community.cadence.com/cadence_blogs_8/b/rf/posts/how-to-specify-phase-noise-as-an-instance-parameter-in-spectre-sources-e-g-vsource-isource-port]