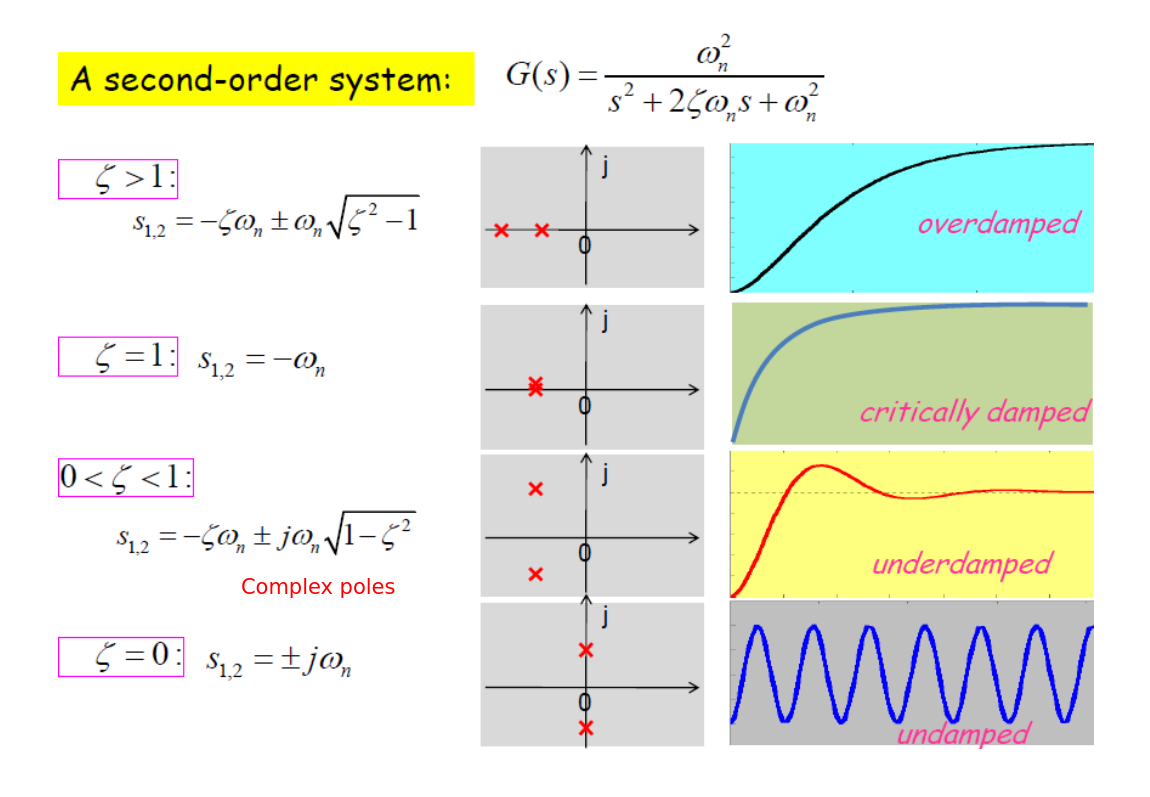
Second-Order System

Posted on Edited on In

overview

image-20240721172721884

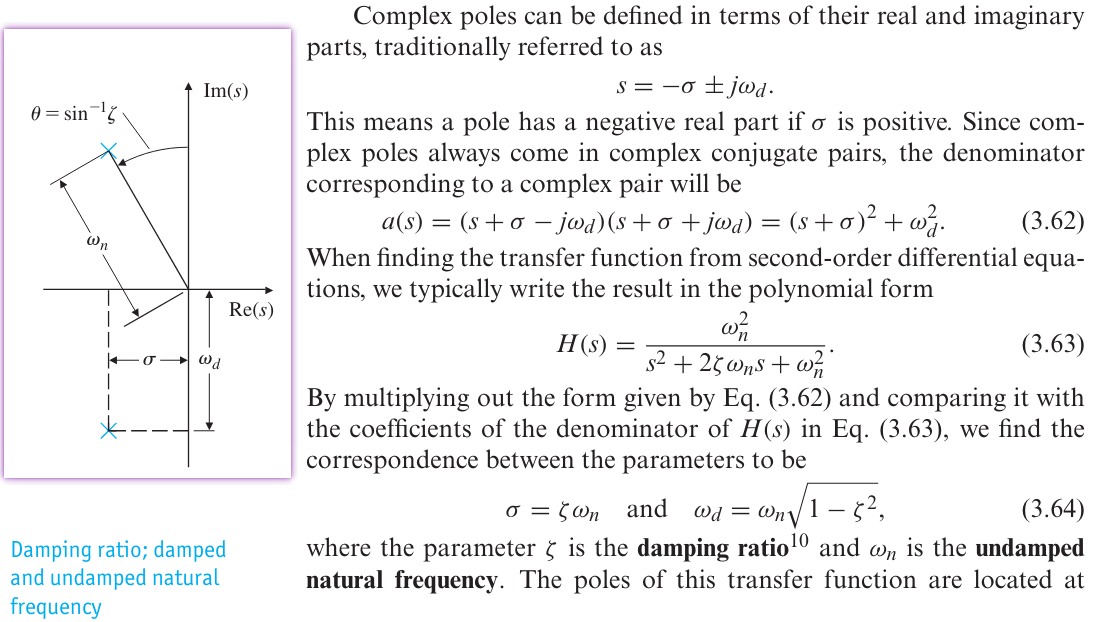
image-20240629140001275

\(\omega_d\) called damped natural frequency

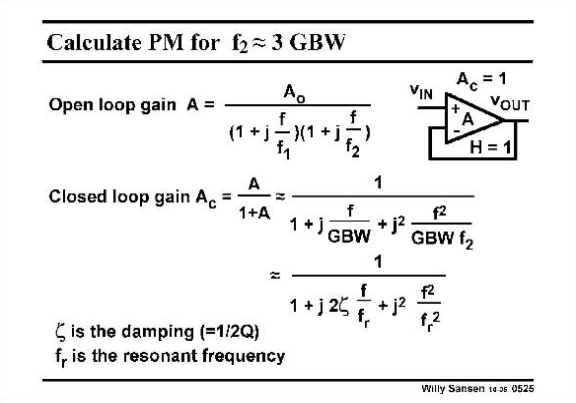
closed loop frequency response

image-20240629134127219 \[\begin{align} A &= \frac{\frac{A_0}{(1+s/\omega_1)(1+s/\omega_2)}}{1+\beta \frac{A_0} {(1+s/\omega_1)(1+s/\omega_2)}} \\ &= \frac{A_0}{1+A_0 \beta}\frac{1}{\frac{s^2}{\omega_1\omega_2(1+A_0\beta)}+\frac{1/\omega_1+1/\omega_2}{1+A_0\beta}s+1} \\ &\simeq \frac{A_0}{1+A_0 \beta}\frac{1}{\frac{s^2}{\omega_u\omega_2}+\frac{1}{\omega_u}s+1} \\ &= \frac{A_0}{1+A_0 \beta}\frac{\omega_u\omega_2}{s^2+\omega_2s+\omega_u\omega_2} \end{align}\]

That is \(\omega_n = \sqrt{\omega_u\omega_2}\) and \(\zeta = \frac{1}{2}\sqrt{\frac{\omega_2}{\omega_u}}\)

where \(\omega_u\) is the unity gain bandwidth

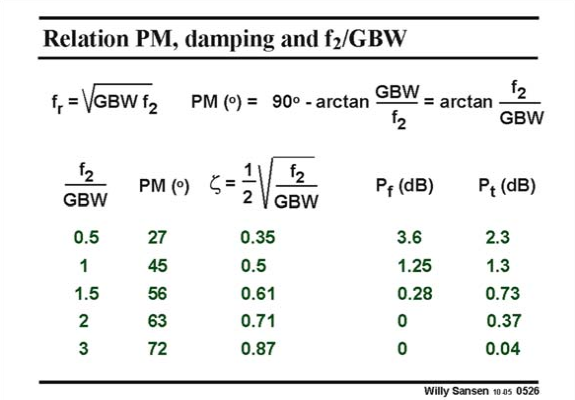
image-20240629112429803

where \(f_r\) is resonant frequency, \(\zeta\) is damping ratio, \(P_f\) maximum peaking, \(P_t\) is the peak of the first overshoot (step response)

image-20240629142324982

damping factor & phase margin

  • phase margin is defined for open loop system

  • damping factor (\(\zeta\)) is defined for close loop system

The roughly 90 to 100 times of damping factor (\(\zeta\)​) is phase margin \[ \mathrm{PM} = 90\zeta \sim 100\zeta \] In order to have a good stable system, we want \(\zeta > 0.5\) or phase margin more than \(45^o\)

We can analyze open loop system in a better perspective because it is simpler. So, we always use the loop gain analysis to find the phase margin and see whether the system is stable or not.

additional Zero

\[\begin{align} TF &= \frac{s +\omega_z}{s^2+2\zeta \omega_ns+\omega_n^2} \\ &= \frac{\omega _z}{\omega _n^2}\cdot \frac{1+s/\omega _z}{1+s^2/\omega_n^2+2\zeta s/\omega_n} \end{align}\]

Let \(s=j\omega\) and omit factor, \[ A_\text{dB}(\omega) = 10\log[1+(\frac{\omega}{\omega _z})^2] - 10\log[1+\frac{\omega^4}{\omega_n^4}+\frac{2\omega^2(2\zeta ^2 -1)}{\omega_n^2}] \] peaking frequency \(\omega_\text{peak}\) can be obtained via \(\frac{d A_\text{dB}(\omega)}{d\omega} = 0\) \[ \omega_\text{peak} = \omega_z \sqrt{\sqrt{(\frac{\omega_n}{\omega_z})^4 - 2(\frac{\omega_n}{\omega_z})^2(2\zeta ^2-1)+1} - 1} \]

Settling Time

single-pole

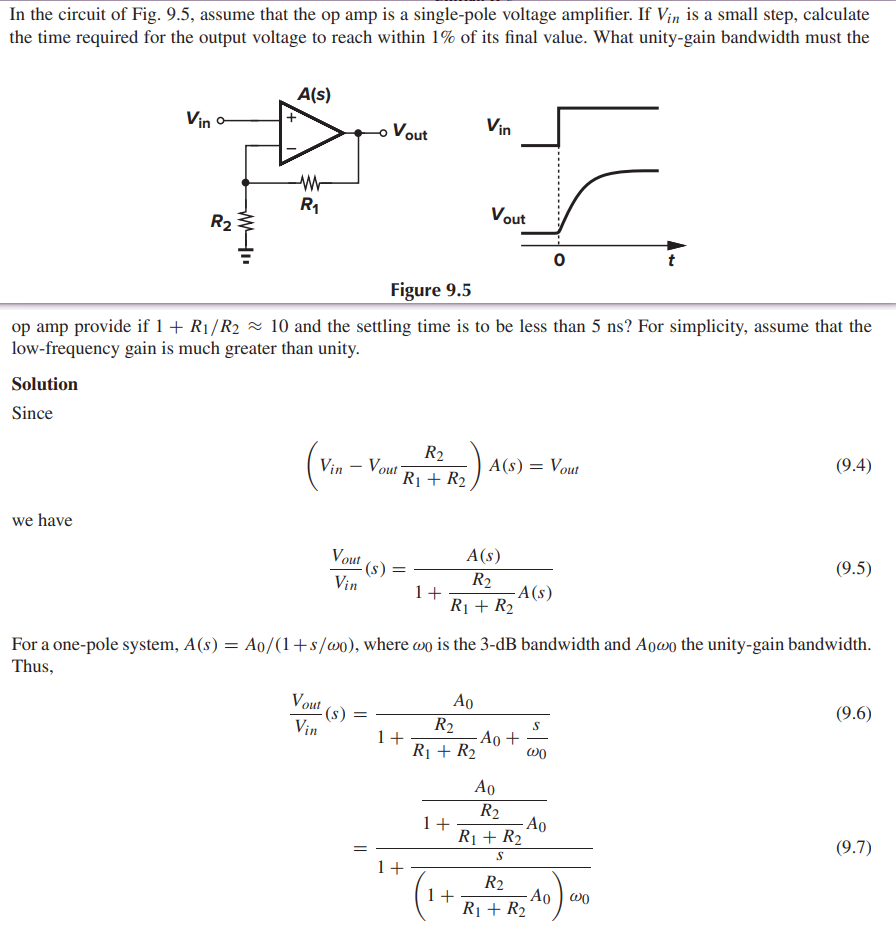
image-20240725204501781

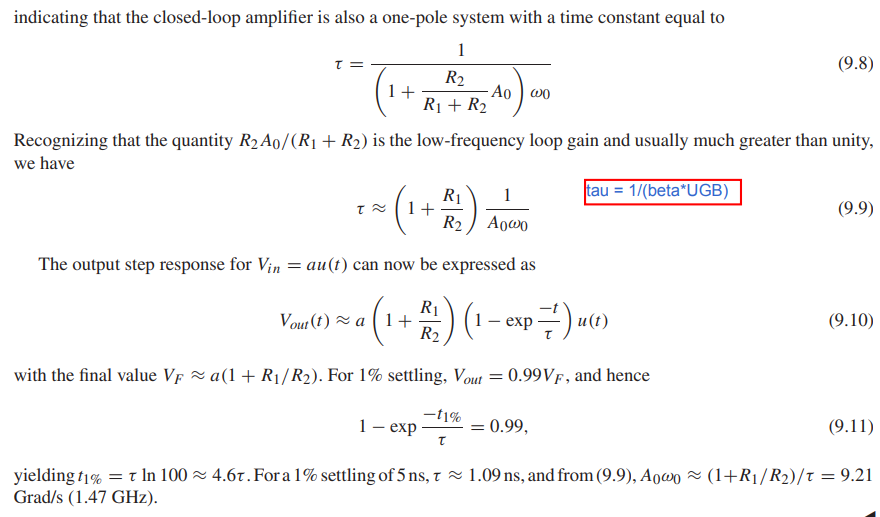image-20240725204527121 \[ \tau \simeq \frac{1}{\beta \omega_\text{ugb}} \]

tau_1pole.drawio

two poles

Rise Time

Katsuhiko Ogata, Modern Control Engineering Fifth Edition

image-20240721180718116

For underdamped second order systems, the 0% to 100% rise time is normally used

For \(\text{PM}=70^o\)

  • \(\omega_2=3\omega_u\), that is \(\omega_n = 1.7\omega_u\).
  • \(\zeta = 0.87\)

Then \[ t_r = \frac{3.1}{\omega_u} \]

Settling Time

Gene F. Franklin, Feedback Control of Dynamic Systems, 8th Edition

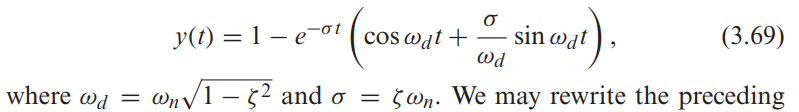
image-20240721181956221

image-20240721182025547

As we know \[ \zeta \omega_n=\frac{1}{2}\sqrt{\frac{\omega_2}{\omega_u}}\cdot \sqrt{\omega_u\omega_2}=\frac{1}{2}\omega_2 \]

Then \[ t_s = \frac{9.2}{\omega_2} \]

For \(\text{PM}=70^o\), \(\omega_2 = 3\omega_u\), that is \[ t_s \simeq \frac{3}{\omega_u} \space\space \text{, for PM}=70^o \]

For \(\text{PM}=45^o\), \(\omega_2 = \omega_u\), that is \[ t_s \simeq \frac{9.2}{\omega_u} \space\space \text{, for PM}=45^o \]

Above equation is valid only for underdamped, \(\zeta=\frac{1}{2}\sqrt{\frac{\omega_2}{\omega_u}}\lt 1\), that is \(\omega_2\lt 4\omega_u\)

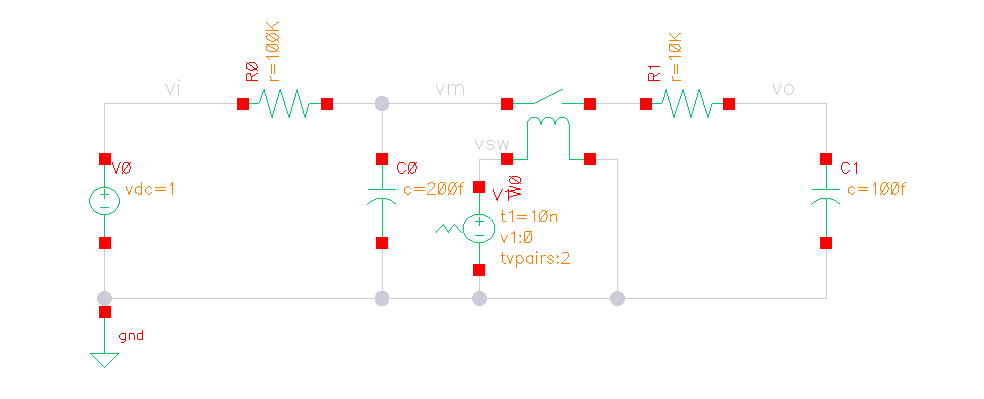
2 Stage RC filter

high frequency pass

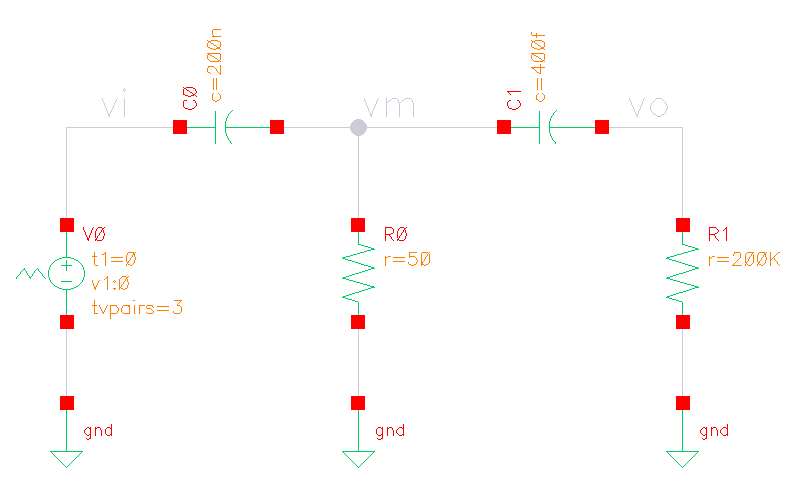
image-20240112002314153

Since \(1/sC_1+R_1 \gg R_0\) \[ \frac{V_m}{V_i}(s) \simeq \frac{R_0}{R_0 + 1/sC_0} = \frac{sR_0C_0}{1+sR_0C_0} \] step response of \(V_m\) \[ V_m(t) = e^{-t/R_0C_0} \] where \(\tau = R_0C_0\)

And \(V_o(s)\) can be expressed as \[\begin{align} \frac{V_o}{V_i}(s) & \simeq \frac{sR_0C_0}{1+sR_0C_0} \cdot \frac{sR_1C_1}{1+sR_1C_1} \\ &= \frac{sR_0C_0R_1C_1}{R_0C_0-R_1C_1}\left(\frac{1}{1+sR_1C_1} - \frac{1}{1+sR_0C_0}\right) \end{align}\]

Then step response of \(Vo\) \[\begin{align} Vo(t) &= \frac{R_0C_0R_1C_1}{R_0C_0-R_1C_1} \left(\frac{1}{R_1C_1}e^{-t/R_1C_1} - \frac{1}{R_0C_0}e^{-t/R_0C_0}\right) \\ &= \frac{1}{R_0C_0-R_1C_1}\left(R_0C_0e^{-t/R_1C_1} - R_1C_1e^{-t/R_0C_0}\right) \\ &\approx \frac{1}{R_0C_0-R_1C_1}\left(R_0C_0e^{-t/R_1C_1} - R_1C_1\right) \end{align}\]

where \(\tau=R_1C_1\)

Partial-fraction Expansion

1
2
3
4
5
6
7
8
9
10
syms C0
syms R0
syms C1
syms R1
syms s

Z0 = 1/s/C1 + R1;
Z1 = R0*Z0/(R0+Z0);
vm = Z1 / (Z1 + 1/s/C0);
vo = R1/Z0 * vm;
1
2
3
4
5
6
7
8
9
10
11
>> partfrac(vm, s)
 
ans =
 
1 - (s*(C1*R0 + C1*R1) + 1)/(C0*C1*R0*R1*s^2 + (C0*R0 + C1*R0 + C1*R1)*s + 1)
 
>> partfrac(vo, s)
 
ans =
 
1 - (s*(C0*R0 + C1*R0 + C1*R1) + 1)/(C0*C1*R0*R1*s^2 + (C0*R0 + C1*R0 + C1*R1)*s + 1)

\[\begin{align} V_m(s) &= 1 - \frac{s(C_1R_0+C_1R_1)+1}{C_0C_1R_0R_1s^2+(C_0R_0+C_1R_0+C_1R_1)s+1} \\ V_o(s) &= 1 - \frac{s(C_0R_0+C_1R_0+C_1R_1)+1}{C_0C_1R_0R_1s^2+(C_0R_0+C_1R_0+C_1R_1)s+1} \end{align}\]

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
C0 = 200e-9;
R0 = 50;
C1 = 400e-15;
R1 = 200e3;

s = tf("s");

Z0 = 1/s/C1 + R1;
Z1 = R0*Z0/(R0+Z0);
vm = Z1 / (Z1 + 1/s/C0);
vo = R1/Z0 * vm;

vm_exp = 1 - (s*(C1*R0 + C1*R1) + 1)/(C0*C1*R0*R1*s^2 + (C0*R0 + C1*R0 + C1*R1)*s + 1);
vo_exp = 1 - (s*(C0*R0 + C1*R0 + C1*R1) + 1)/(C0*C1*R0*R1*s^2 + (C0*R0 + C1*R0 + C1*R1)*s + 1);

figure(1)
subplot(1,2,1)
step(vm, 500e-9, 'k-o');
hold on;
step(vm_exp, 500e-9, 'r-^')
title('vm step response')
grid on;
legend()


subplot(1,2,2)
step(vo, 500e-9, 'k-o');
hold on;
step(vo_exp, 500e-9, 'r-^')
title('vo step response')
grid on;
legend()


% with approximation
figure(2)
vm_exp2 = s*R0*C0/(1+s*R0*C0);
vo_exp2 = s*R0*C0/(1+s*R0*C0) * s*R1*C1/(1+s*R1*C1);

subplot(1,2,1)
step(vm, 500e-9, 'k-o');
hold on;
step(vm_exp2, 500e-9, 'r-^')
title('vm step response')
grid on;
legend()

subplot(1,2,2)
step(vo, 500e-9, 'k-o');
hold on;
step(vo_exp2, 500e-9, 'r-^')
title('vo step response')
grid on;
legend()

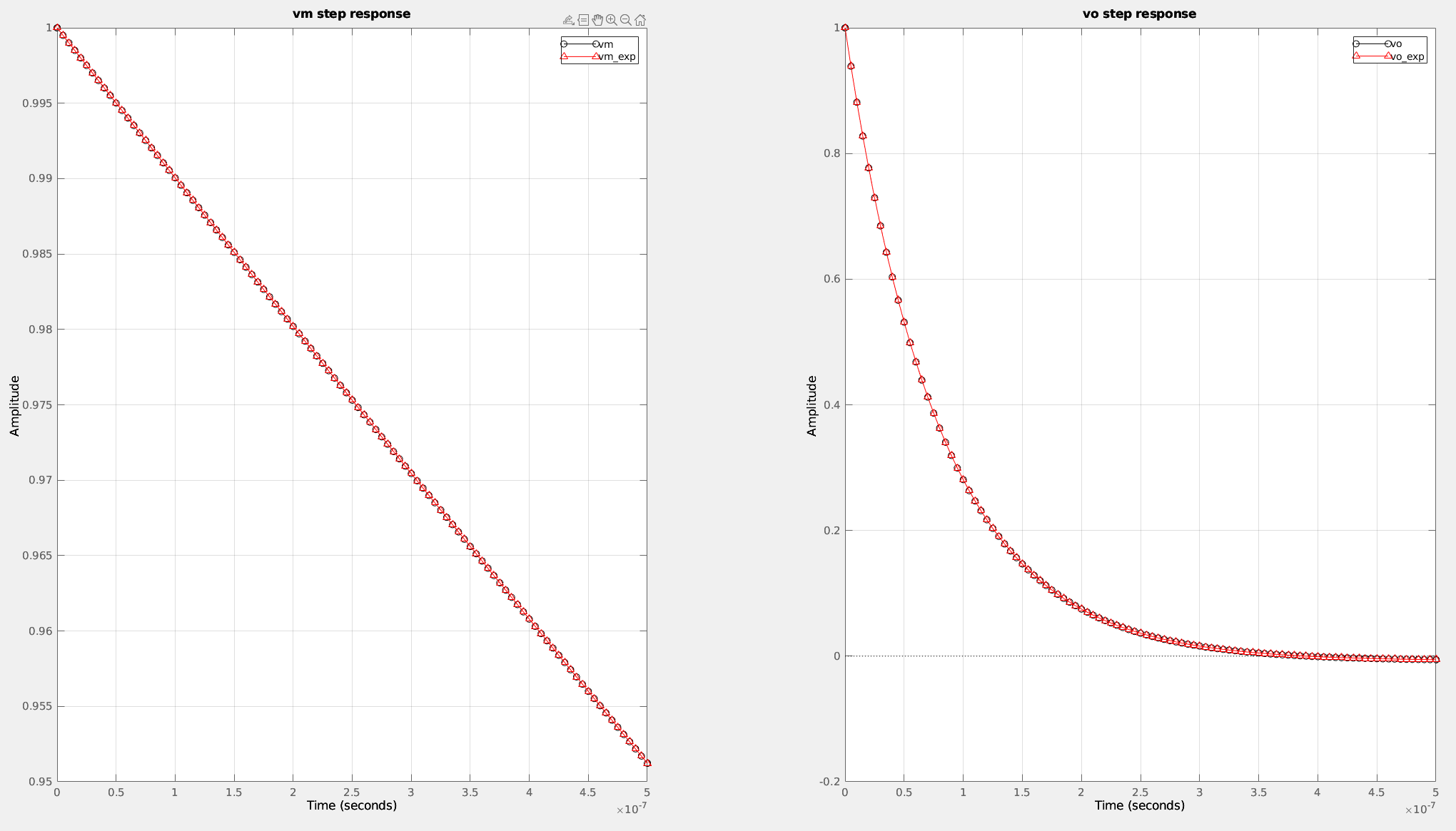
image-20240113181003272

image-20240113181032379

spectre simulation vs matlab

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
C0 = 200e-9;
R0 = 50;
C1 = 400e-15;
R1 = 200e3;

s = tf("s");

Z0 = 1/s/C1 + R1;
Z1 = R0*Z0/(R0+Z0);
vm = Z1 / (Z1 + 1/s/C0);
vo = R1/Z0 * vm;

step(vm, 500e-9);
hold on;
step(vo, 500e-9);

grid on
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
>> vm

vm =
 
          1.024e-44 s^5 + 2.56e-37 s^4 + 1.6e-30 s^3
  -----------------------------------------------------------
  1.024e-44 s^5 + 2.571e-37 s^4 + 1.626e-30 s^3 + 1.6e-25 s^2
 
Continuous-time transfer function.

>> vo

vo =
 
                 8.194e-52 s^6 + 2.048e-44 s^5 + 1.28e-37 s^4
  ---------------------------------------------------------------------------
  8.194e-52 s^6 + 3.081e-44 s^5 + 3.871e-37 s^4 + 1.638e-30 s^3 + 1.6e-25 s^2
 
Continuous-time transfer function.

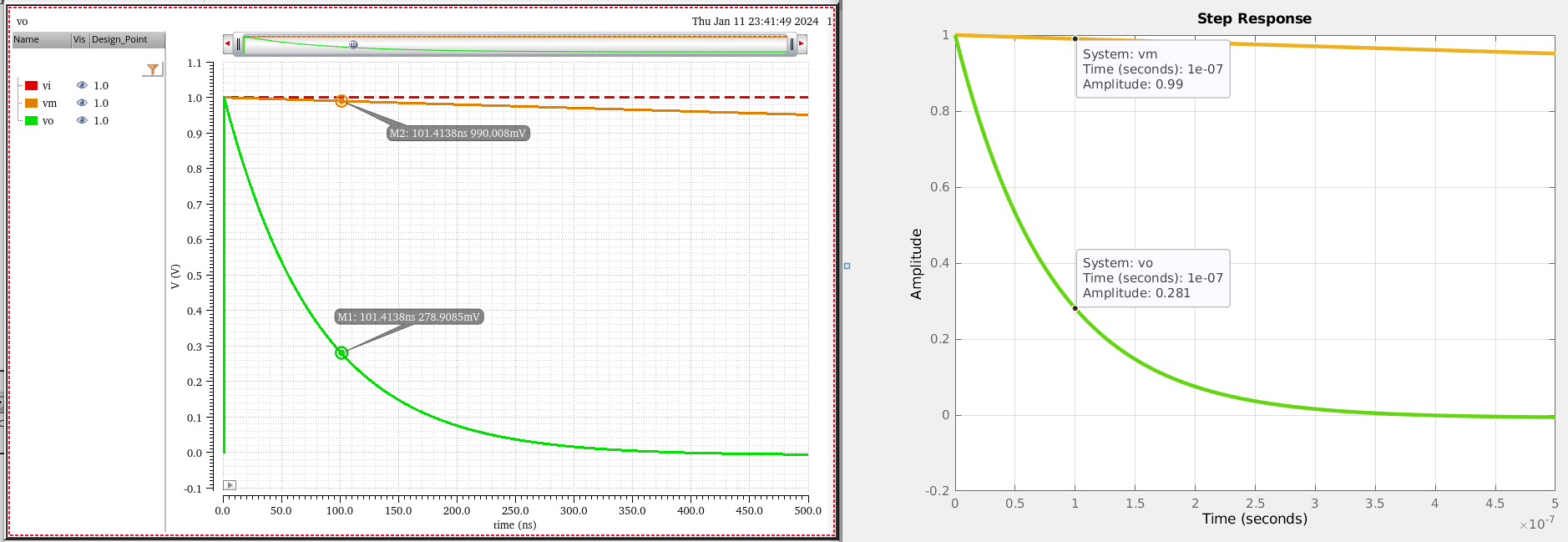
image-20240112002155622

low frequency pass

image-20241218231322792

\[ \left\{ \begin{array}{cl} \frac{V_i - V_m}{R_0} &= C_0\frac{dV_m}{dt} + C_1\frac{dV_o}{dt} \\ \frac{V_m - V_o}{R_1} &= C_1\frac{dV_o}{dt} \\ V_m(t=0) &= 1 \\ V_o(t=0) &= 0 \end{array} \right. \]

Take into initial condition account \[ \frac{dV_m}{dt} \overset{\mathcal{L}}{\longrightarrow} sV_M-V_{m0} \] where \(V_{m0} = 1\)

\[\begin{align} V_O(s) &= \frac{V_I}{s^2R_0C_0R_1C_1+s(R_0C_0+R_1C_1+R_0C_1)+1} + \frac{V_{m0}R_0C_0}{s^2R_0C_0R_1C_1+s(R_0C_0+R_1C_1+R_0C_1)+1} \\ &\approx \frac{V_I}{s^2R_0C_0R_1C_1+sR_0(C_0+C_1)+1} + \frac{V_{m0}R_0C_0}{s^2R_0C_0R_1C_1+sR_0(C_0+C_1)+1} \\ &= \frac{V_I}{R_0(C_0+C_1)}\left(\frac{R_0(C_0+C_1)}{sR_0(C_0+C_1)+1} - \frac{R_1\frac{C_0C_1}{C_0+C_1}}{sR_1\frac{C_0C_1}{C_0+C_1}+1}\right) \\ &+ \frac{C_0}{C_0+C_1}\left(\frac{R_0(C_0+C_1)}{sR_0(C_0+C_1)+1} - \frac{R_1\frac{C_0C_1}{C_0+C_1}}{sR_1\frac{C_0C_1}{C_0+C_1}+1}\right) \end{align}\]

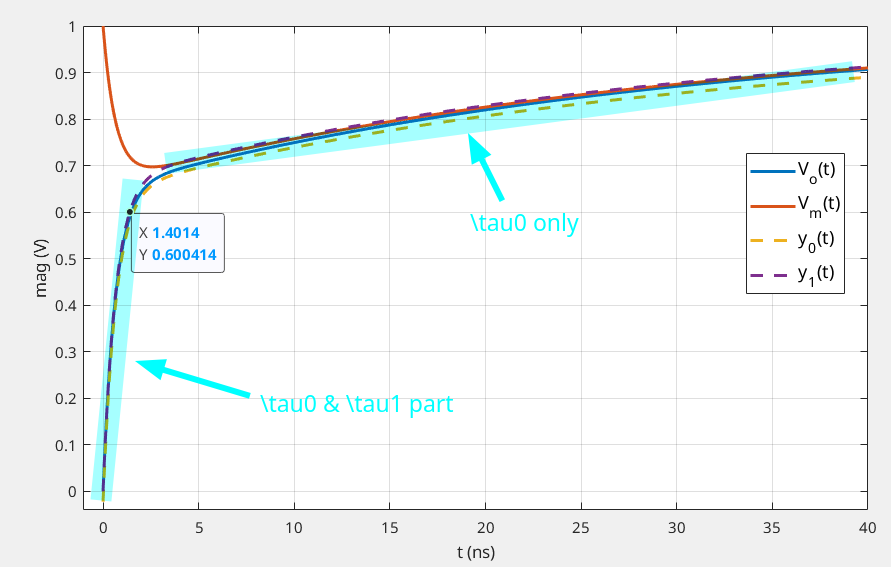
with \(V_I = \frac{1}{s}\), using inverse Laplace transform \[\begin{align} V_o(t) &= 1 - \frac{C_1}{C_0+C_1}e^{-t/\tau_0}-\frac{C_0}{C_0+C_1}e^{-t/\tau_1} - \frac{R_1C_0C_1}{R_0(C_0+C_1)^2} \tag{Eq.0} \\ &= 1 - \frac{C_1}{C_0+C_1}e^{-t/\tau_0}-\frac{C_0}{C_0+C_1}e^{-t/\tau_1} \tag{Eq.1} \end{align}\]

where

\[\begin{align} \tau_0 &= R_0(C_0+C_1) \\ \tau_1 &= R_1C_1\cdot \frac{C_0}{C_0+C_1} \end{align}\]

and \(\tau_0 \gg \tau_1\)

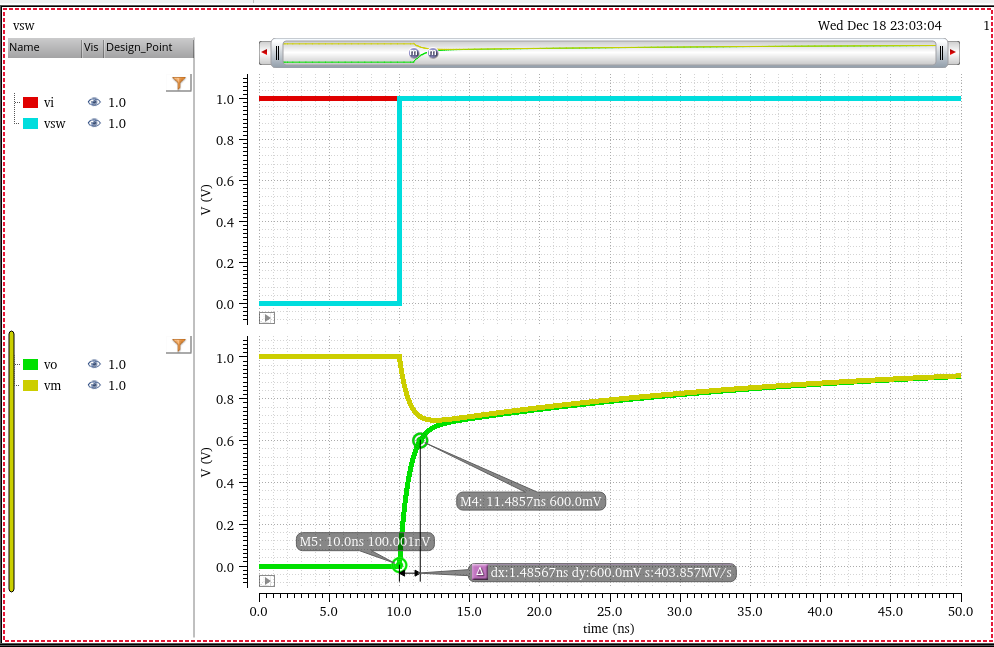
image-20241218230713148

image-20241218231130166

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
R0 = 100e3;
C0 = 200e-15;
R1 = 10e3;
C1 = 100e-15;

s = tf('s');

VI = 1/s;
Vm0 = 1;

deno = (s^2*R0*C0*R1*C1 + s*(R0*C0 + R1*C1 + R0*C1) + 1);
VO = VI/deno + (Vm0*R0*C0)/deno;
VM = (1+s*R1*C1)*VO;

t = linspace(0,40,1e3);  % ns
[vot, ~] = impulse(VO, t*1e-9);
[vmt, ~] = impulse(VM, t*1e-9);

tau0 = R0*(C0+C1)*1e9; %ns
tau1 = R1*C0*C1/(C0+C1)*1e9; %ns
y0 = 1 - C1/(C0+C1)*exp(-t/tau0) - C0/(C0+C1)*exp(-t/tau1) - R1*C0*C1/R0/(C0+C1)^2;  % Eq.0
y1 = 1 - C1/(C0+C1)*exp(-t/tau0) - C0/(C0+C1)*exp(-t/tau1);  % Eq.1

plot(t, vot, t, vmt,LineWidth=2);
hold on
plot(t,y0, t,y1, LineWidth=2, LineStyle="--" );
xlim([-10, 40])
legend('V_o(t)', 'V_m(t)','y_0(t)', 'y_1(t)', fontsize=12)
xlabel('t (ns)')
ylabel('mag (V)')
grid on;

reference

Gene F. Franklin, J. David Powell, and Abbas Emami-Naeini. 2018. Feedback Control of Dynamic Systems (8th Edition) (8th. ed.). Pearson.

Katsuhiko Ogata, Modern Control Engineering, 5th edition