SAR ADC

Posted on Edited on In

Reference Ripple

C-H Chan (U. of Macau) "Extreme SAR ADCs - Exploring New Frontiers" Online Course (2024) : Reference Buffer in SAR ADC [https://youtu.be/vj98B7AaC9E?si=hMt0PM07CdkHN5Qn]

C. Li, C. -H. Chan, Y. Zhu and R. P. Martins, "Analysis of Reference Error in High-Speed SAR ADCs With Capacitive DAC," in IEEE Transactions on Circuits and Systems I: Regular Papers, vol. 66, no. 1, pp. 82-93, Jan. 2019 [https://ime.um.edu.mo/wp-content/uploads/magazines/961546494e705f6fd16b9f785a121030.pdf]

J. Zhong, Y. Zhu, S. -W. Sin, S. -P. U and R. P. Martins, "Thermal and Reference Noise Analysis of Time-Interleaving SAR and Partial-Interleaving Pipelined-SAR ADCs," in IEEE Transactions on Circuits and Systems I: Regular Papers, vol. 62, no. 9, pp. 2196-2206, Sept. 2015 [https://sci-hub.st/10.1109/TCSI.2015.2452331]

C. -H. Chan et al., "60-dB SNDR 100-MS/s SAR ADCs With Threshold Reconfigurable Reference Error Calibration," in IEEE Journal of Solid-State Circuits, vol. 52, no. 10, pp. 2576-2588, Oct. 2017 [https://ime.um.edu.mo/wp-content/uploads/magazines/407e580ac0218605bcf9b9bbd0ea1109.pdf]

TODO 📅

sample-by-sample

3rd harmonic

sample2sample-gain-distortion.drawio

bit-by-bit

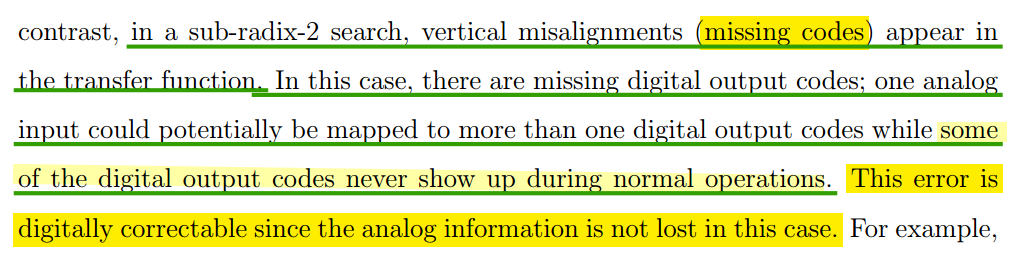
The amplitude of the reference ripple is code-dependent as it is correlated with switching energy in each bit cycling

quantization error & quantization noise

image-20250910210909363

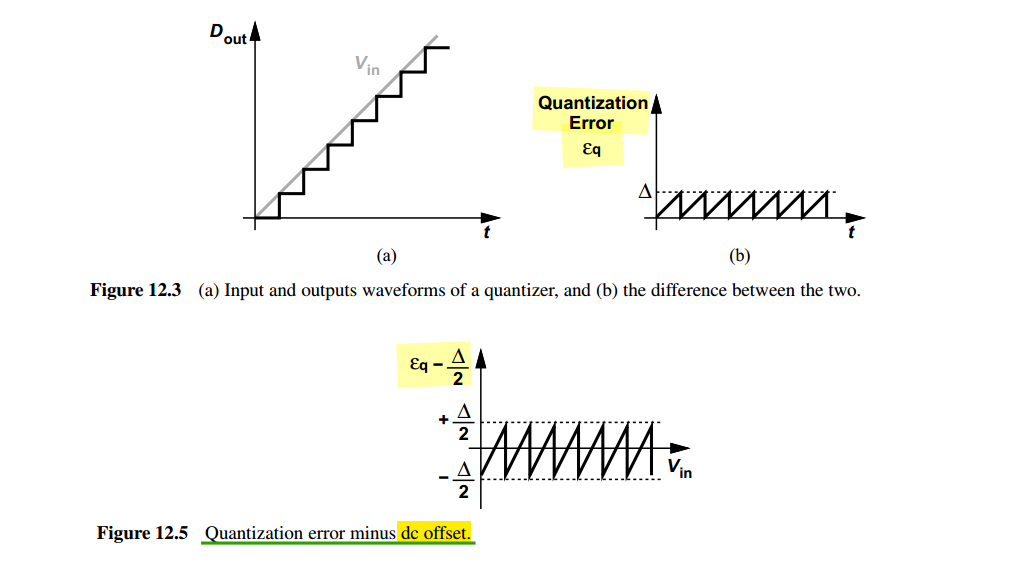
image-20250910211207655

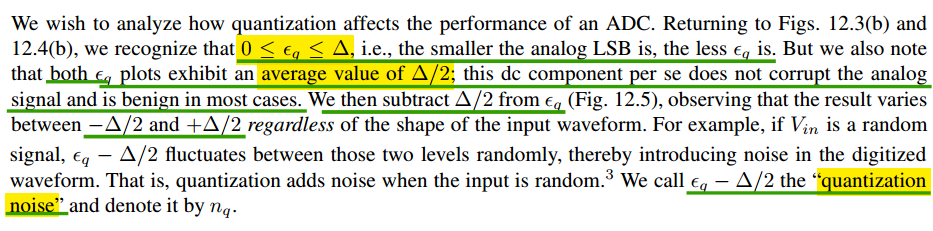
image-20250910211034914

Notice \(e_q\in (0, \Delta)\) and its average is \(\Delta/2\). To calculate SNDR, DC component shall be excluded

Don't confuse resolution \(\Delta\) with Bounded Quantization Noise \(-\Delta/2 \sim \Delta/2\)

image-20250909233010702

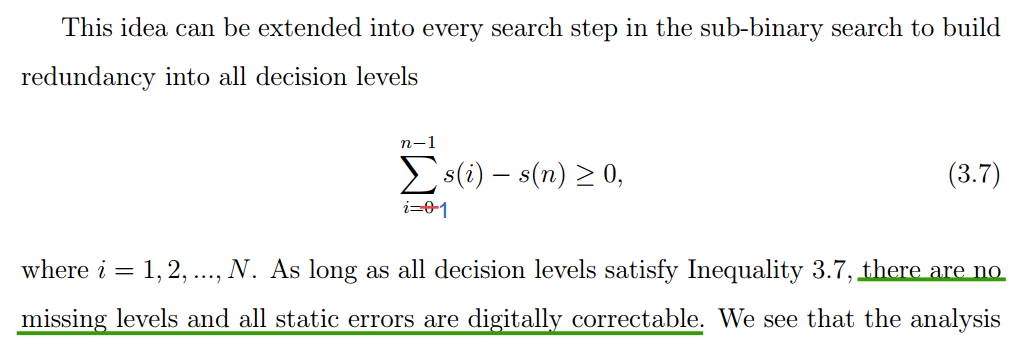
Redundancy

decision level

final digital output for an \(N\)-bit \(M\)-step ADC can be calculated \[ D_{out} = s(M) + \sum_{i=1}^{M-1}(2\cdot b[i] - 1)\times s(i) + (b[0] -1)\cdot s(1) \]

i M M-1 M-2 ... 2 1 0
b[i] b[M-1] b[M-2] ... b[2] b[1] b[0]
s[i] s(M) s(M-1) s(M-2) ... s(2) s(1)

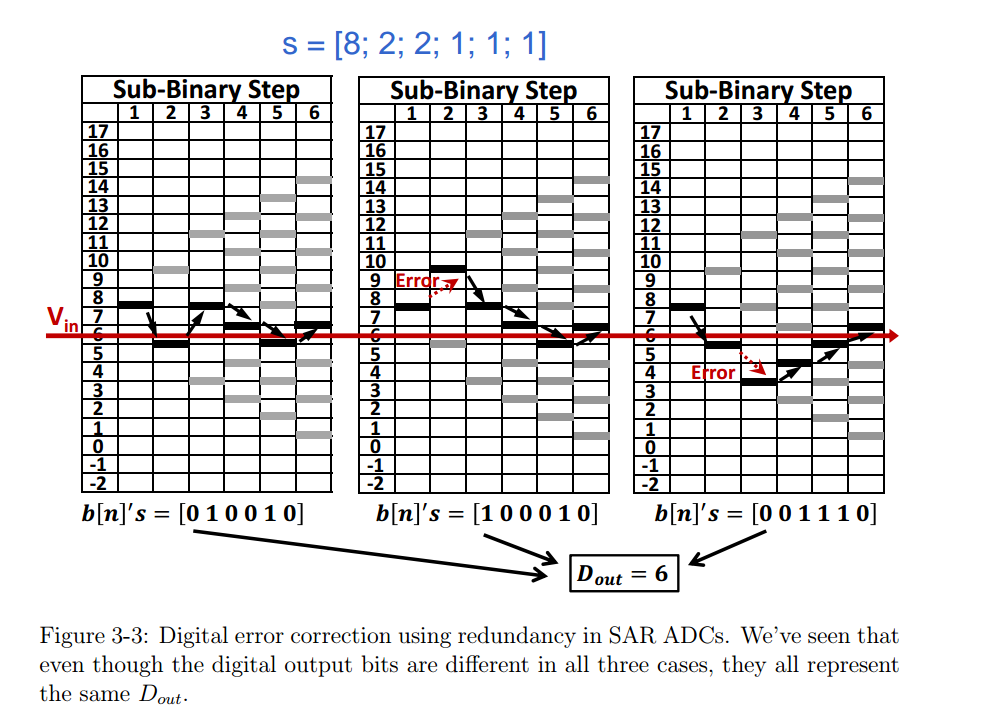
image-20250909211030234

track the decision level

For \(N\)-bit binary weighted algorithm,\(N=M\) and \(s(i)=2^{i-1}\), where \(i\in \{N, N-1,...,2,1 \}\)

\[\begin{align} D_{out} &= s(M) + \sum_{i=1}^{M-1}(2\cdot b[i] - 1)\times s(i) + (b[0] -1) \\ &= 2^{N-1} + \sum_{i=1}^{N-1}2^i\cdot b[i] - \sum_{i=0}^{N-2}2^{i} + (b[0] -1) \\ &= \sum_{i=0}^{N-1} b[i] \cdot 2^i \end{align}\]

Error Tolerance Window

\[ \varepsilon_t(n) = \sum_{i=1}^{n-2} s(i) - s(n-1) \]

where \(n\in [1, N]\), and \(N\)-bit SAR

etw.drawio

For the \(n\)th output bit, once a decision is made, the next decision level will either move up or down by the step size of \(s(n − 1)\)

If this decision is erroneous, then the sum of the follow-on step sizes, \(s(n − 2)\), \(s(n − 3)\), ..., \(s(1)\), must be large enough and exceed the value of the current step size to counteract this mistake

The exceeded amount is the tolerance window for that decision level

image-20250909222730303

image-20250909222310476

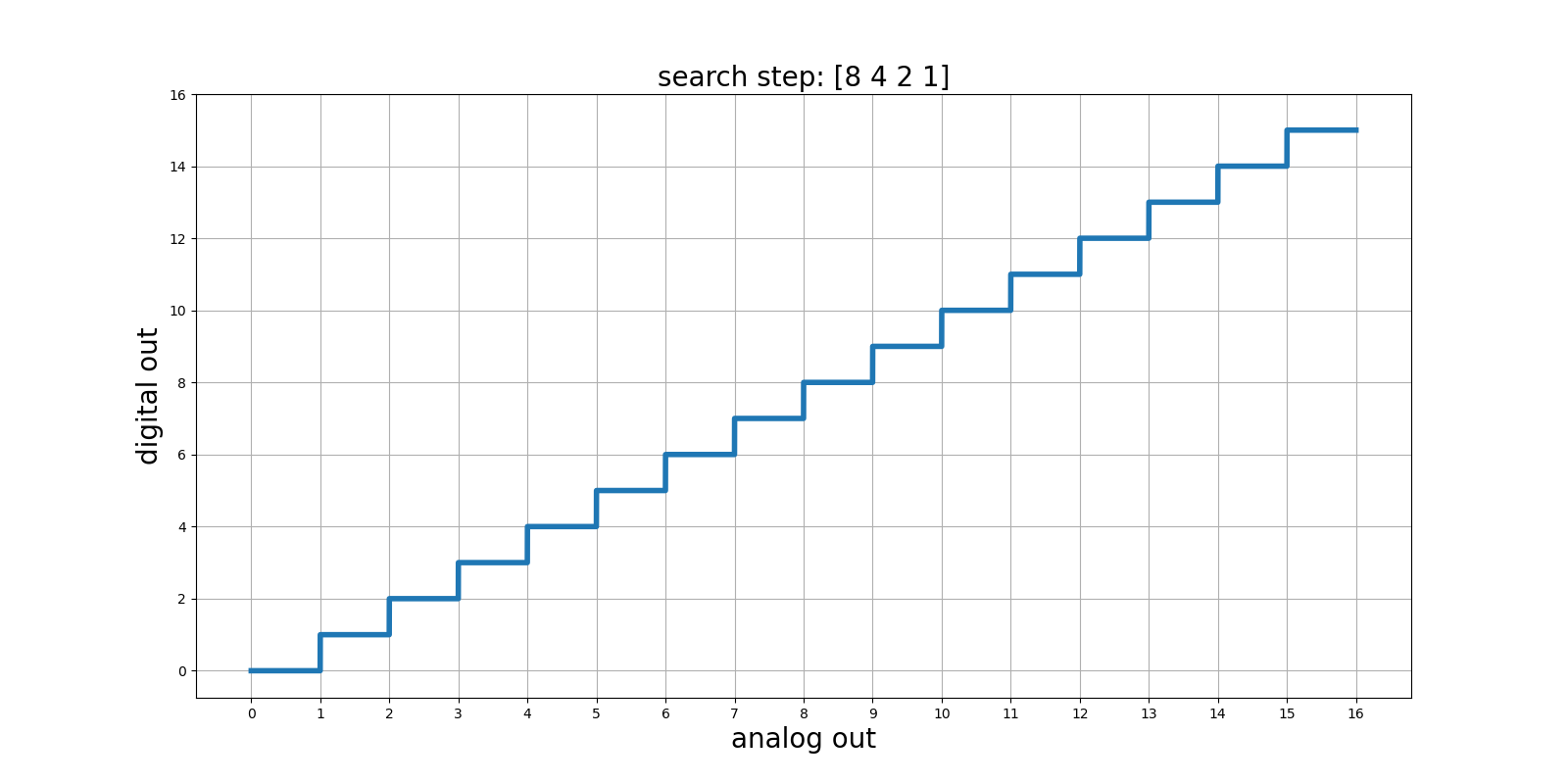
image-20250909231804142

image-20250909222622340

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
import numpy as np
import matplotlib.pyplot as plt


def sar(xi, ss):
    M = ss.size
    th = ss[0]
    oob = []
    for i in range(M):
        ocur = 1 if xi >= th else 0
        oob.append(ocur)
        if i + 1 < M:
            th += (2 * ocur - 1) * ss[i + 1]
        else:
            break

    binstr = ''.join([str(s) for s in oob])
    decval = int(binstr, 2)
    return binstr, decval


def sar_plot(ss, Npts=10000):
    ss = np.asarray(ss)
    ssum = np.sum(ss)
    xilist = np.linspace(0, ssum + 1, Npts)
    outlist = []
    for i in range(Npts):
        _, decval = sar(xilist[i], ss)
        outlist.append(decval)
    outmax = np.max(outlist)
    plt.figure(figsize=(16,8))
    plt.plot(xilist, outlist, '-', linewidth=4)
    plt.xticks(range(0, ssum + 2))
    plt.yticks(range(0, outmax + 2, 2))
    plt.title('search step: {}'.format(ss), fontsize=20)
    plt.xlabel('analog out', fontsize=20); plt.ylabel('digital out', fontsize=20)

    plt.grid(True)

ss = [8, 4, 2, 1]
sar_plot(ss)

ss = [8, 2, 2, 2, 1]
sar_plot(ss)

plt.show()

ENOB vs. fixed radix

When the ADC is designed with a fixed radix, \(\alpha\) and the required number of conversion steps, \(M\)

the sum of all the step sizes \(s_{tot}\) \[ s_{tot} = \sum_{k=0}^{M-1} s_0 \alpha^k = s_0\frac{\alpha^M-1}{\alpha-1} \]

where \(s(i)\) is step size and \(i \in [0, 1, 2, M-1]\)

The effective number of bits, \(N\), can be calculated \[ N \leq \log 2\left(\frac{s_{tot} + s_0}{s_0}\right) = \frac{\alpha^M+\alpha-2}{\alpha-1} \]

Speed Benefit

TODO 📅

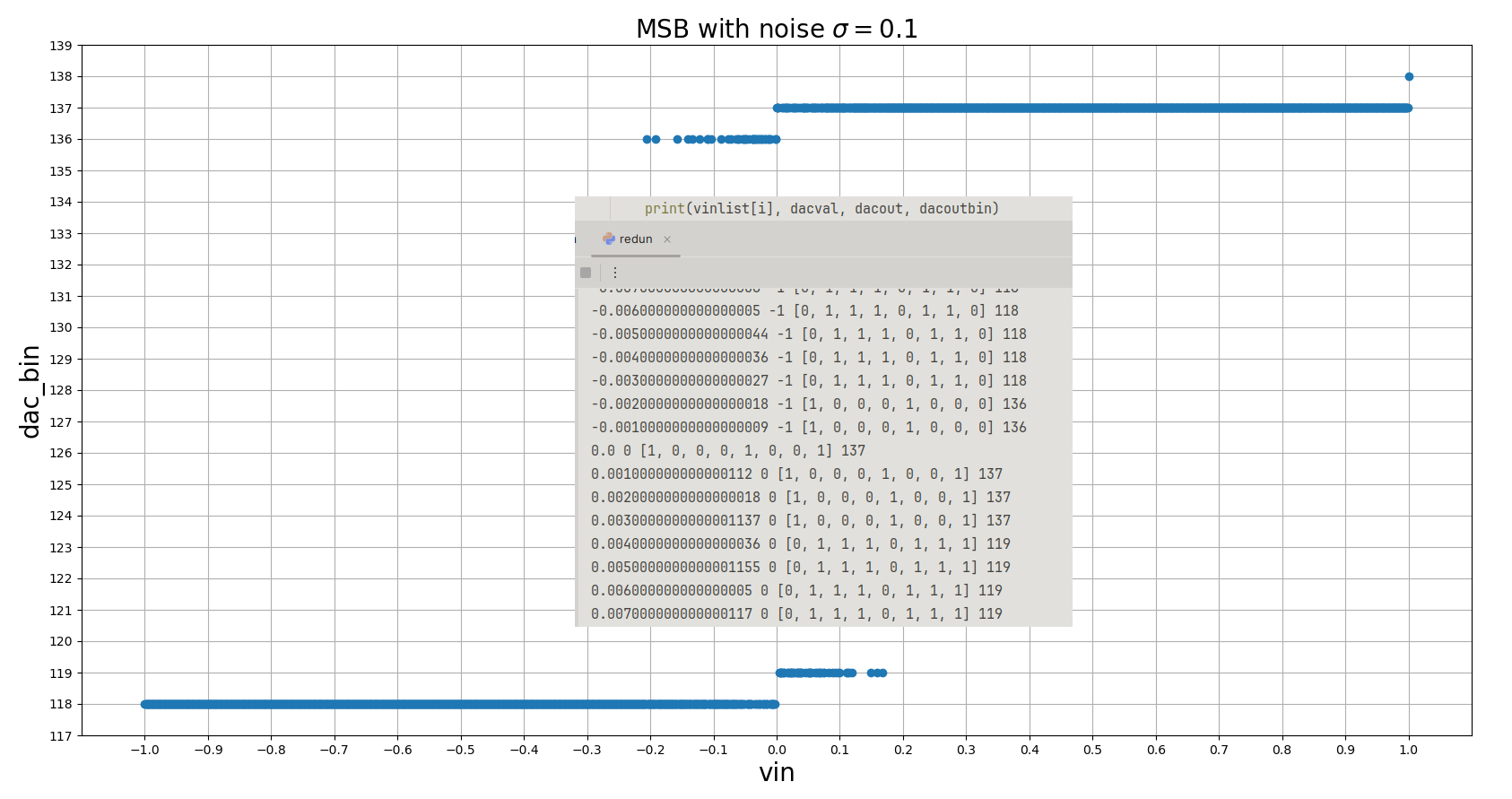
CDAC

The charge redistribution capacitor network is used to sample the input signal and serves as a digital-to-analog converter (DAC) for creating and subtracting reference voltages

sampling charge \[ Q = V_{in} C_{tot} \] conversion charge \[ Q = -C_{tot}V_c + V_{ref}C_\Delta \] That is \[ V_c = \frac{C_\Delta}{C_{tot}}V_{ref} - V_{in} \]

CDAC is actually working as a capacitive divider during conversion phase, the charge of internal node retain (charge conservation law)

assuming \(\Delta V_i\) is applied to series capacitor \(C_1\) and \(C_2\)

cap_divider.drawio \[ (\Delta V_i - \Delta V_x) C_1 = \Delta V_x \cdot C_2 \] Then \[ \Delta V_x = \frac{C_1}{C_1+C_2}\Delta V_i \]

\(V_x= V_{x,0} + \Delta V_x\)

CDAC settling accuracy

cdac-tau.drawio \[\begin{align} V_x(s) &= \frac{C_1+C_2}{RC_1C_2}\cdot \frac{1}{s+\frac{C_1+C_2}{RC_1C_2}}\cdot V_i(s) \\ &= \frac{1}{\tau}\cdot \frac{1}{s+\frac{1}{\tau}}\cdot \frac{1}{s}\\ &= \frac{1}{\tau}\cdot \tau(\frac{1}{s} - \frac{1}{s+\frac{1}{\tau}})=\frac{1}{s} - \frac{1}{s+\frac{1}{\tau}} \end{align}\]

inverse Laplace Transform is \(V_x(t) = 1 - e^{-t/\tau}\)

\[\begin{align} V_y(s) &= V_x\frac{C_1}{C_1+C_2} \\ &= \frac{C_1}{C_1+C_2} \left(\frac{1}{s} - \frac{1}{s+\frac{1}{\tau}}\right)\\ \end{align}\]

inverse Laplace Transform is \(V_y(t) = \frac{C_1}{C_1+C_2}\left(1 - e^{-t/\tau}\right)\)

\(V_x(t)\) and \(V_y(t)\) prove that the settling time is same

\(\tau = R\frac{C_1C_2}{C_1+C_2}\), which means usually worst for MSB capacitor (largest)

both \(\tau\) and \(\Delta V\) are the maximum

A popular way to improve the settling behavior, again, is to employ unit-element DACs that statistically reduce the switching activities, which, unfortunately, exhibits unnecessary complications to the power, area and speed tradeoffs of the design

CDAC Energy Consumption

\[ E_{Vref} = \int P(t)dt = \int V_{ref} I(t) dt = V_{ref}\int I(t)dt = V_{ref}\cdot \Delta Q \]

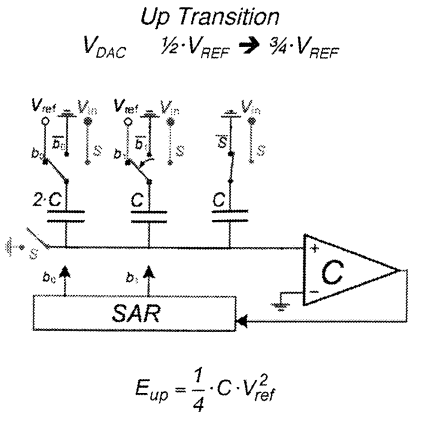
image-20240922093524720

Given \(V_{c,0}=\frac{1}{2}V_{ref}-V_{in}\) and \(V_{c,1}=\frac{3}{4}V_{ref}-V_{in}\) \[\begin{align} Q_{b0,0} &= \left(V_{ref} - V_{c,0} \right)\cdot 2C = \left(\frac{1}{2}V_{ref}+V_{in} \right)\cdot 2C \\ Q_{b1,0} &= (0 - V_{c,0})\cdot C = \left(-\frac{1}{2}V_{ref}+V_{in} \right)\cdot C \\ Q_{b0,1} &= \left(V_{ref} - V_{c,1} \right)\cdot 2C = \left(\frac{1}{4}V_{ref}+V_{in} \right)\cdot 2C \\ Q_{b1,1} &= \left(V_{ref} - V_{c,1} \right)\cdot C = \left(\frac{1}{4}V_{ref}+V_{in} \right)\cdot C \end{align}\]

Therefore \[ E_{Vref} = V_{ref}\cdot (Q_{b0,1}+Q_{b1,1} - Q_{b0,0}-Q_{b1,0}) = \frac{1}{4}C V_{ref}^2 \]

CDAC total energy change \[\begin{align} \Delta E_{tot} &= \frac{1}{2}\cdot 2C \cdot (U_{2c,1}^2 - U_{2c,0}^2) + \frac{1}{2}\cdot C \cdot (U_{c,1}^2 - U_{c,0}^2) + \frac{1}{2}\cdot C \cdot (U_{c1,1}^2 - U_{c1,0}^2) \\ &= \left(-\frac{3}{16}V_{ref}^2 - \frac{1}{2}V_{ref}V_{in} - \frac{3}{32}V_{ref}^2+\frac{3}{4}V_{ref}V_{vin} + \frac{5}{32}V_{ref}^2-\frac{1}{4}V_{ref}V_{in}\right)C \\ &= -\frac{1}{8}CV_{ref}^2 \end{align}\]

alternative method

CapEnergy.drawio \[ \Delta E_{tot} = \frac{1}{2}\cdot\frac{3}{4}C\cdot V_{ref}^2 - \frac{1}{2}\cdot C\cdot V_{ref}^2 = -\frac{1}{8}CV_{ref}^2 \]

The total energy decreases by \(-\frac{1}{8}CV_{ref}^2\), though \(V_{ref}\) provides \(\frac{1}{4}C V_{ref}^2\)

The charge redistribution change the CDAC energy

cap_redis_energy.drawio

\[ E_{c,0} = \frac{1}{2}CV^2 \] After charge redistribution \[ E_{c,1} = \frac{1}{2}\cdot 2C\cdot \left(\frac{1}{2}V\right)^2 = \frac{1}{4}CV^2 \]

That make sense, charge redistribution consume energy

Comparator

Comparator input cap effect

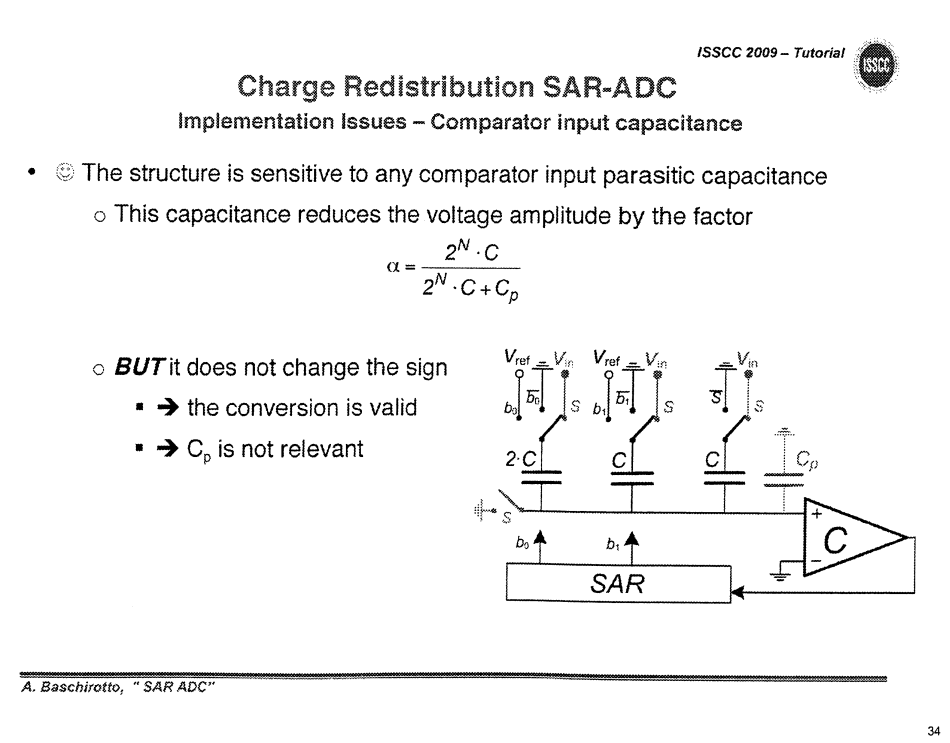image-20240907194621524 \[ -V_{in}\cdot 2^N C = V_c (2^N C + C_p) \] Then \(V_c = -\frac{2^N C}{2^N C + C_p}V_{in}\), i.e. this capacitance reduce the voltage amplitude by the factor

During conversion \[\begin{align} V_c &= -\frac{2^N C}{2^N C + C_p}V_{in} +V_{ref}\sum_{n=0}^{N-1} \frac{b_n\cdot2^n C}{2^N C + C_p} \\ &= \frac{2^N C}{2^N C + C_p}\left(-V_{in} + V_{ref}\sum_{n=0}^{N-1}\frac{b_n }{2^{N-n}} \right) \end{align}\]

That is, it does not change the sign

Comparator offset effect

image-20240825204030645

Synchronous SAR ADC

It also divides a full conversion into several comparison stages in a way similar to the pipeline ADC, except the algorithm is executed sequentially rather than in parallel as in the pipeline case.

However, the sequential operation of the SA algorithm has traditionally been a limitation in achieving high-speed operation

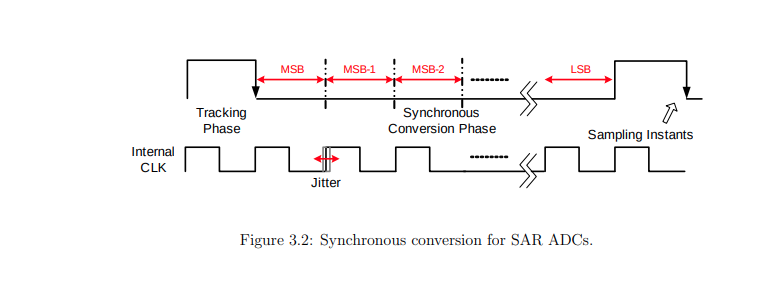
image-20241021214958488

  • a clock running at least \((N + 1) \cdot F_s\) is required for an \(N\)-bit converter with conversion rate of \(F_s\)
  • every clock cycle has to tolerate the worst case comparison time
  • every clock cycle requires margin for the clock jitter

The power and speed limitations of a synchronous SA design comes largely from the high-speed internal clock

Split Arrary CDAC

Split capacitor, double-array cap

attenuation capacitance \(C_a\)

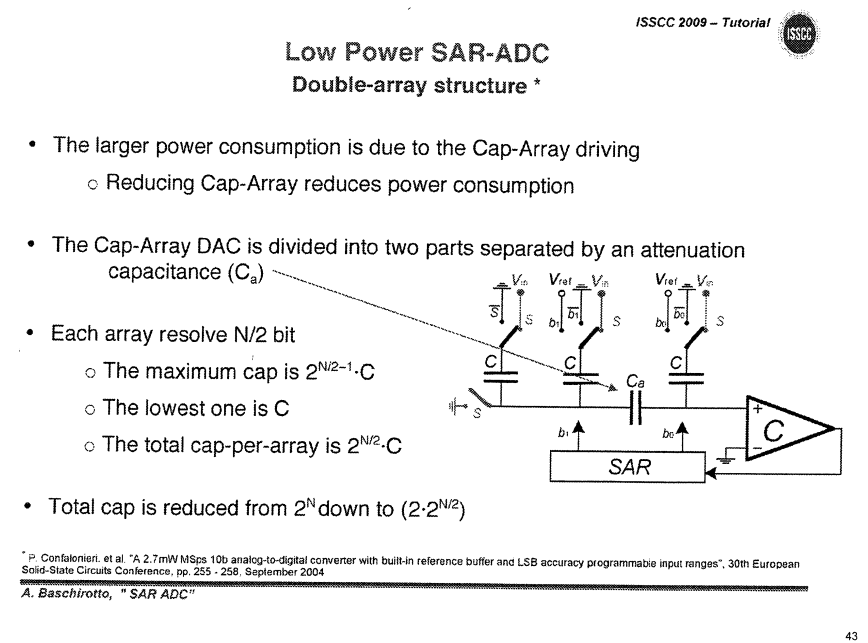
image-20240917192957721

image-20240918213856504

splitArray.drawio

\[\begin{align} \Delta V_{dac} &= \frac{1}{2}b_3+\frac{1}{4}b_2+\frac{1}{4}\left(\frac{1}{2}b_1+\frac{1}{4}b_0 \right) \\ &= \frac{1}{2}b_3+\frac{1}{4}b_2 + \frac{1}{8}b_1+\frac{1}{16}b_0 \end{align}\]

Asynchronous SAR ADC

The comparator itself trigger the next bit-conversion cycle as soon as the present bit decision has been taken

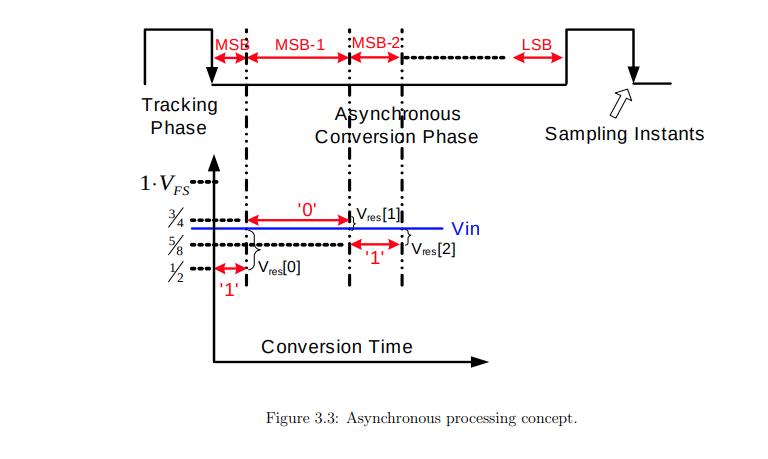
image-20241021214922564

image-20250102225355547

The maximum resolving time reduction between synchronous and asynchronous case is two fold

comparator metastable state

when the input is sufficiently small. The time needed for the comparator outputs to fully resolve may take arbitrarily long

In this case, the ready signal generator should still set the flag and the decision result is simply taken from the previous value stored in the SR latch

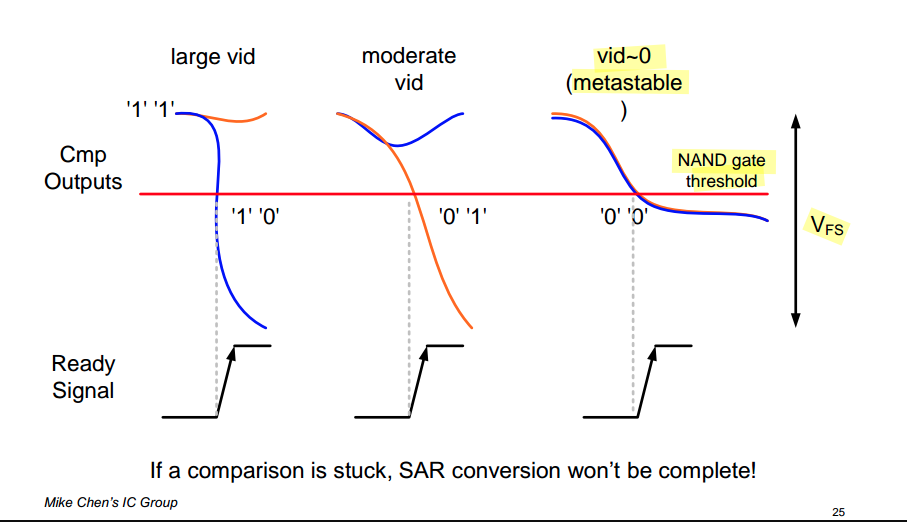
image-20250701231051158

both outputs (\(Q_p\) and \(Q_n\)) will drop together, NAND is inverter actually

The transition point of this NAND gate is skewed to eliminate metastability issues arising when the input differential voltage level is small (comparator)

reference

Andrea Baschirotto, "T6: SAR ADCs" ISSCC2009

Pieter Harpe, ISSCC 2016 Tutorial: "Basics of SAR ADCs Circuits & Architectures"

Mike Shuo-Wei Chen and R. W. Brodersen, "A 6-bit 600-MS/s 5.3-mW Asynchronous ADC in 0.13-Ξm CMOS," in IEEE Journal of Solid-State Circuits, vol. 41, no. 12, pp. 2669-2680, Dec. 2006 [pdf, slides]

—. "Power Efficient System and A/D Converter Design for Ultra-Wideband Radio" [http://www2.eecs.berkeley.edu/Pubs/TechRpts/2006/EECS-2006-71.pdf]

—. "Asynchronous SAR ADC: Past, Present and Beyond" [https://viterbi-web.usc.edu/~swchen/index_files/async_sar_tutorial_chen_final.pdf]

C. -C. Liu, S. -J. Chang, G. -Y. Huang and Y. -Z. Lin, "A 10-bit 50-MS/s SAR ADC With a Monotonic Capacitor Switching Procedure," in IEEE Journal of Solid-State Circuits, vol. 45, no. 4, pp. 731-740, April 2010 [https://sci-hub.se/10.1109/JSSC.2010.2042254]

L. Jie et al., "An Overview of Noise-Shaping SAR ADC: From Fundamentals to the Frontier," in IEEE Open Journal of the Solid-State Circuits Society, vol. 1, pp. 149-161, 2021 [pdf]

W. Liu, P. Huang and Y. Chiu, "A 12-bit, 45-MS/s, 3-mW Redundant Successive-Approximation-Register Analog-to-Digital Converter With Digital Calibration," in IEEE Journal of Solid-State Circuits, vol. 46, no. 11, pp. 2661-2672, Nov. 2011 [https://sci-hub.st/10.1109/JSSC.2011.2163556]