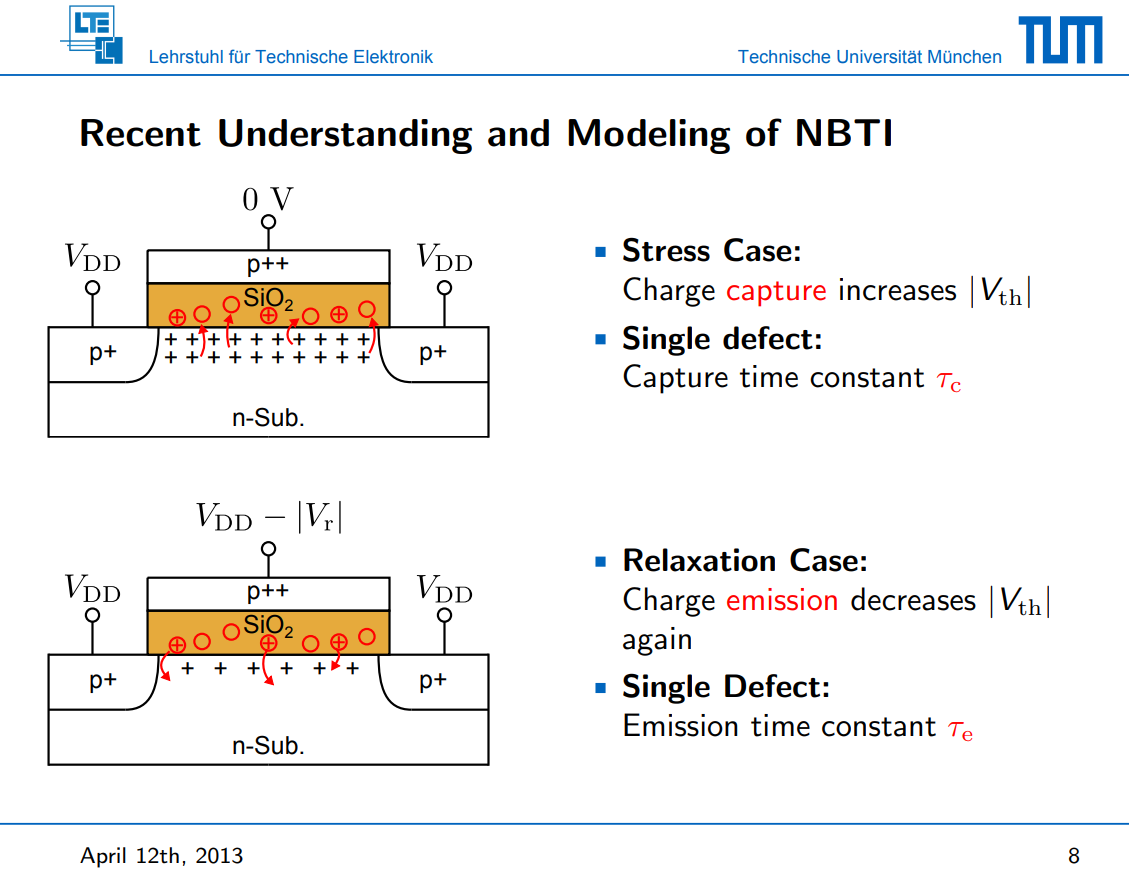
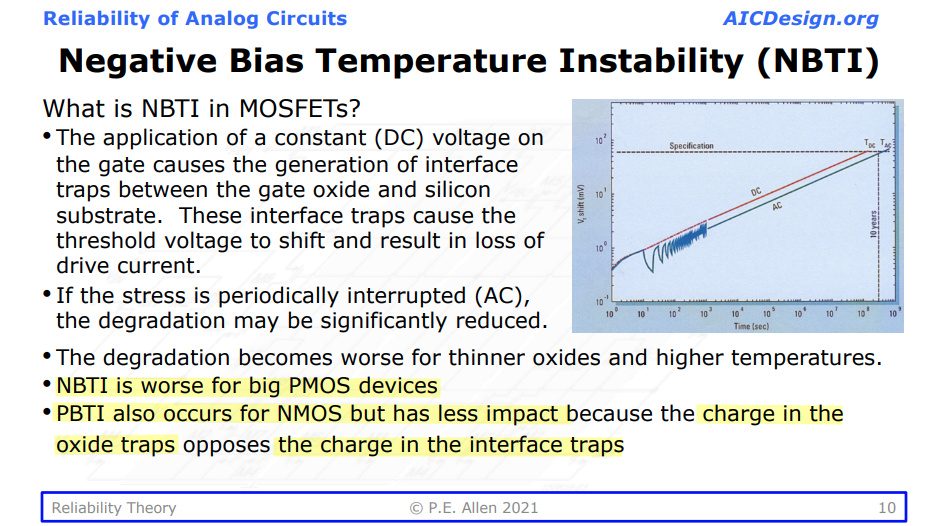
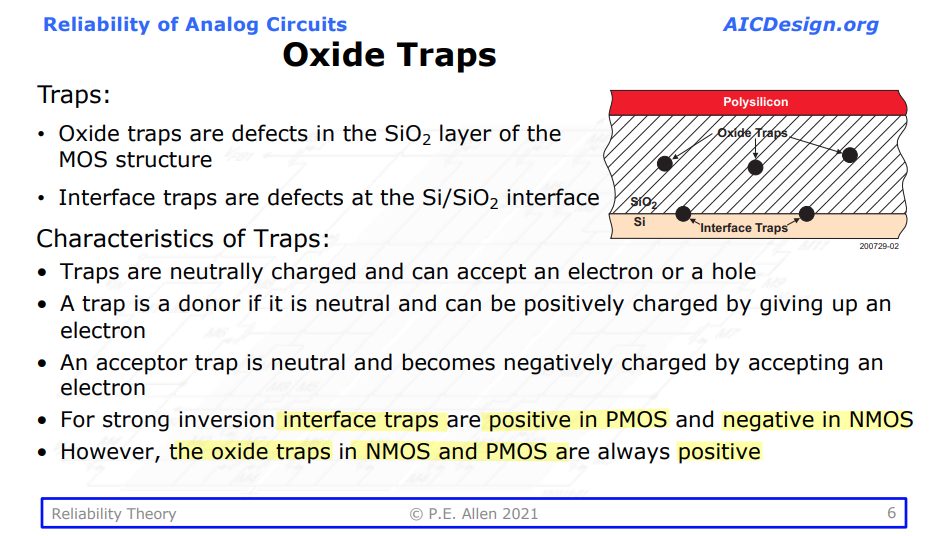
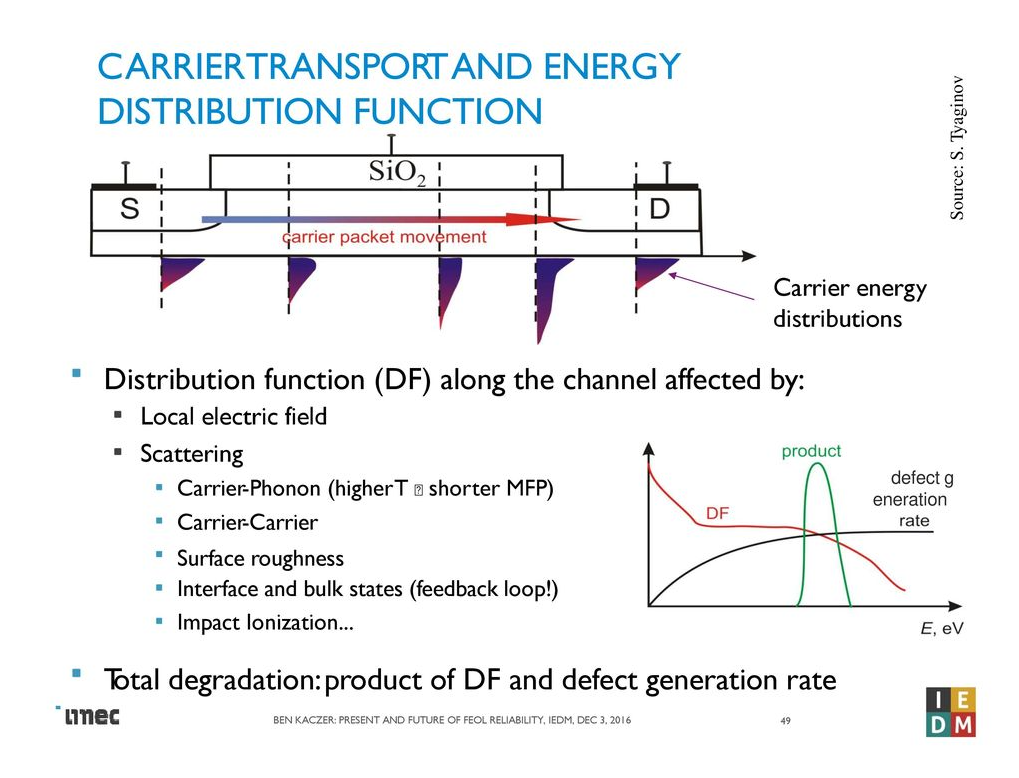
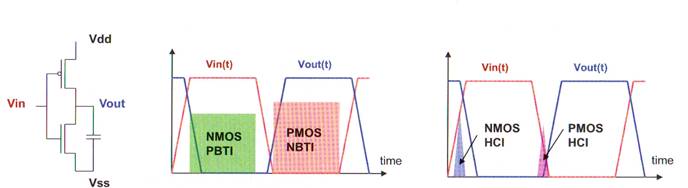
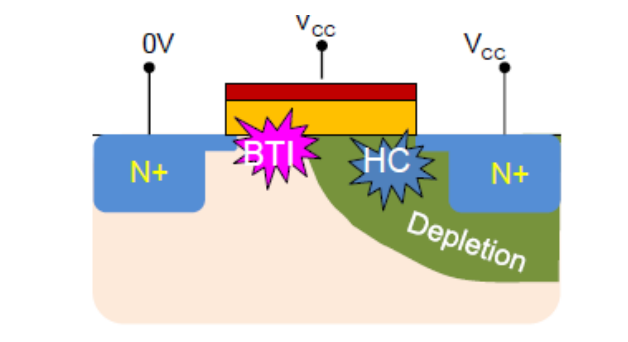
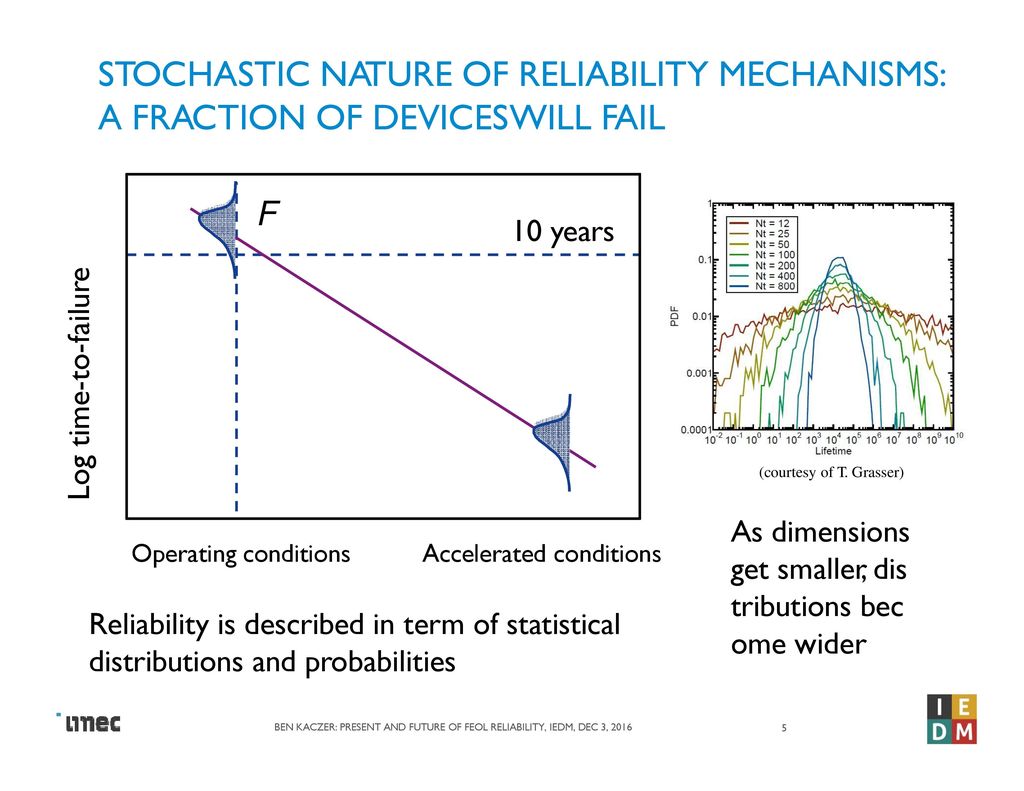
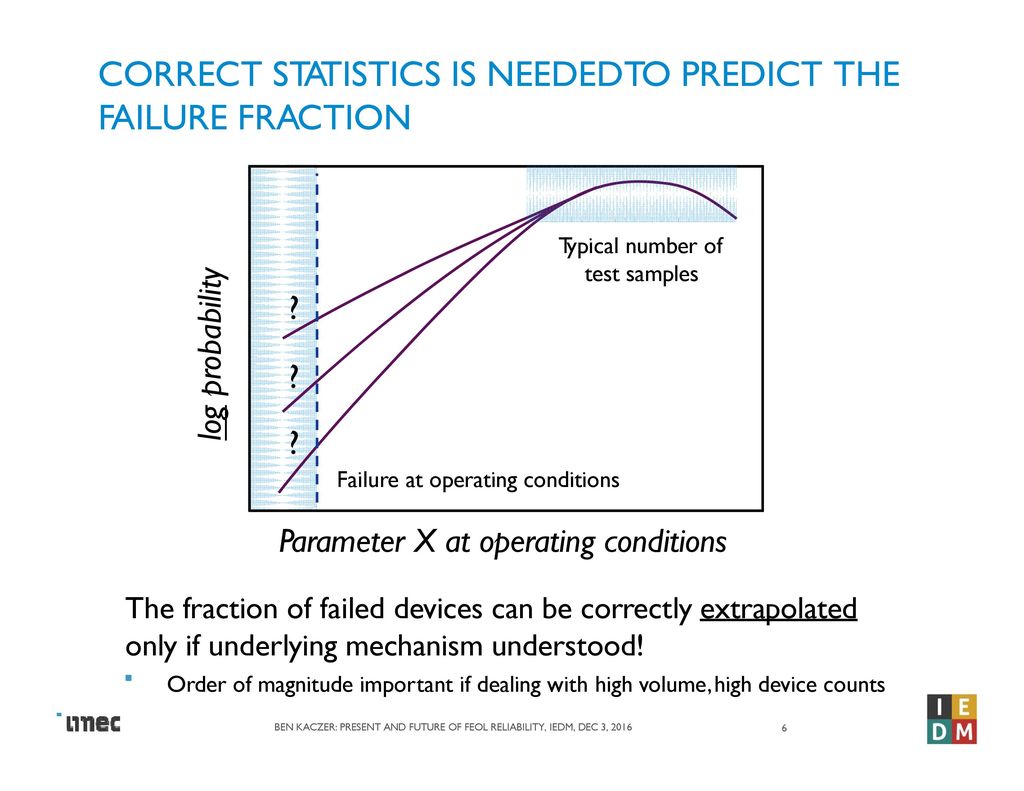
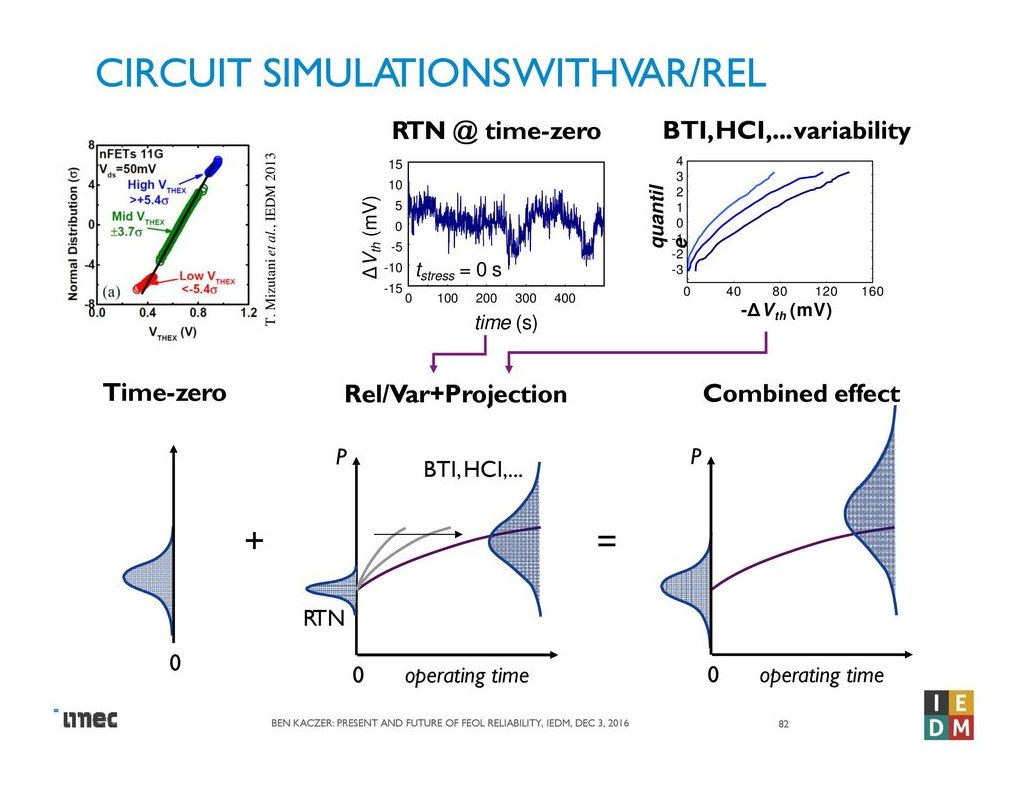
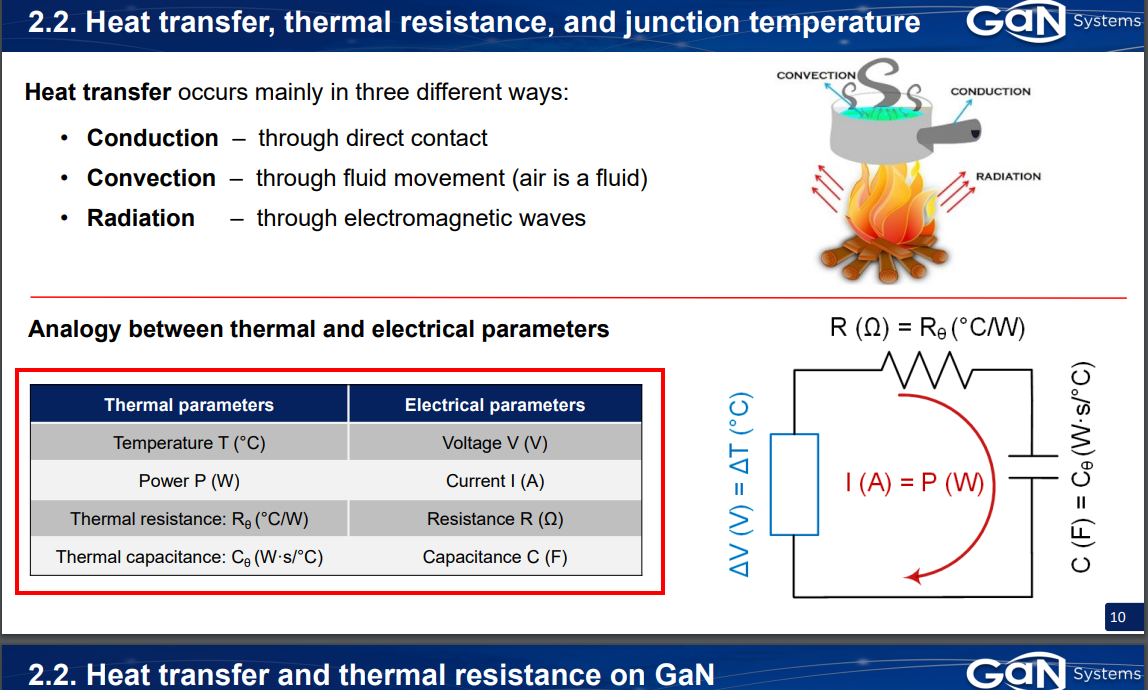
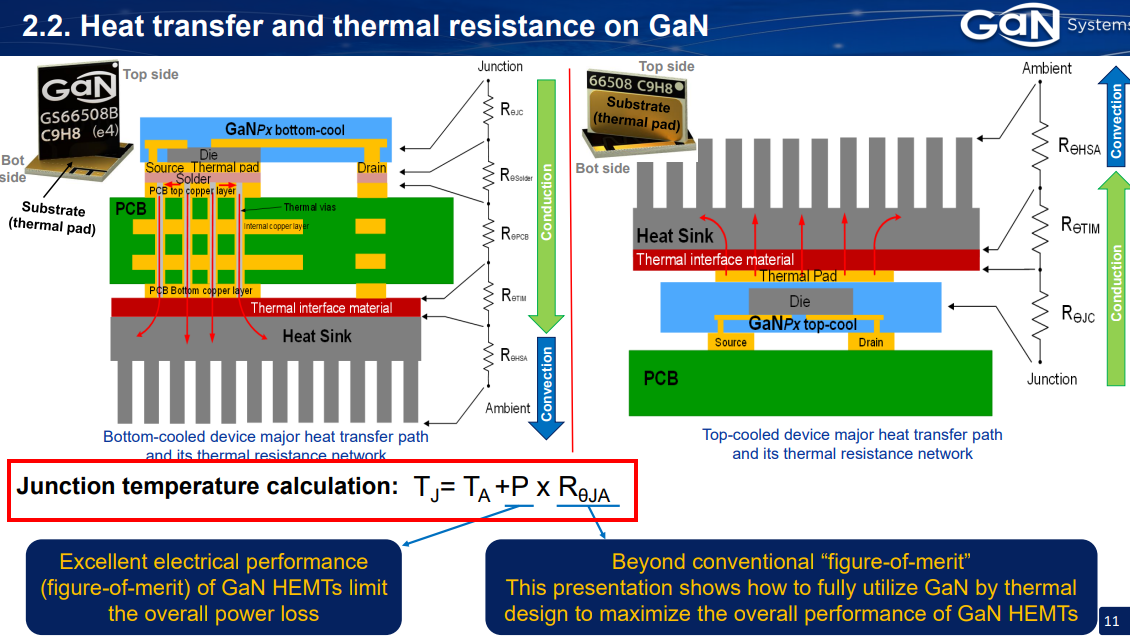
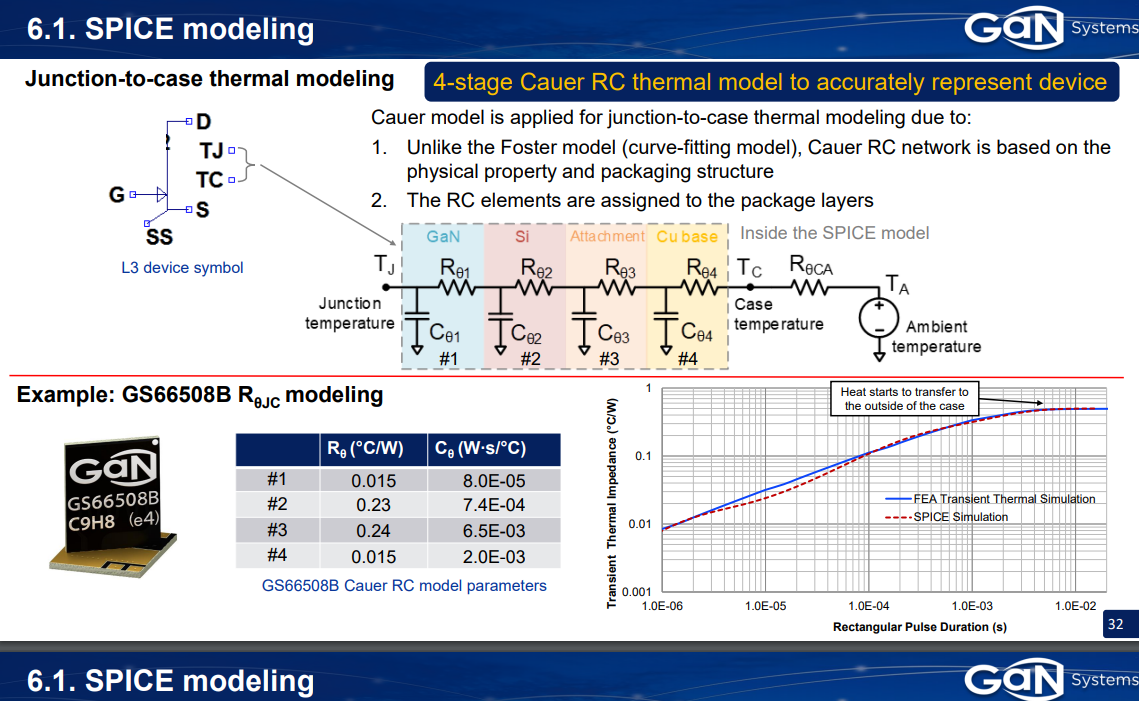
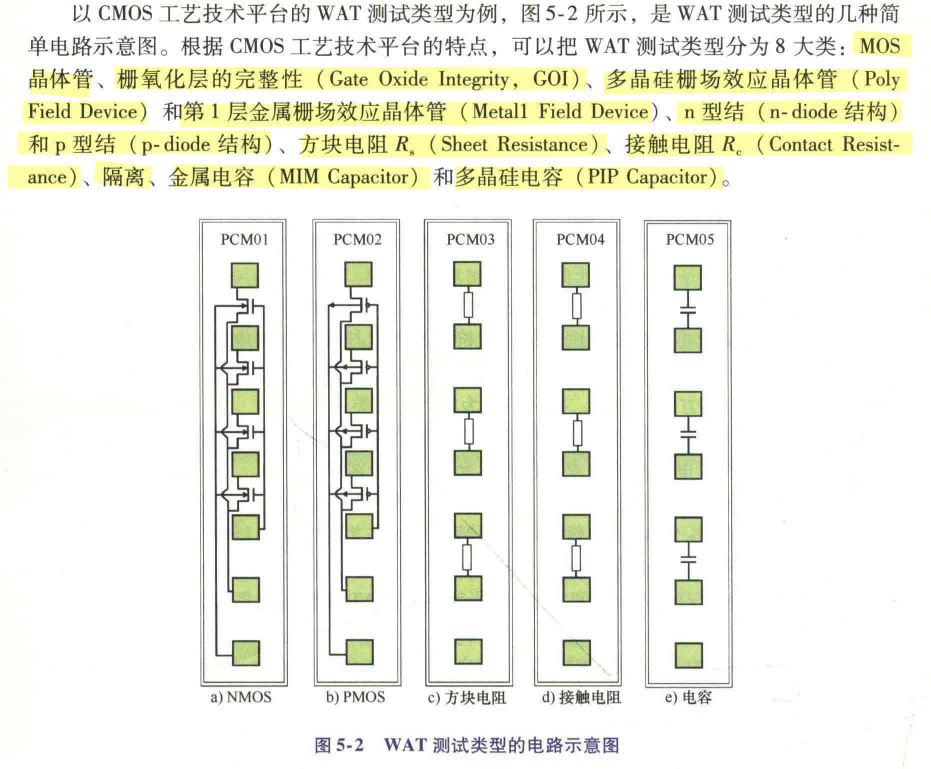
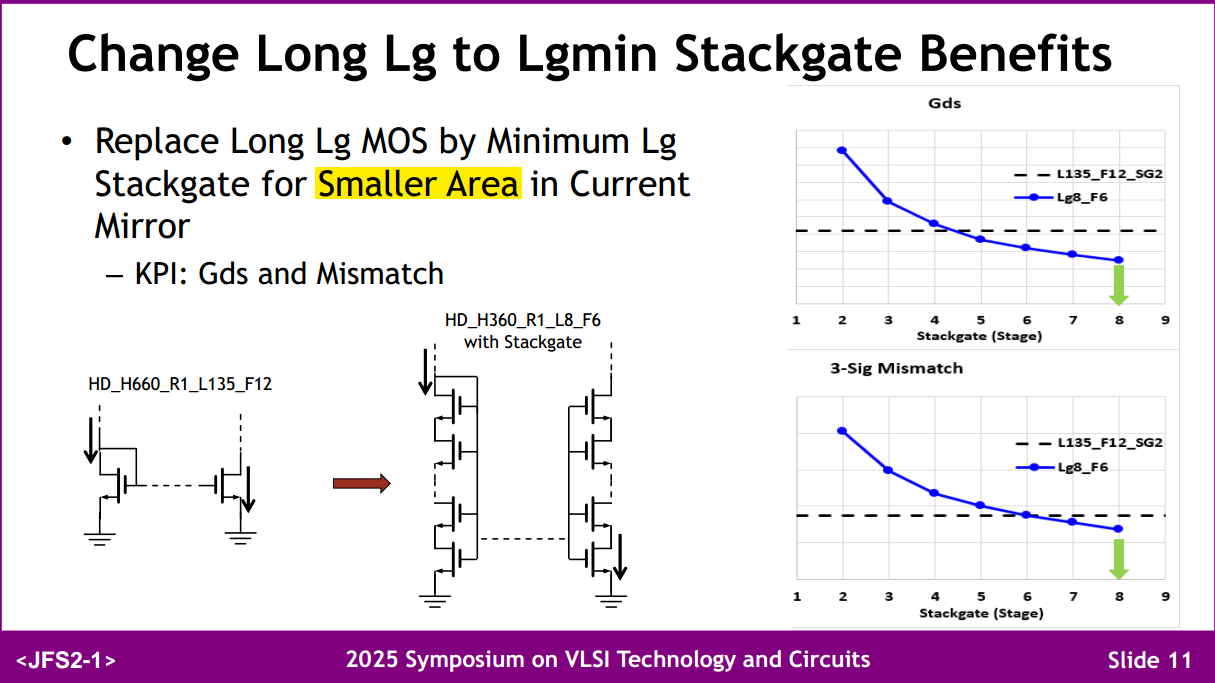
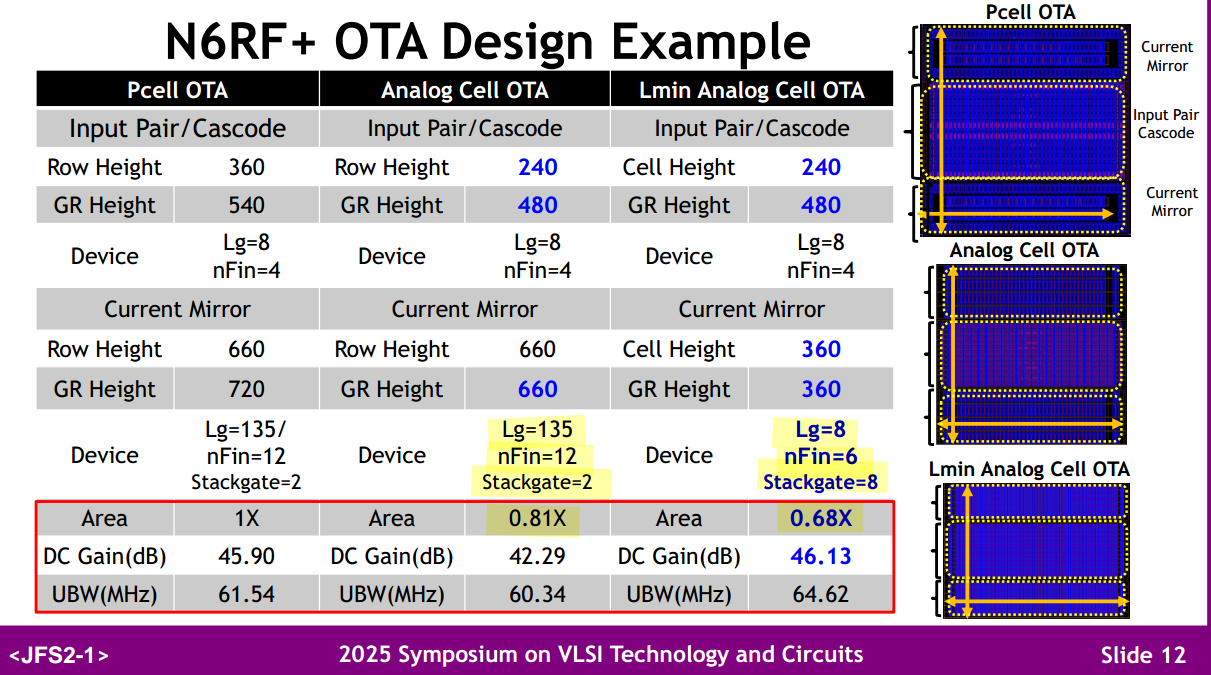
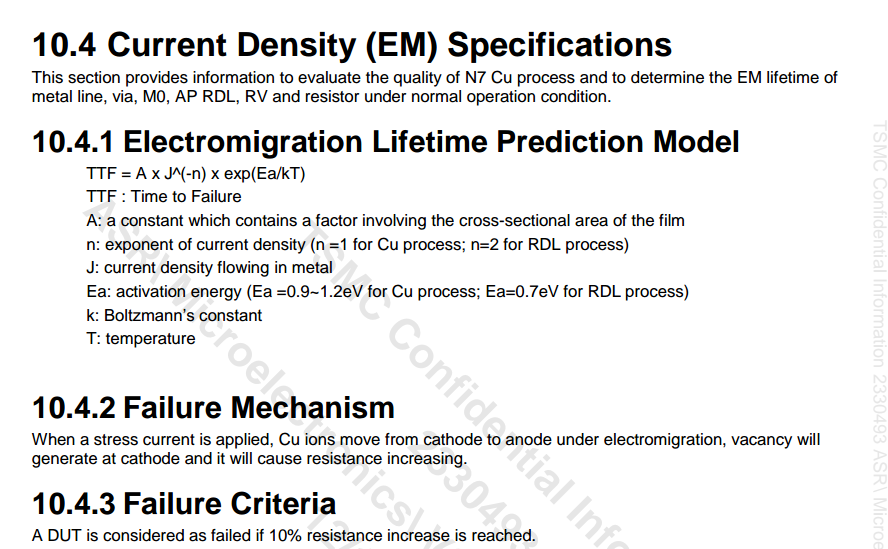
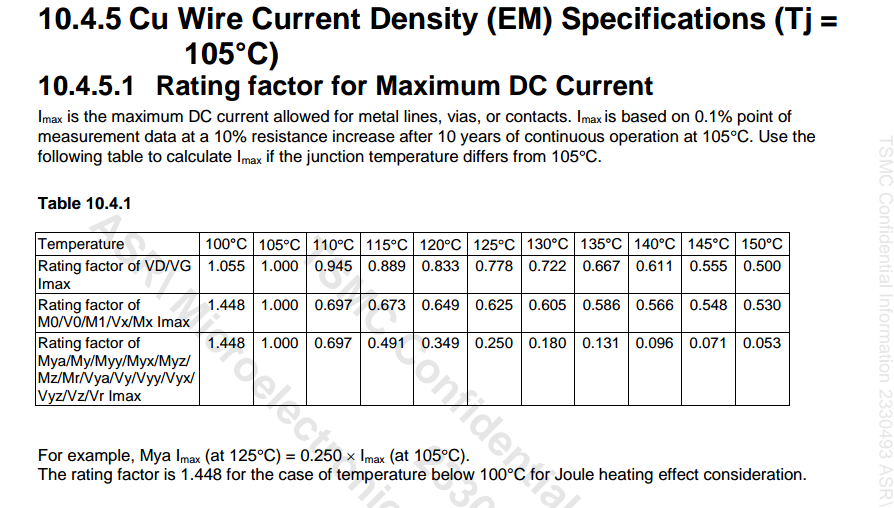
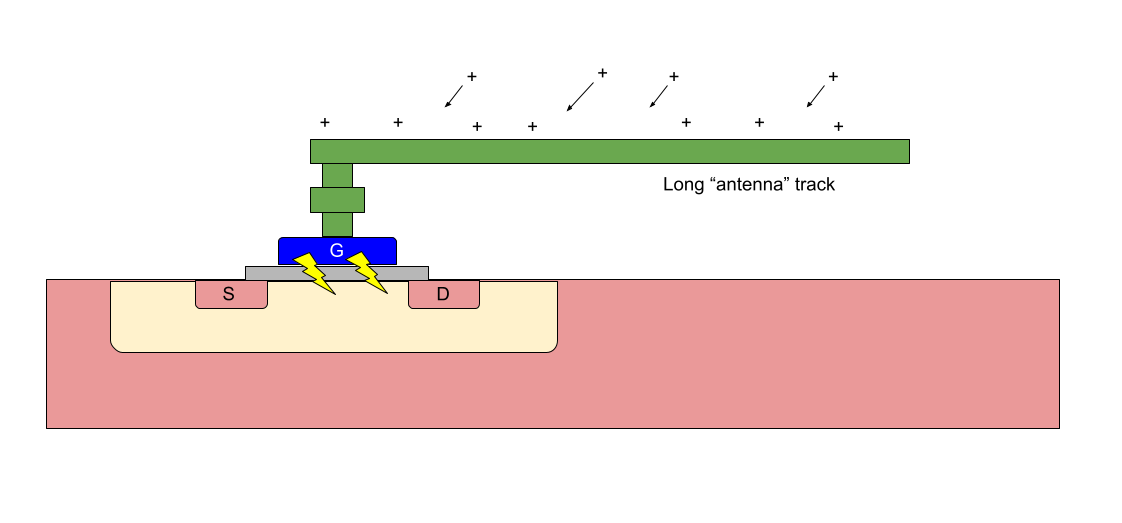
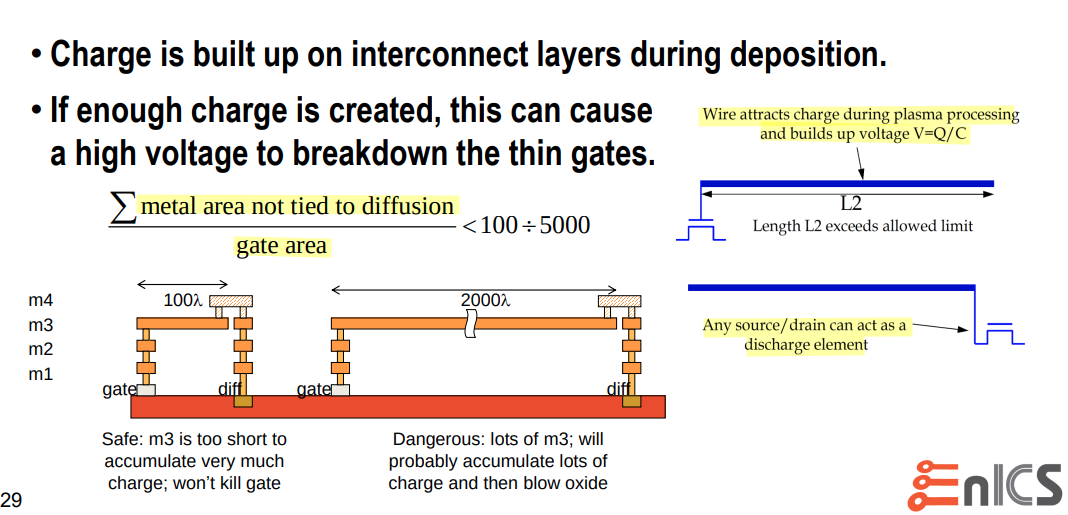
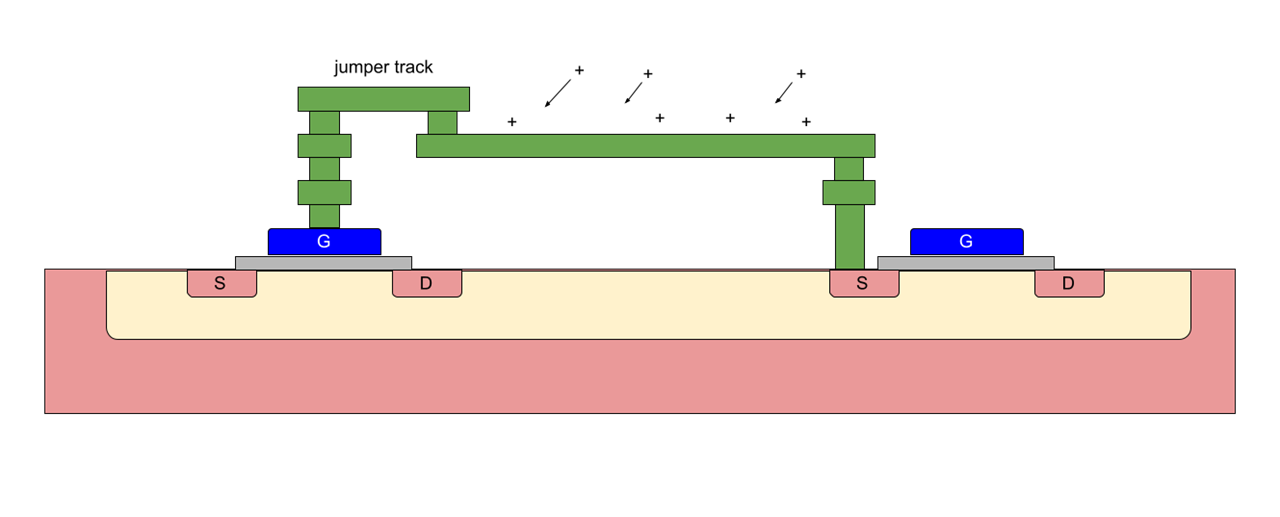
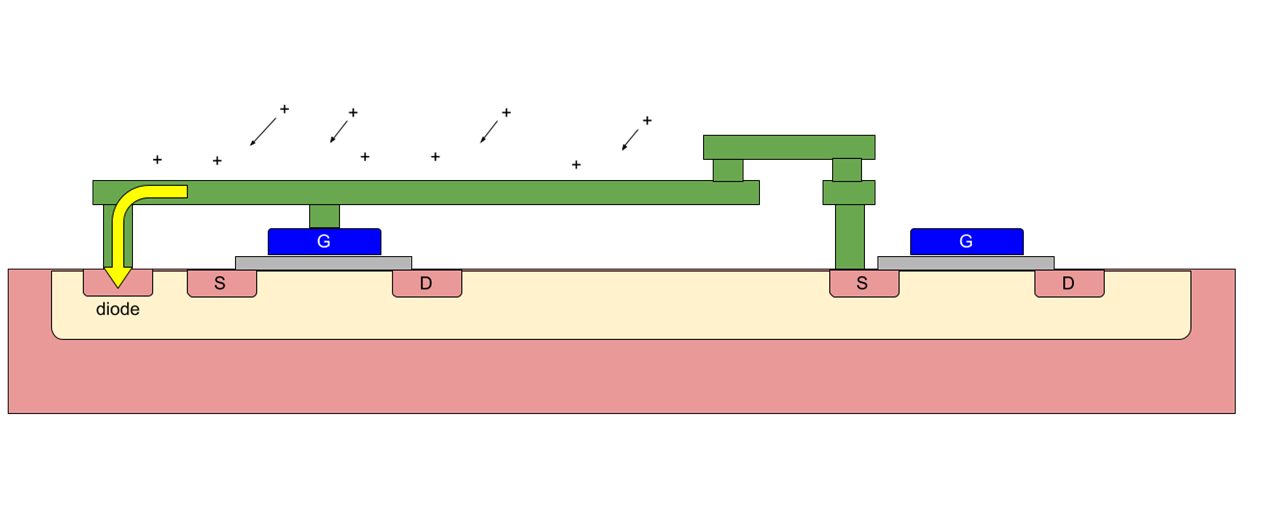
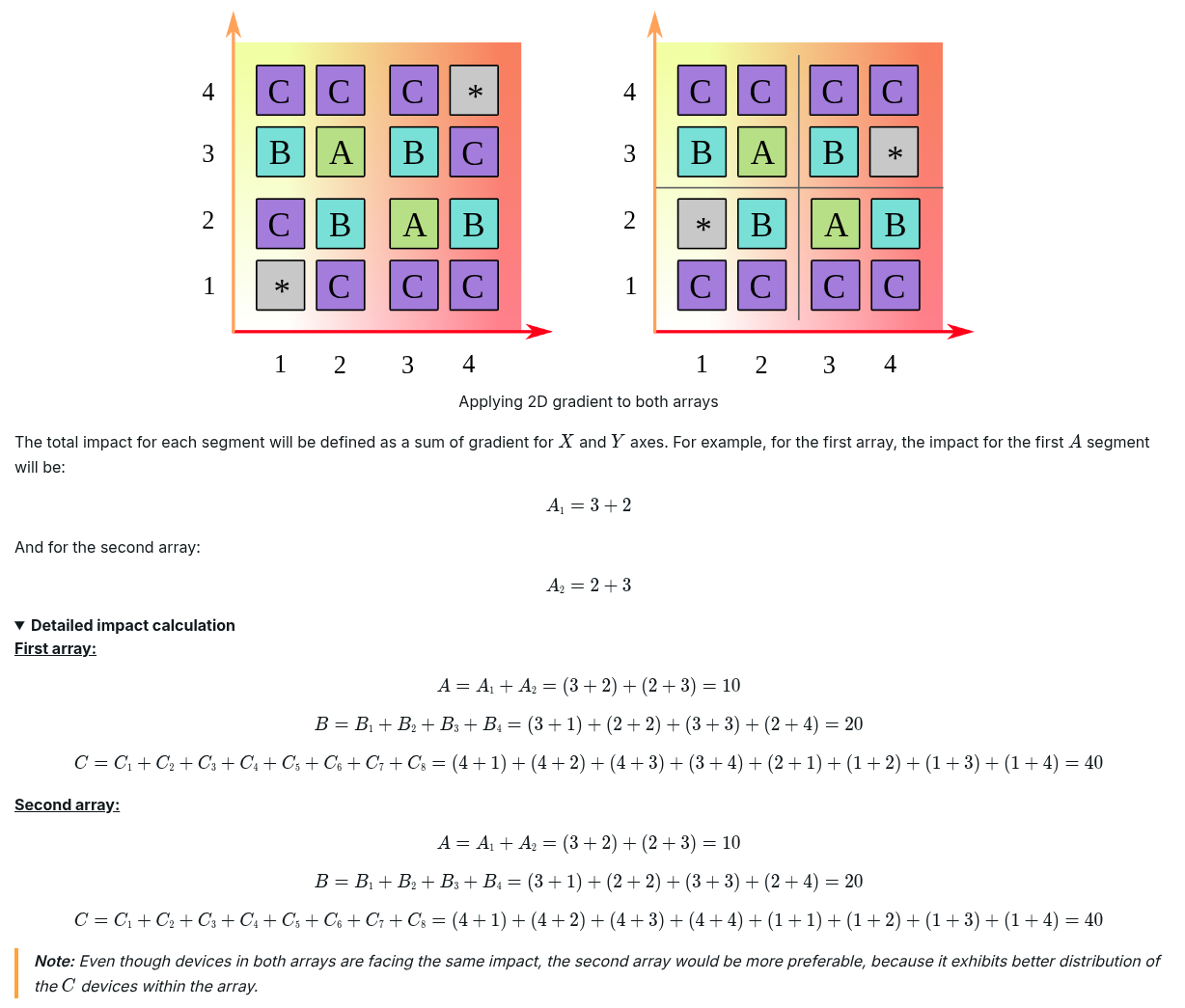
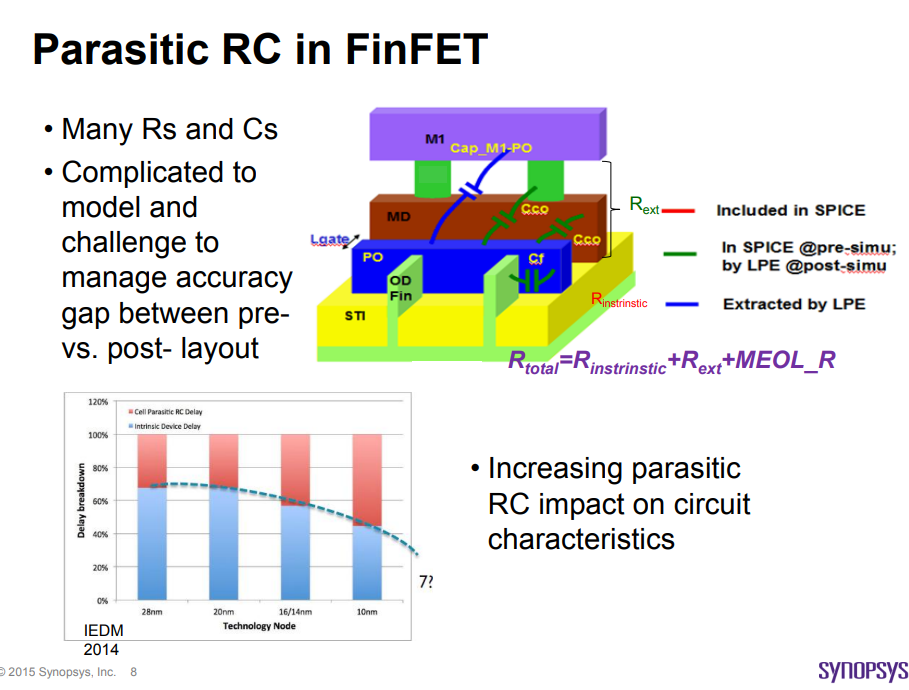
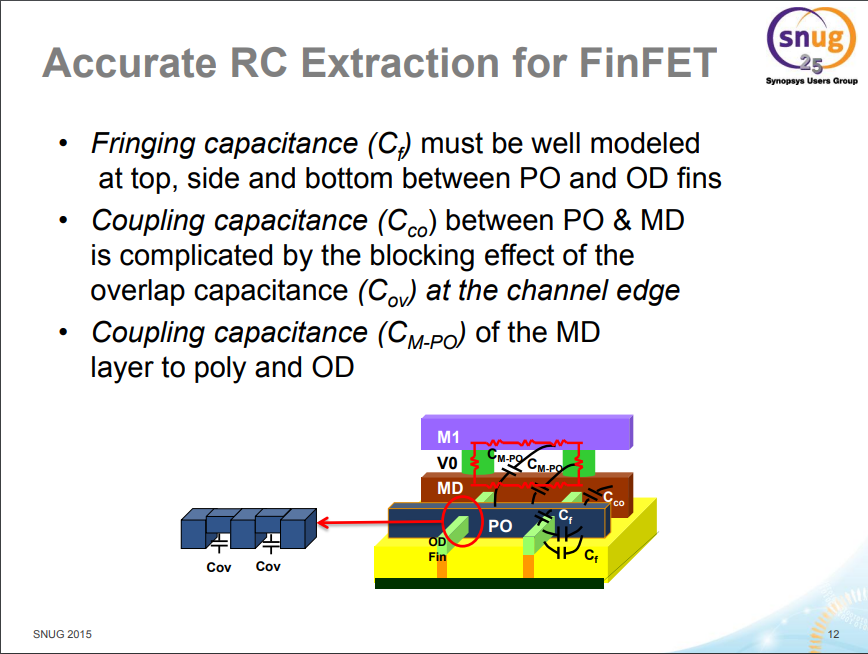
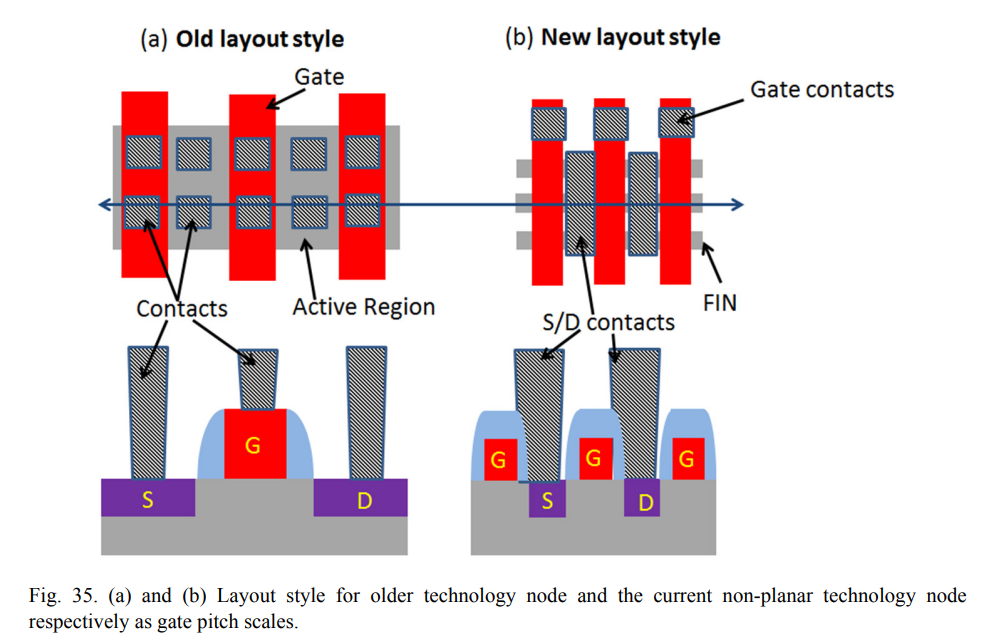
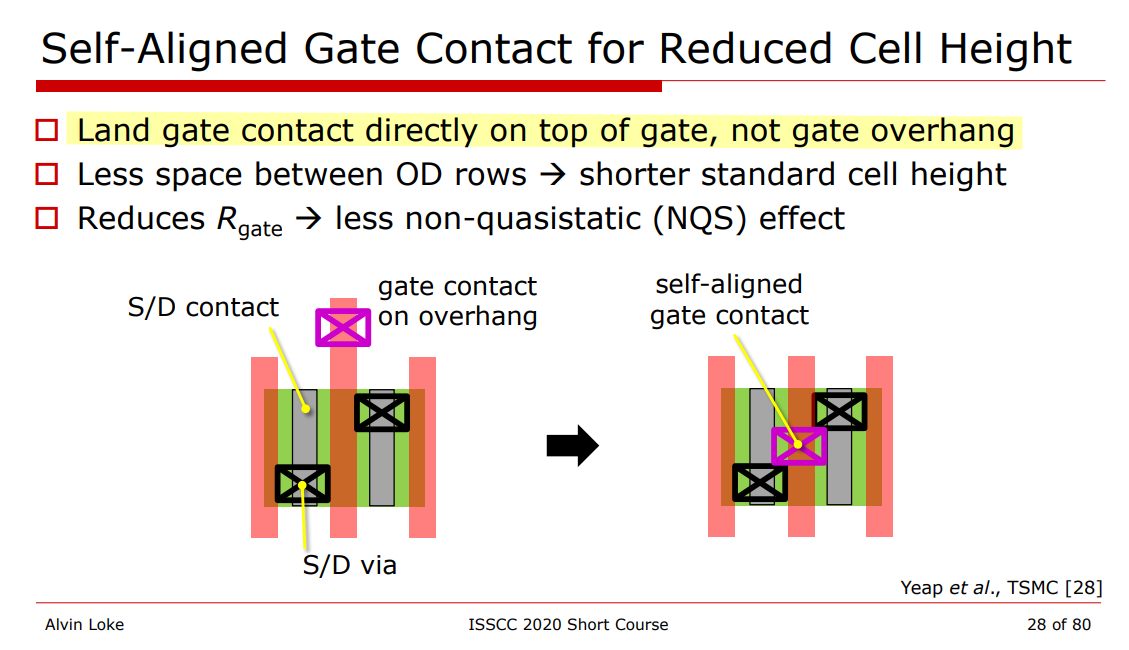
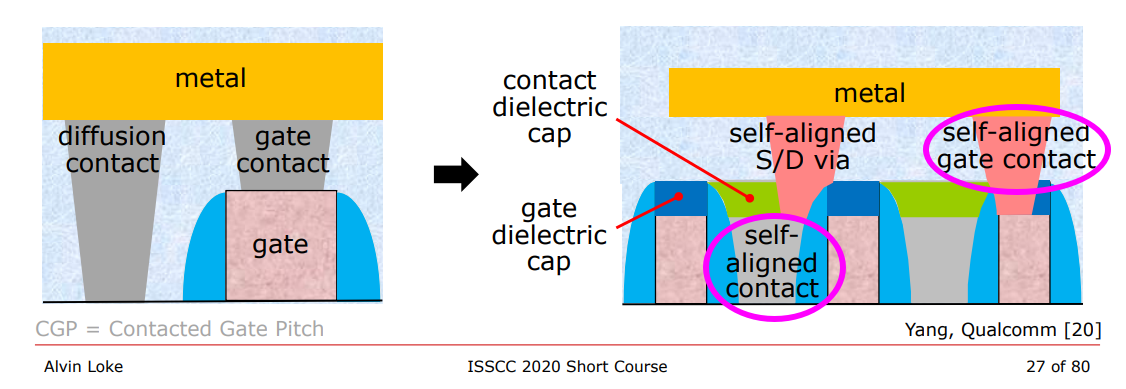
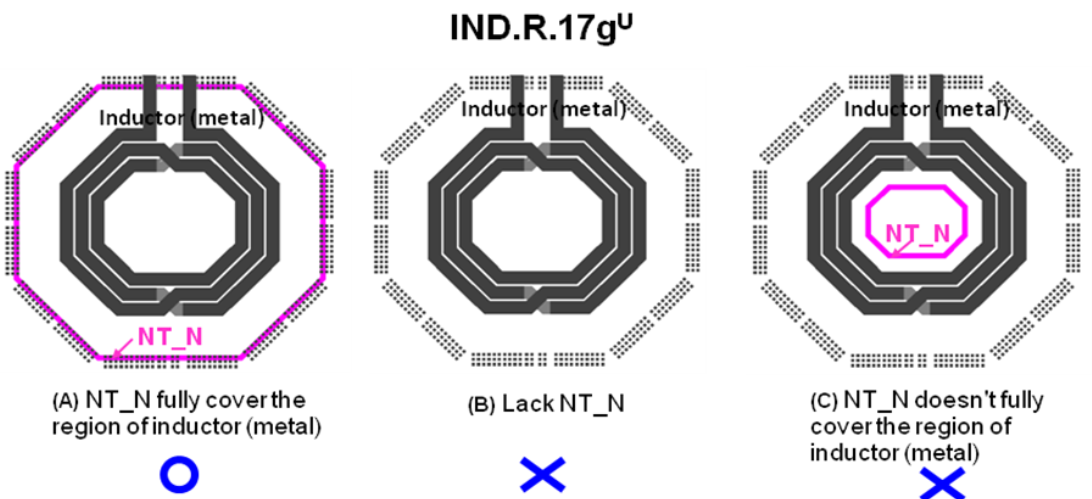
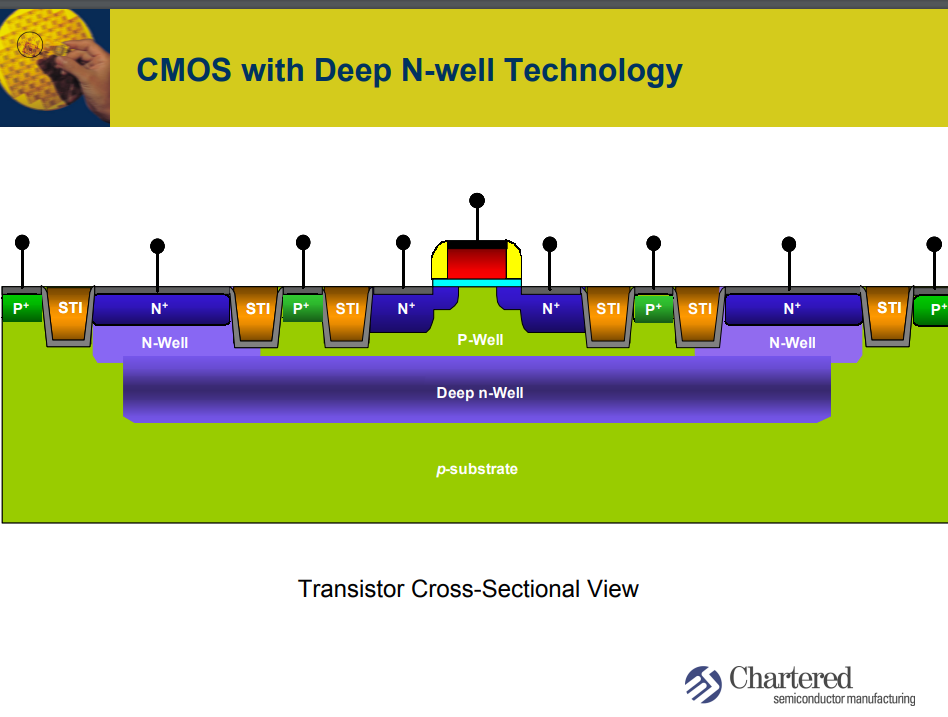
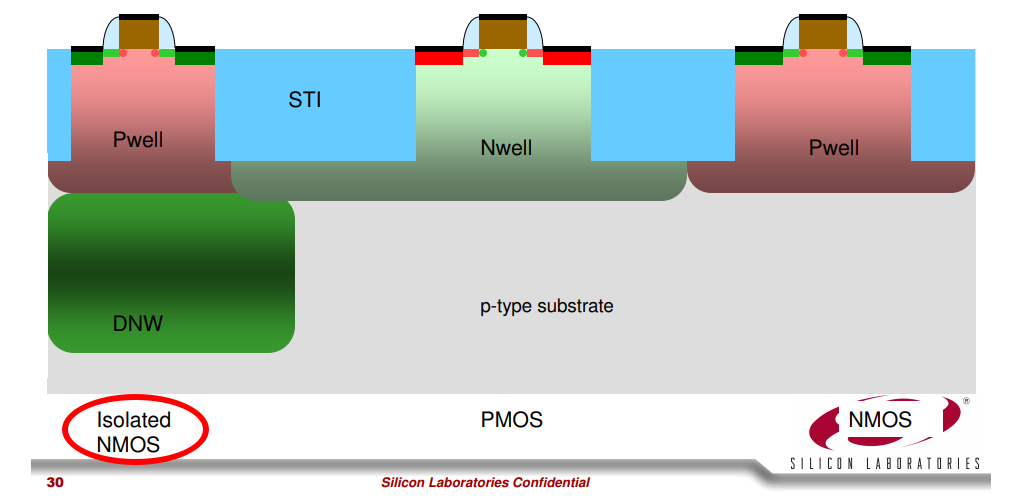
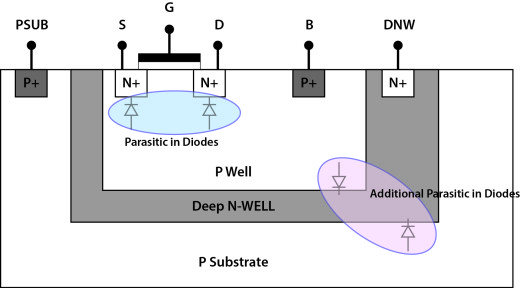
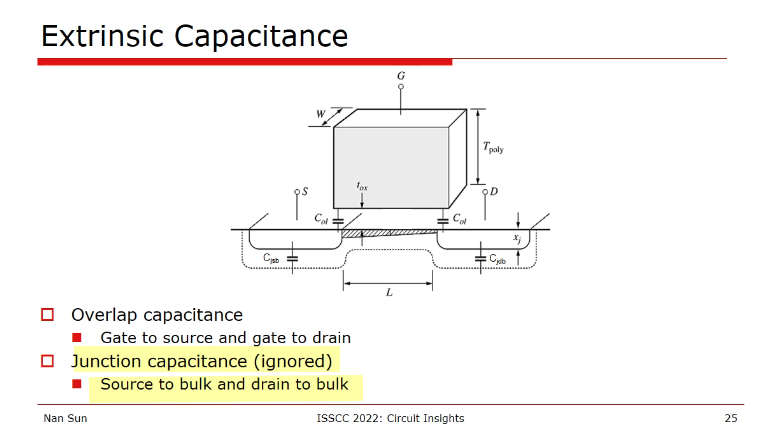
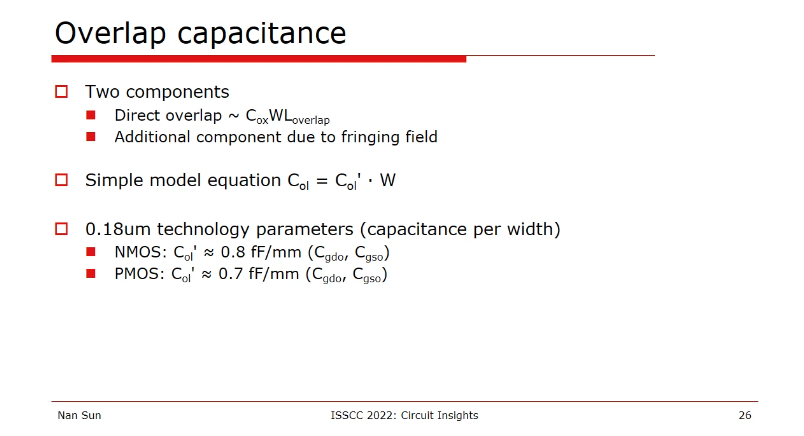
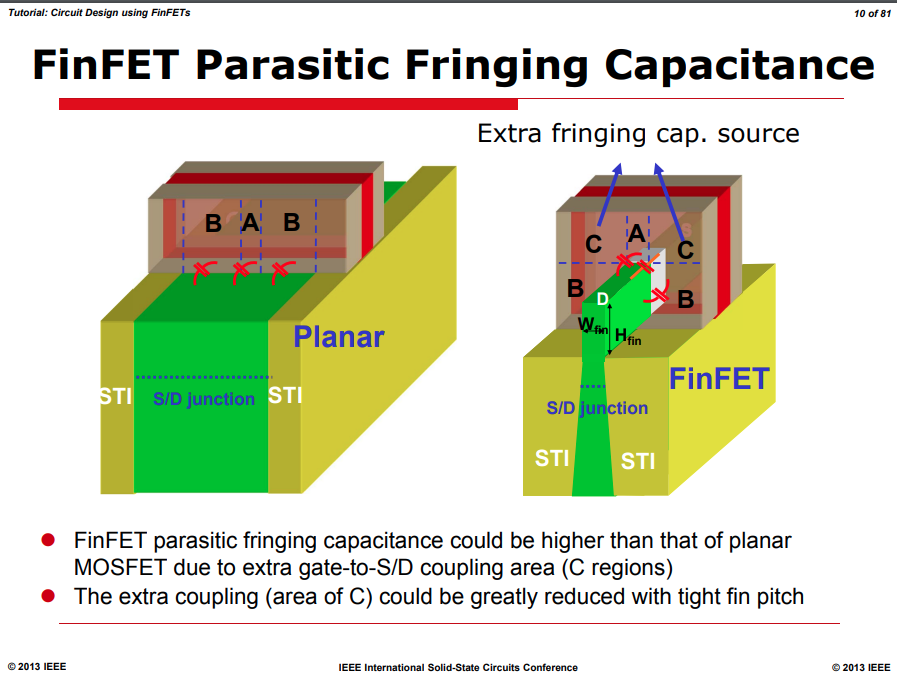
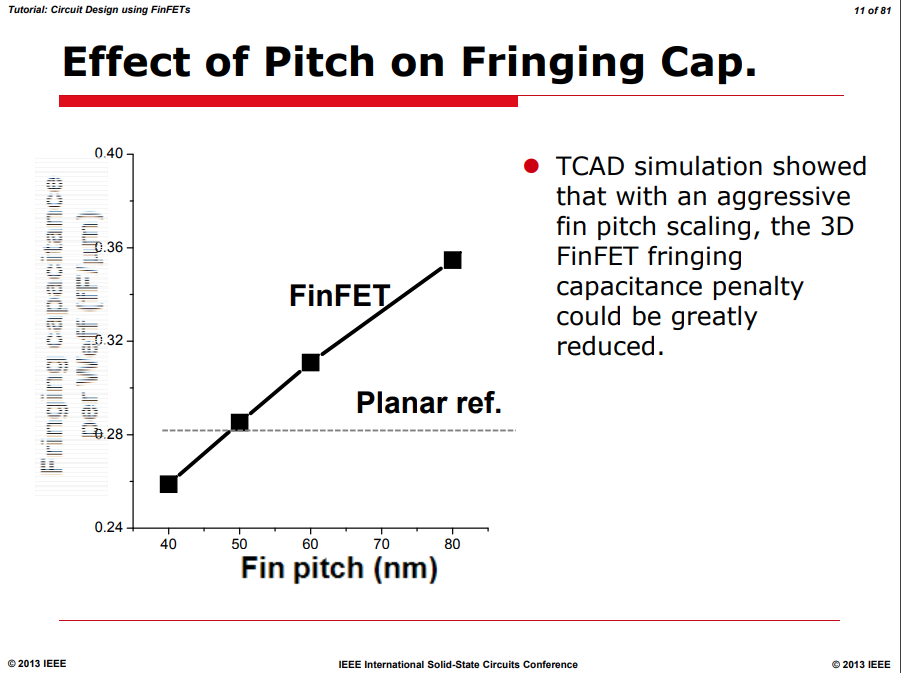
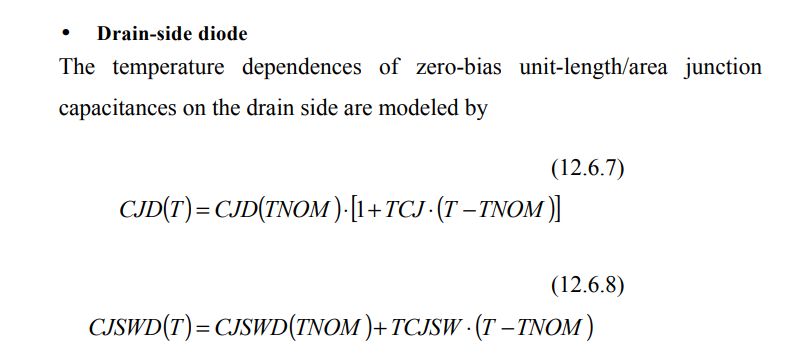
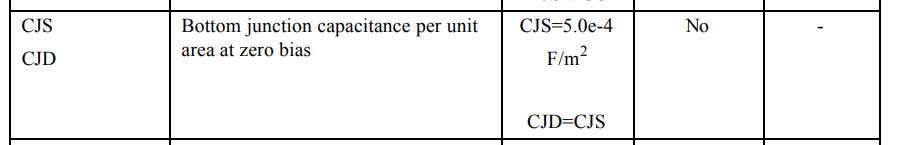
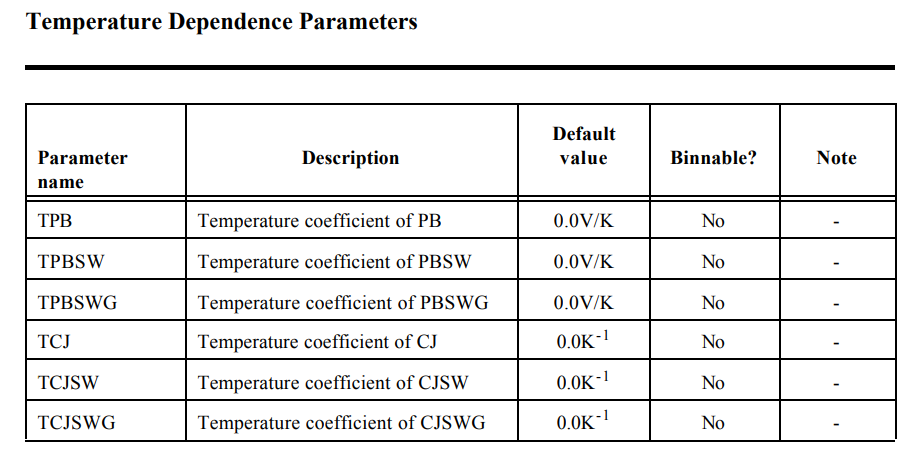
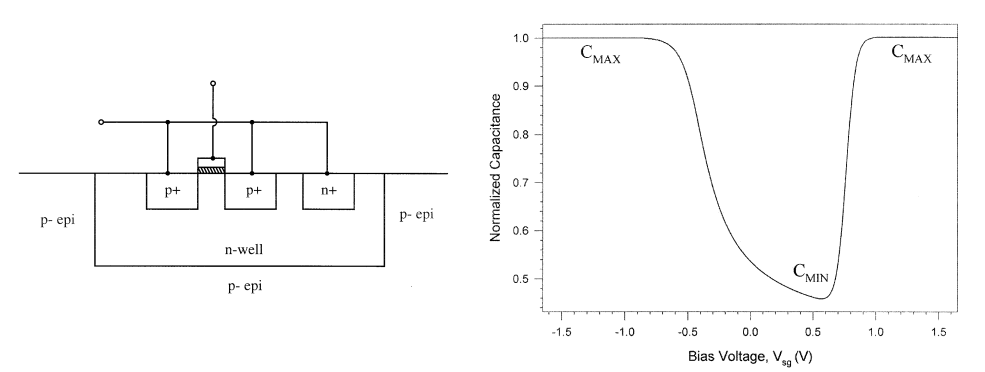
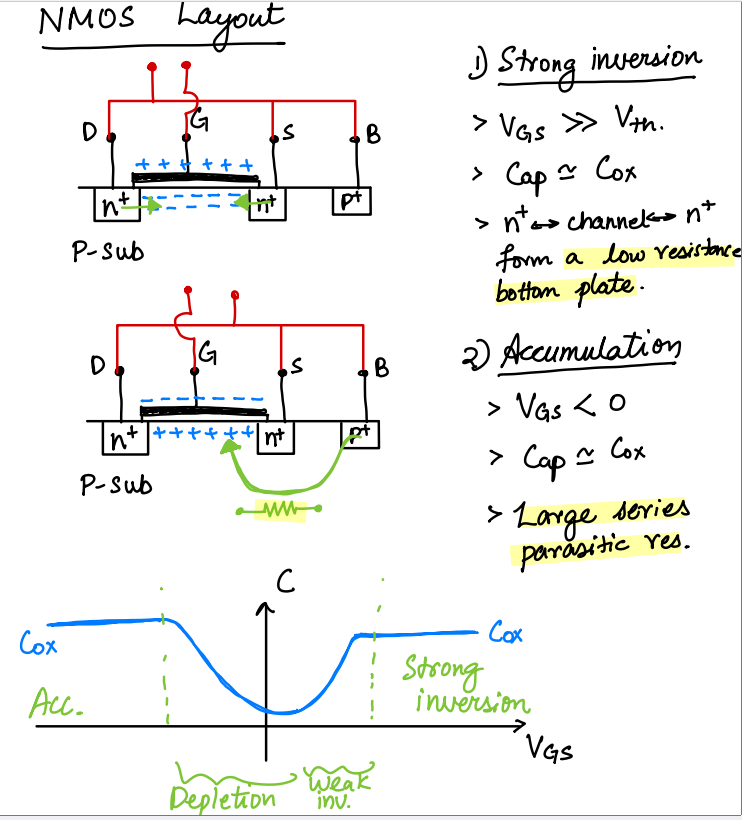
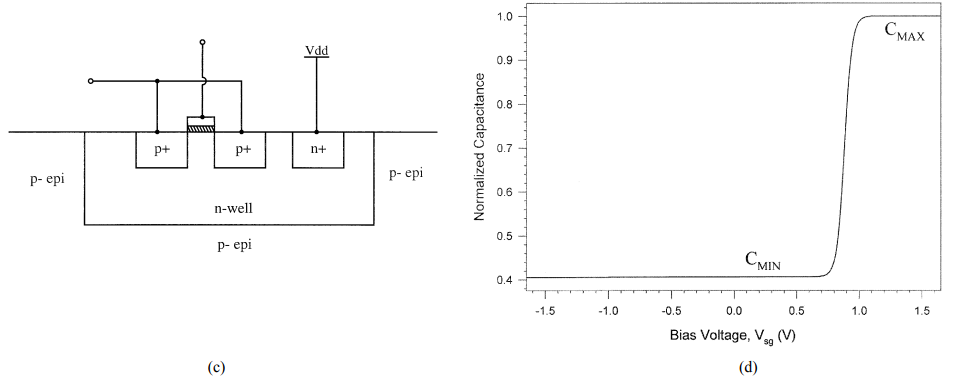
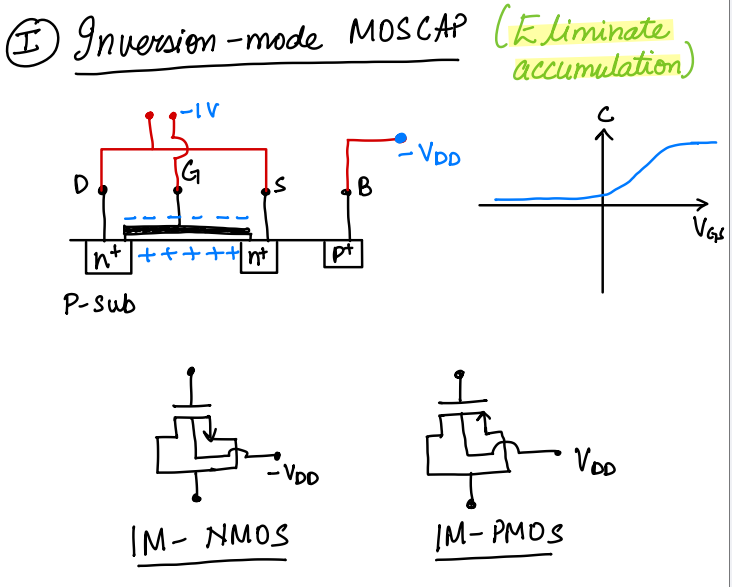
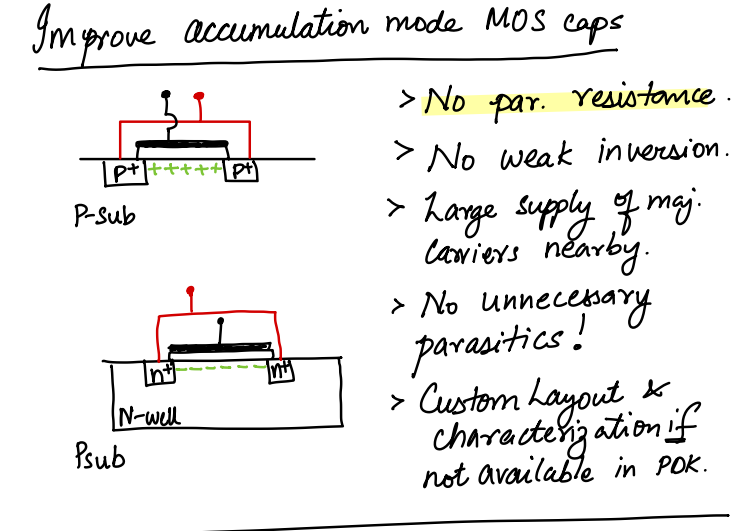
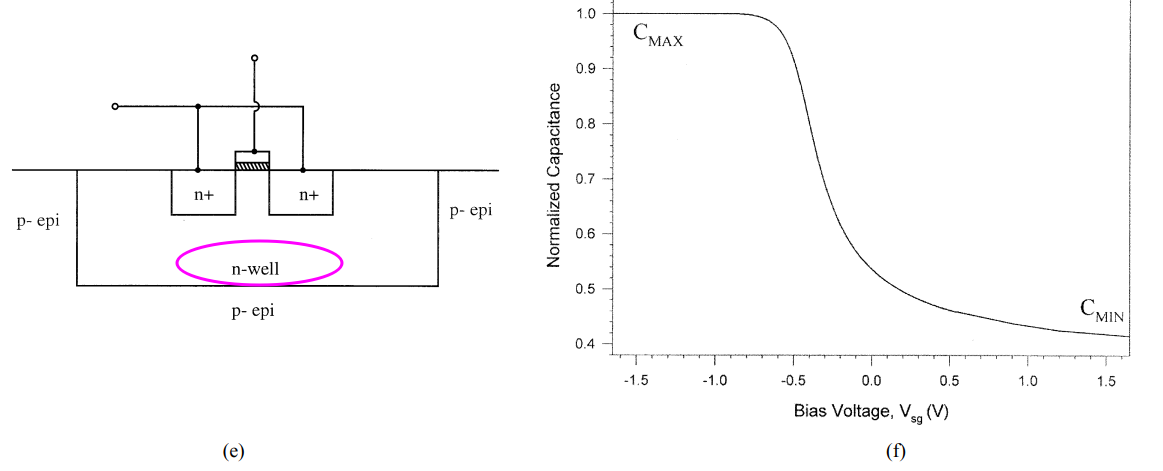
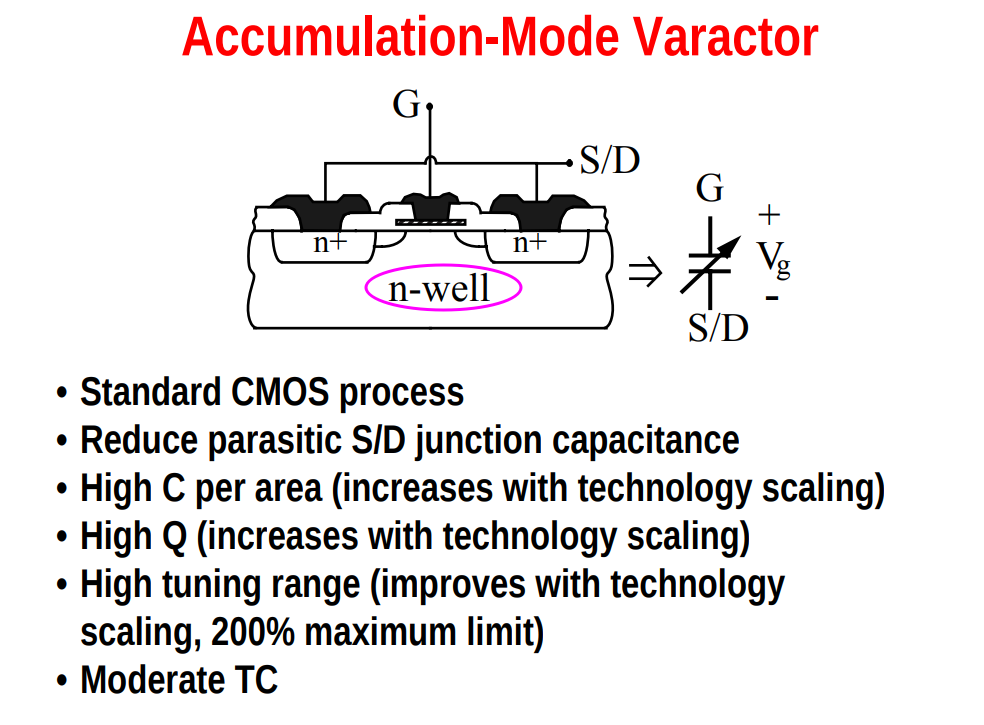
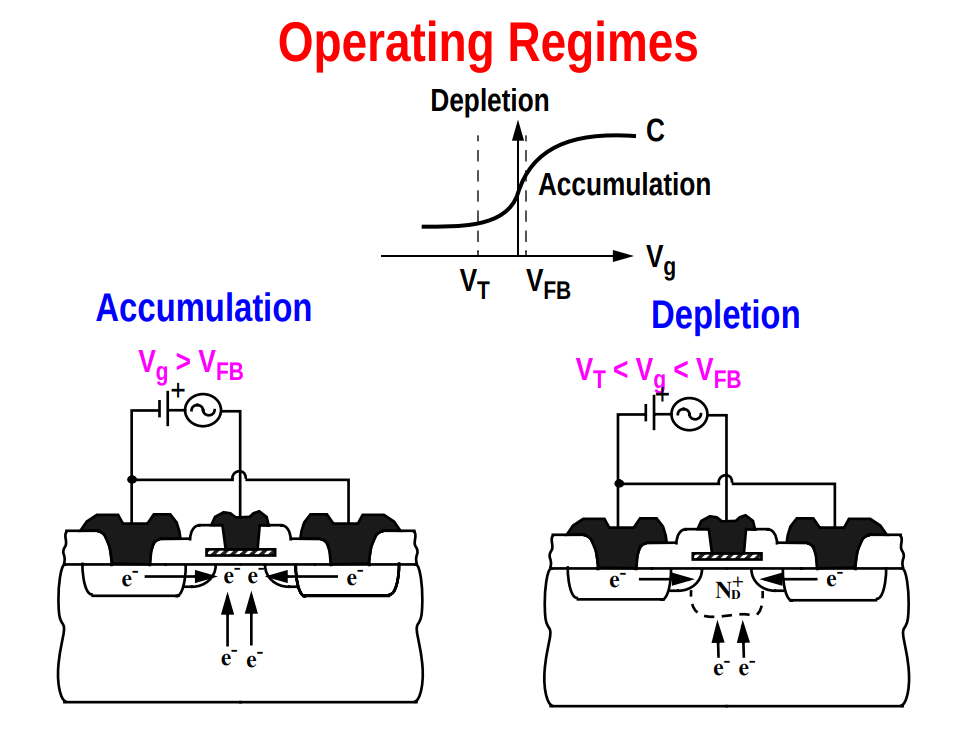
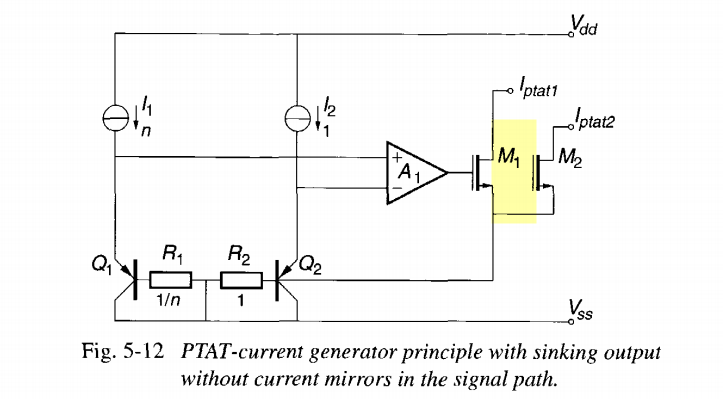
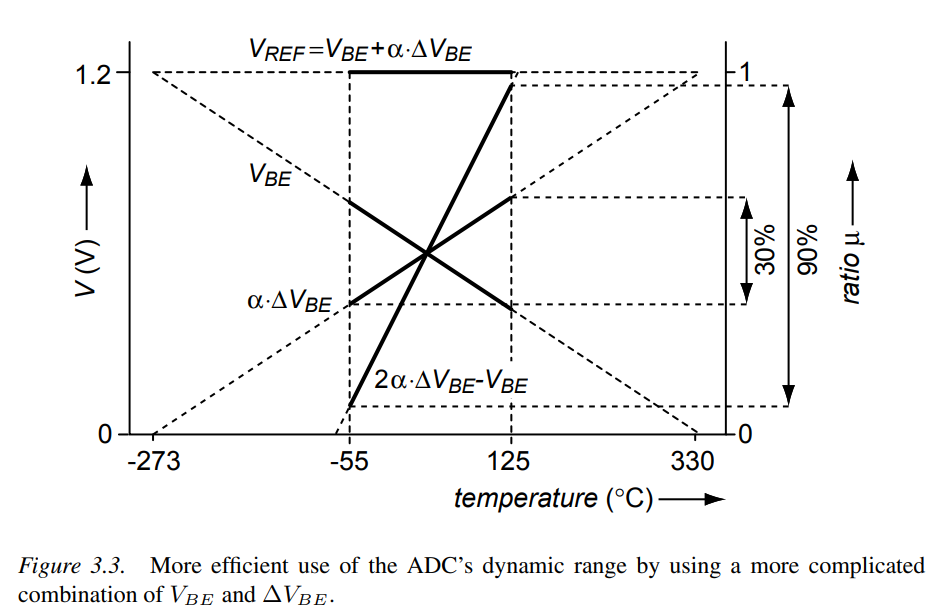
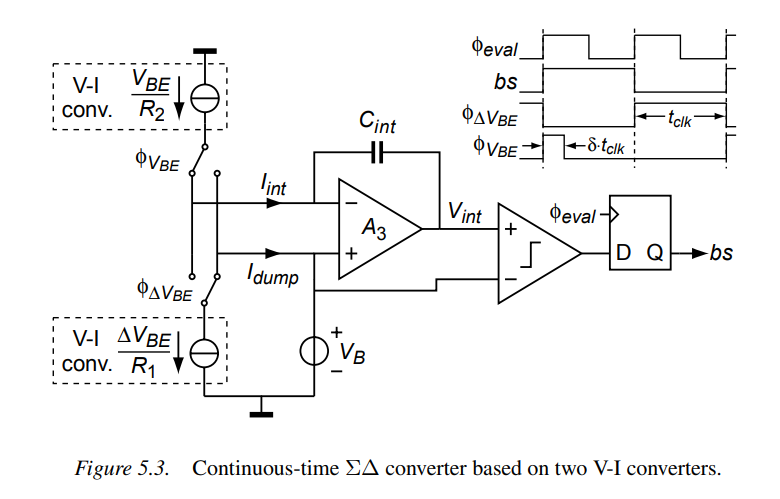
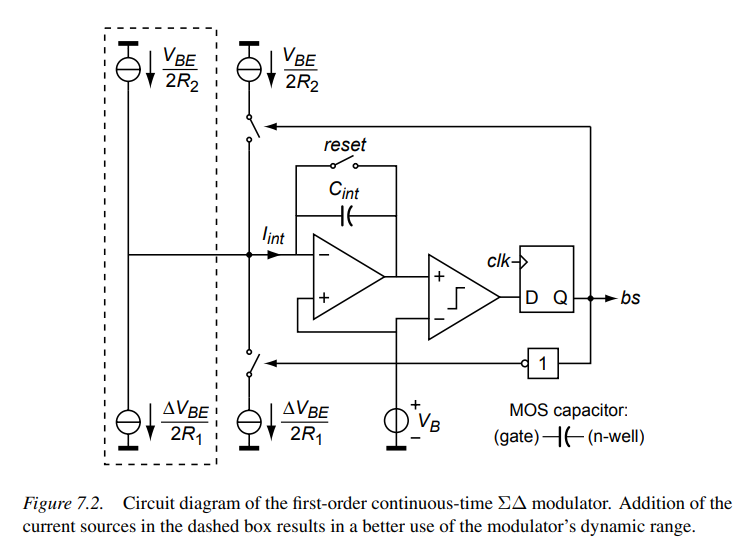
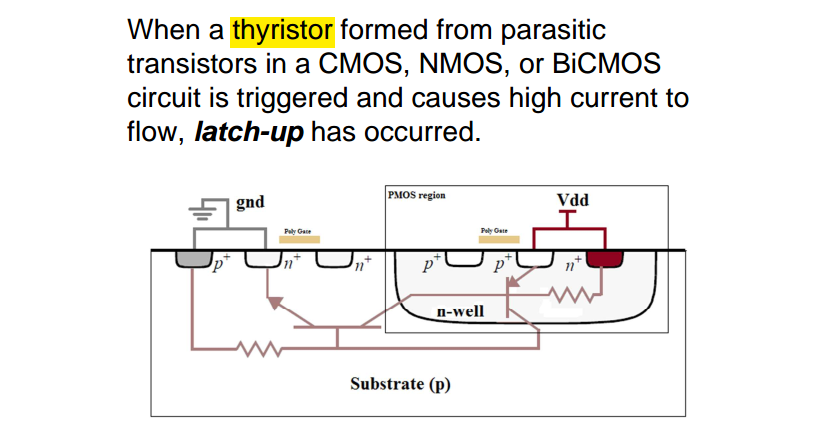
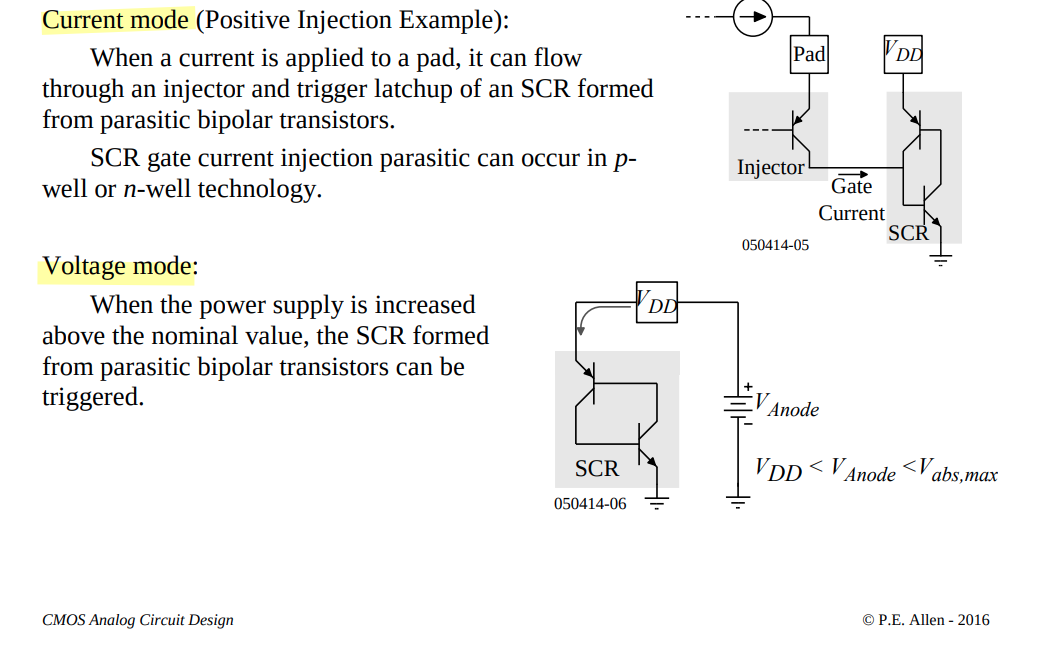
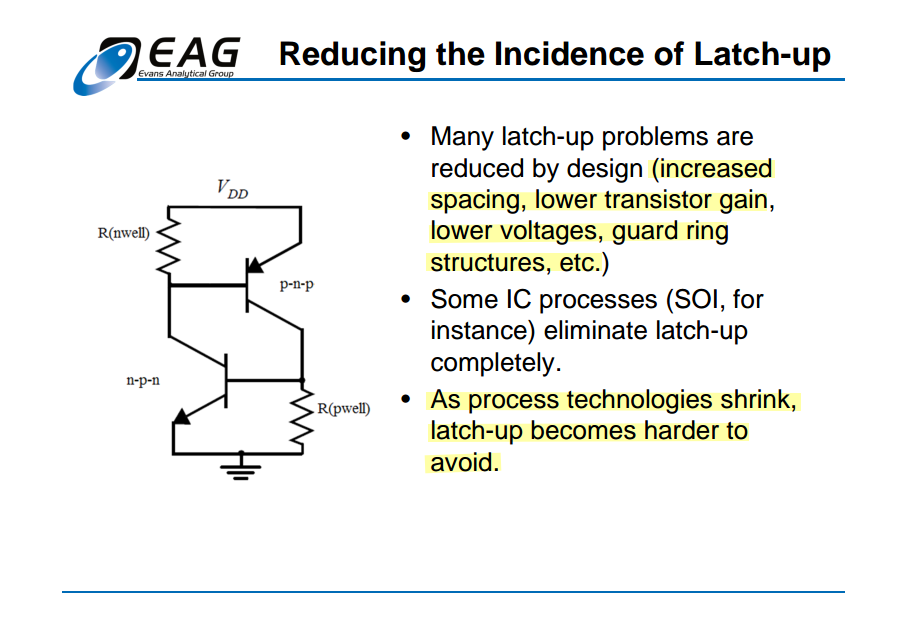
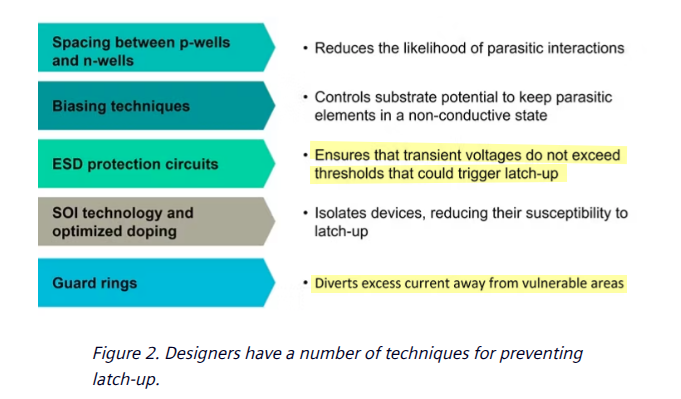
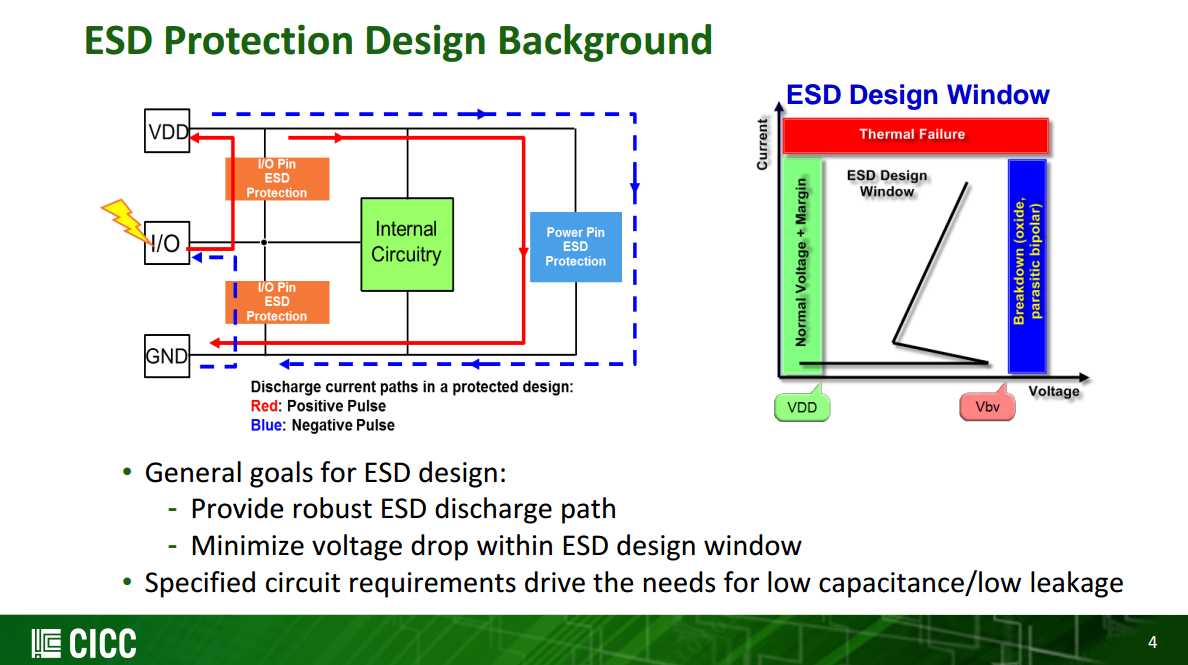
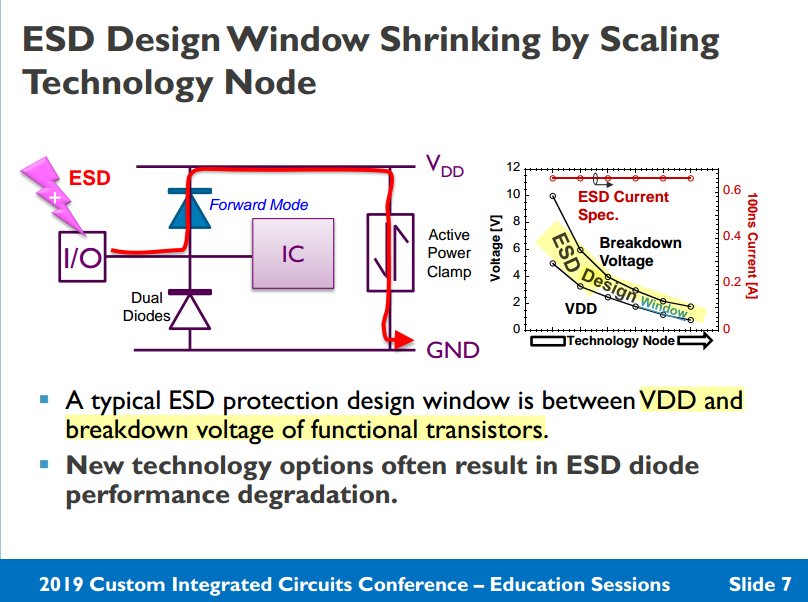
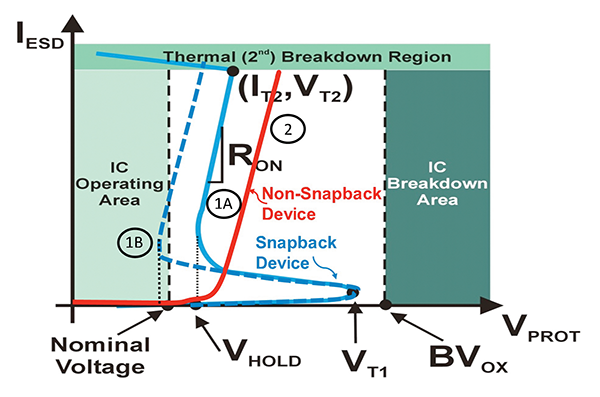
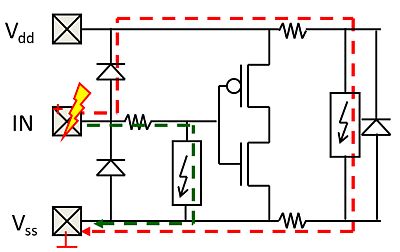
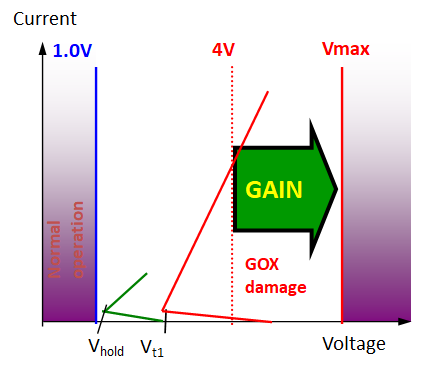
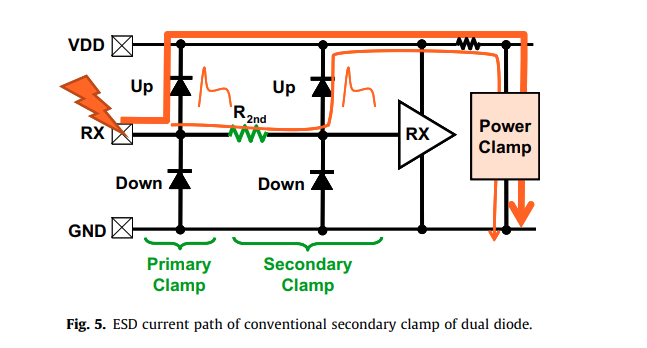
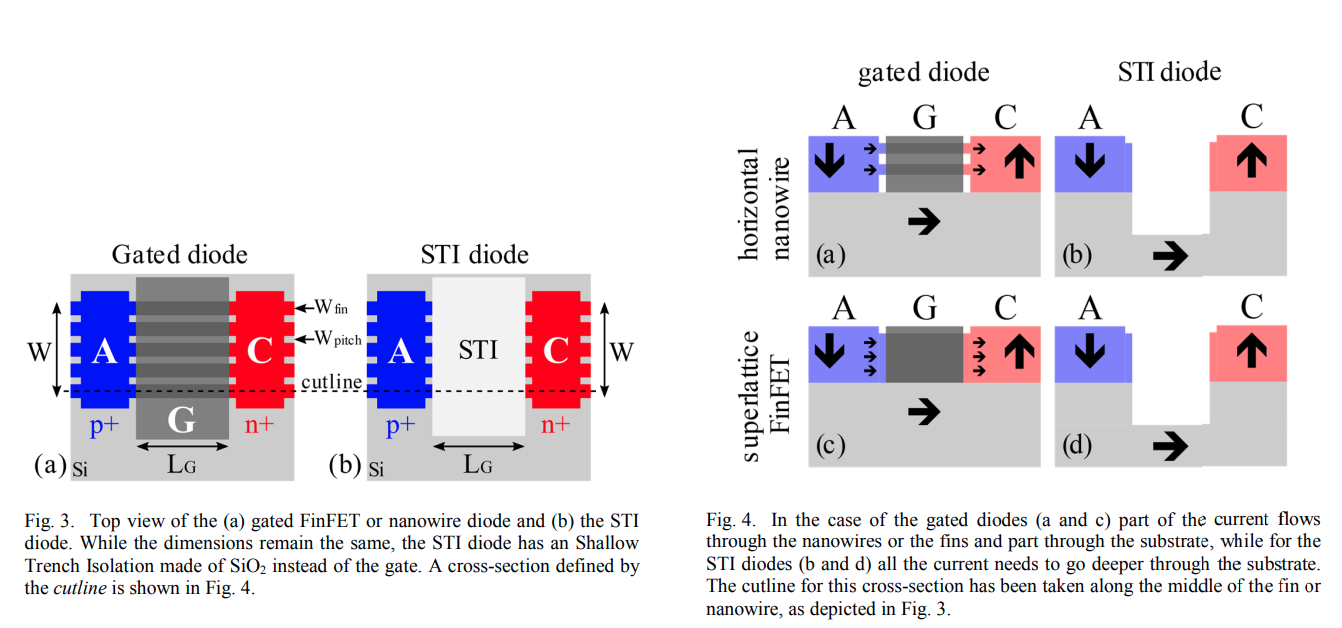
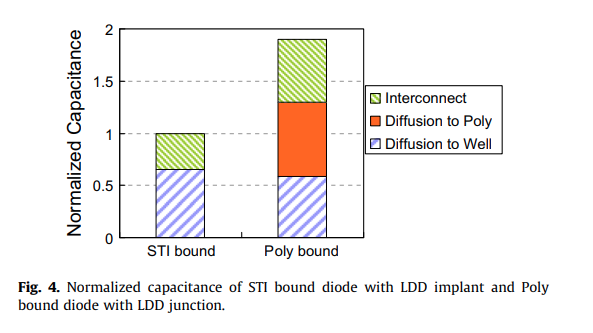
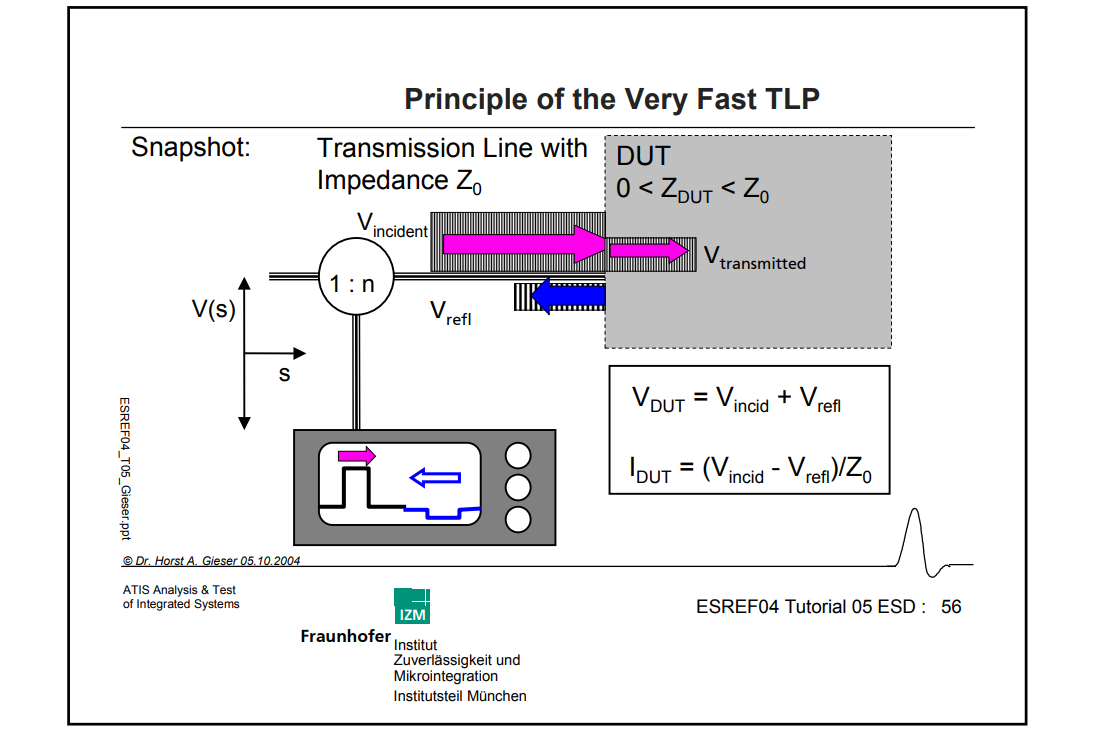
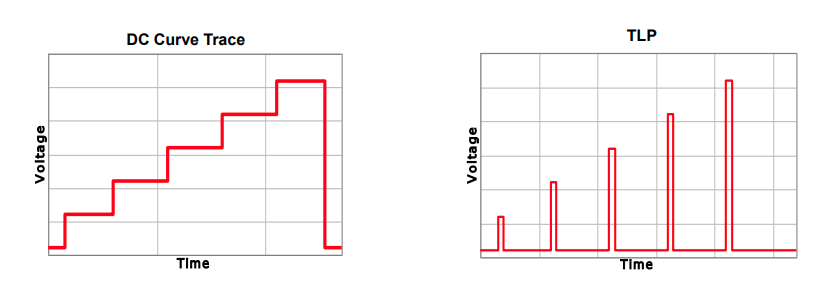
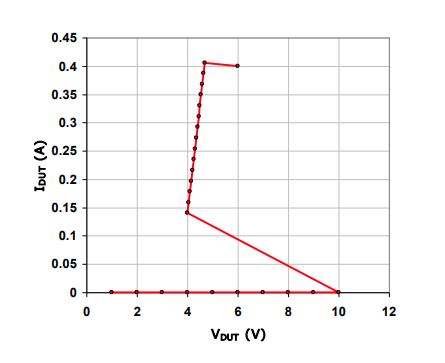
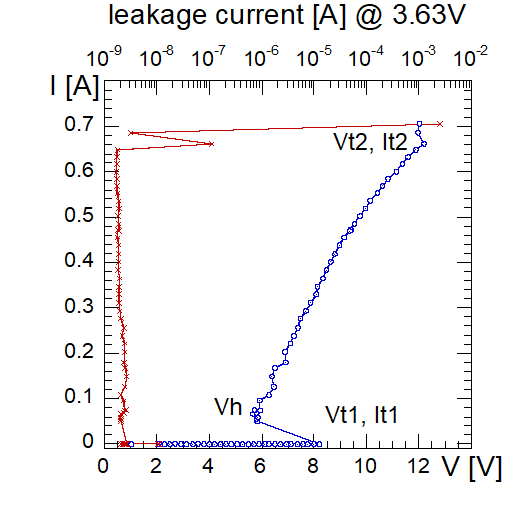
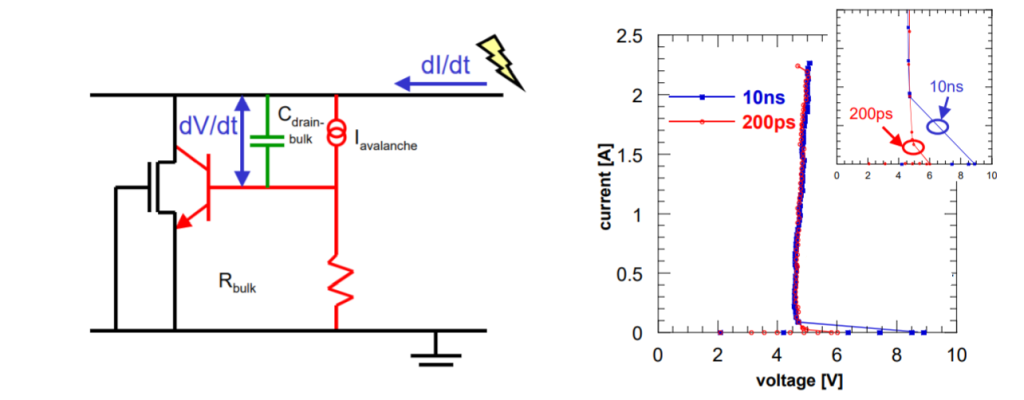
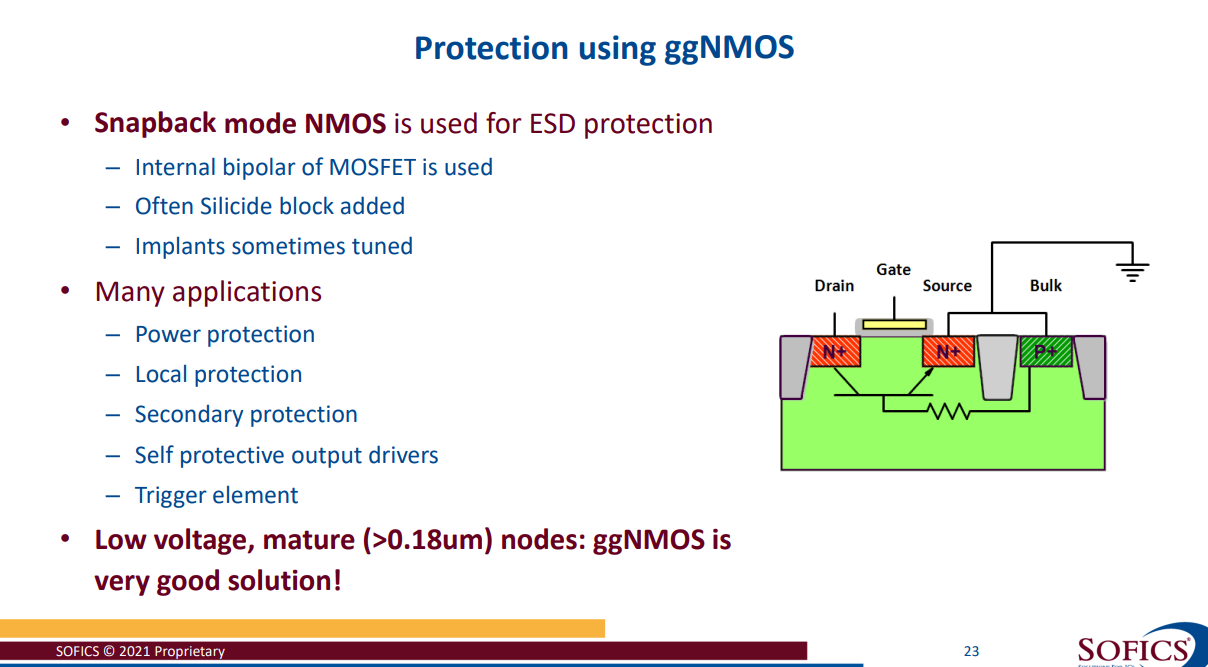
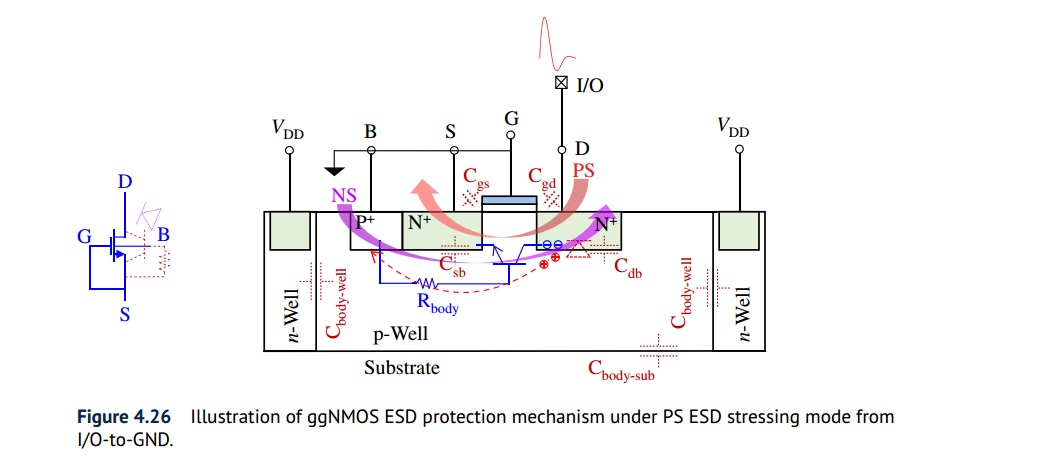
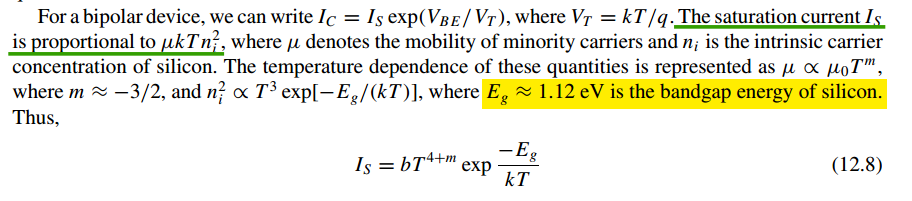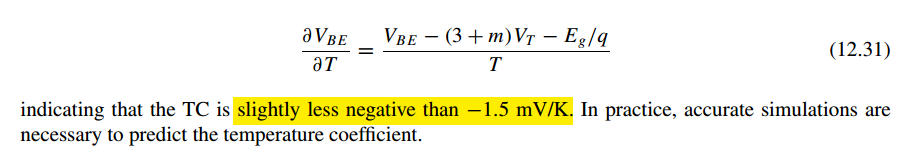Circuit insight
Gain-boosted cascode
TODO 📅
Zero-Value Time Constant Analysis
TODO 📅
Transmission Gate
Equivalent Resistance is defined by large signal
[https://www.ece.ucdavis.edu/~ramirtha/EEC116/F11/TGlecture.pdf]
Why 50-ohm?
Why is 50-ohm characteristic impedance standardized in RF and Microwave systems? [link]
[https://www.microwaves101.com/encyclopedias/why-fifty-ohms]
It was a compromise made and a tradeoff between power handling (red curve) and losses (blue curve)

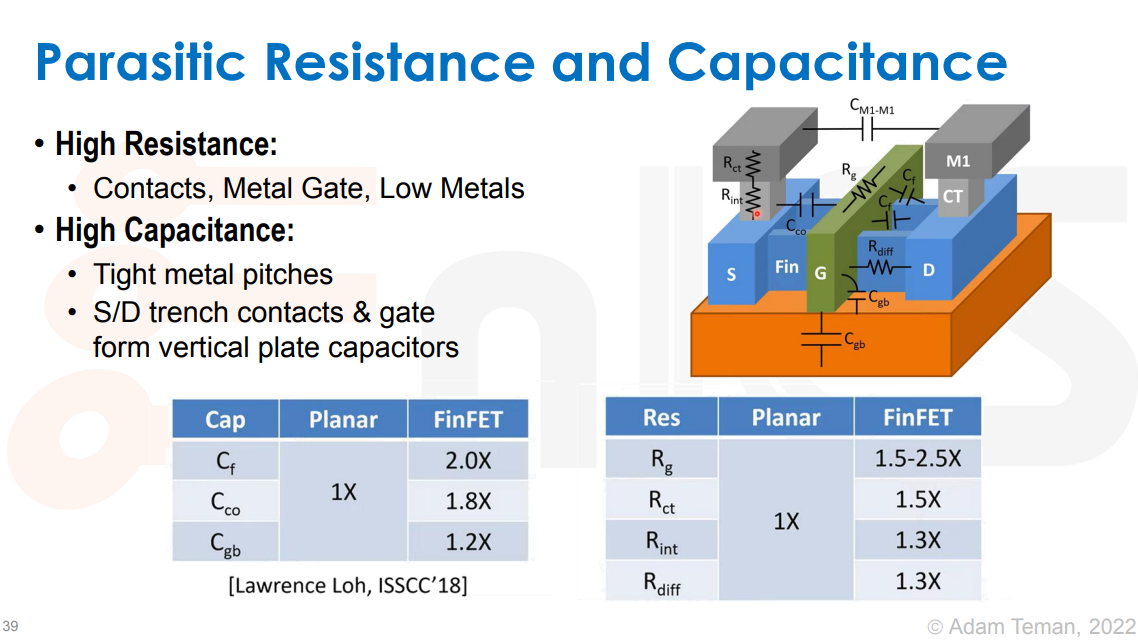
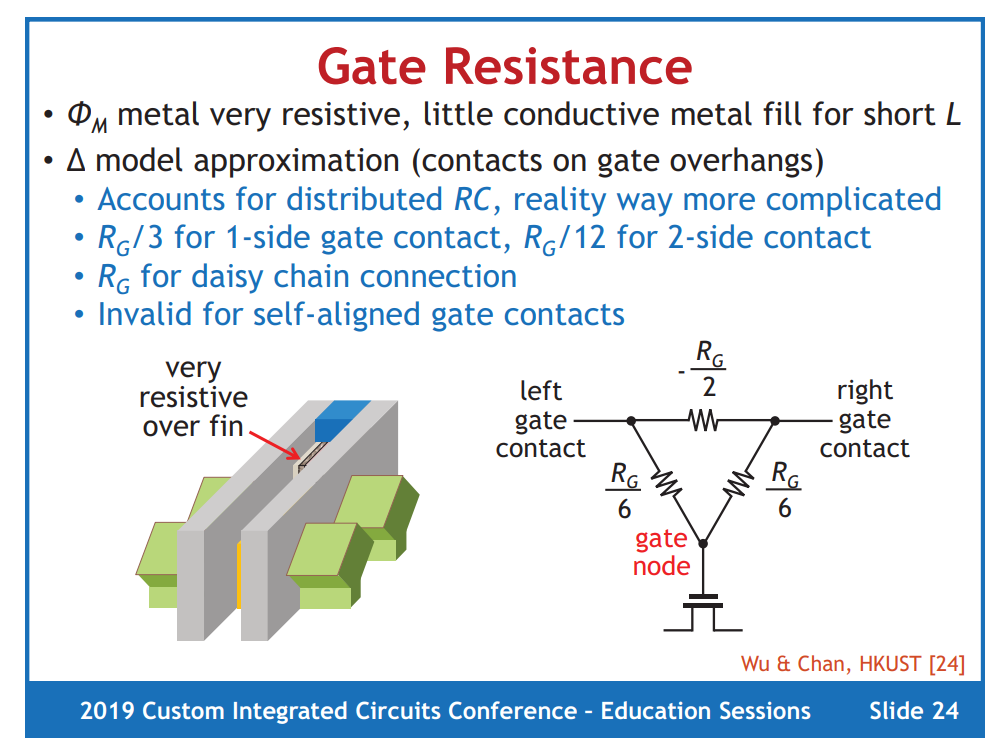
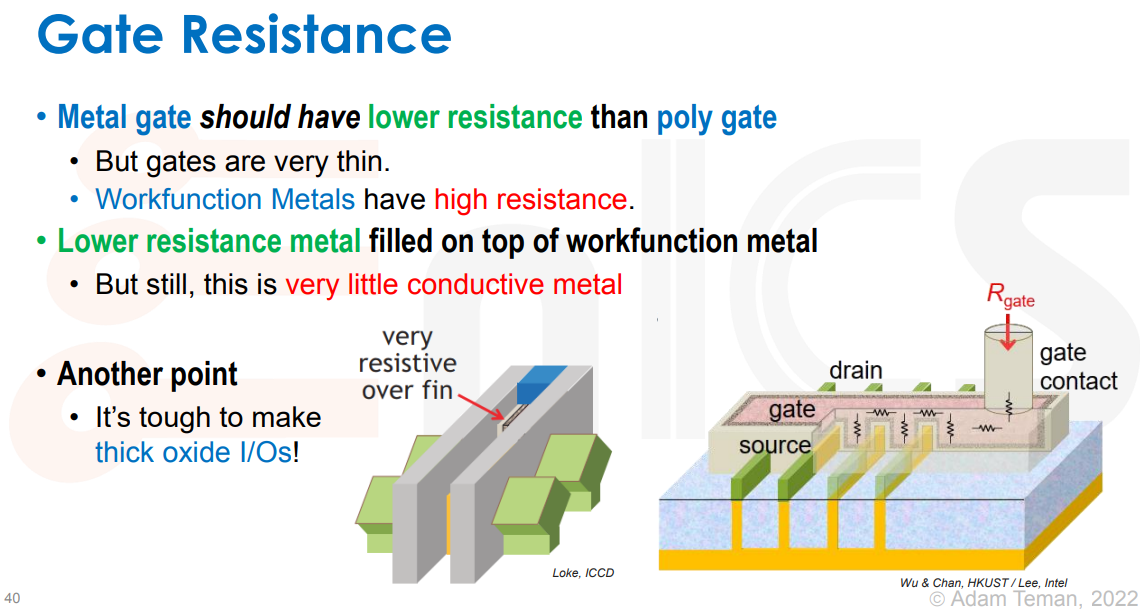
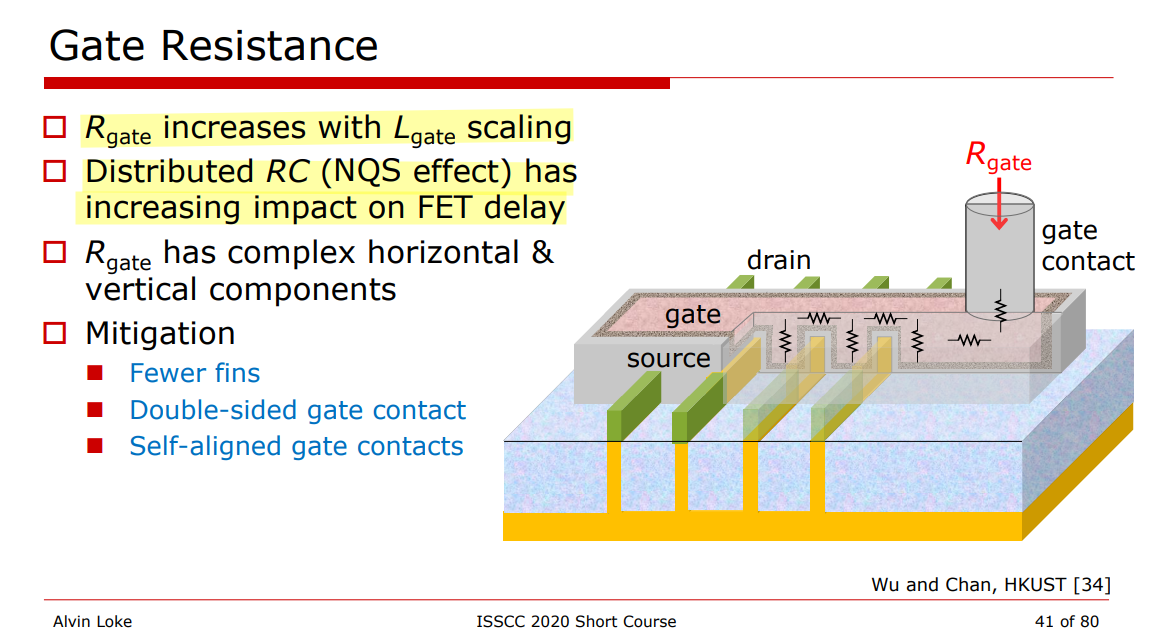
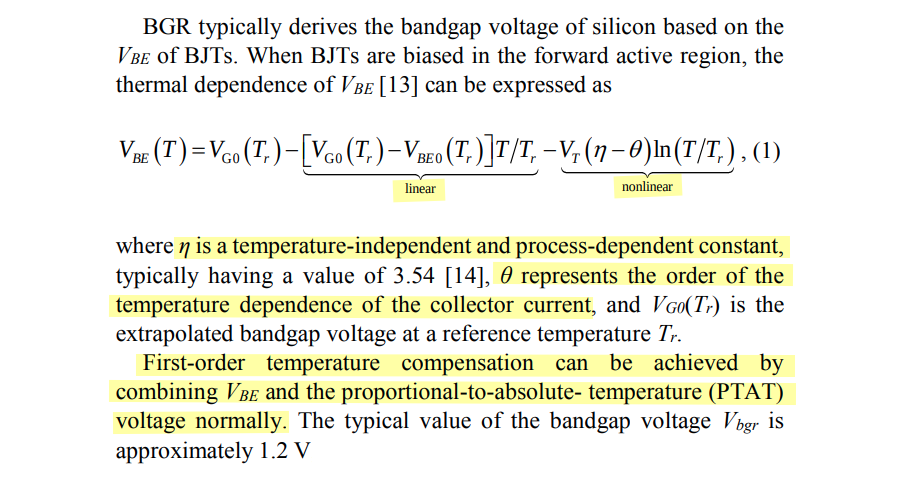
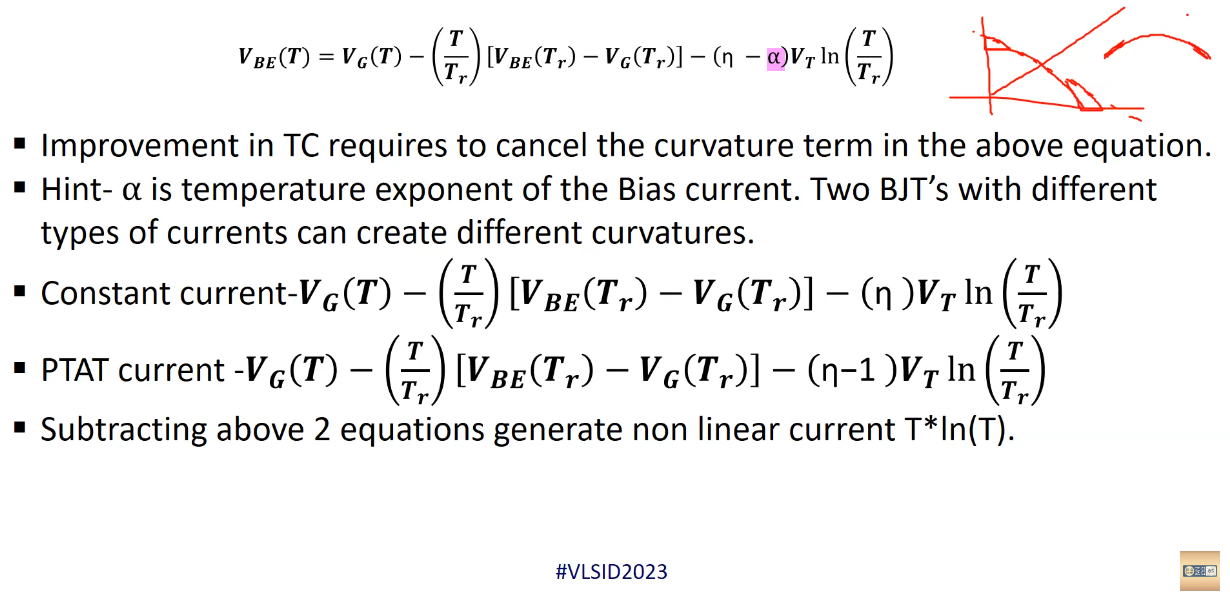
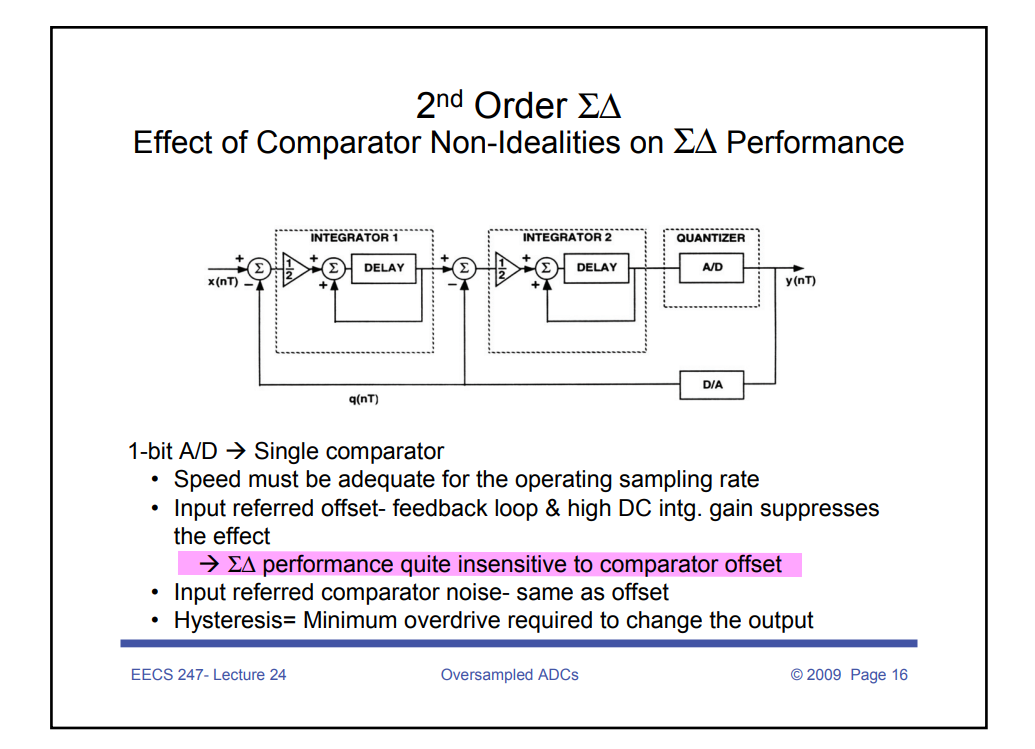
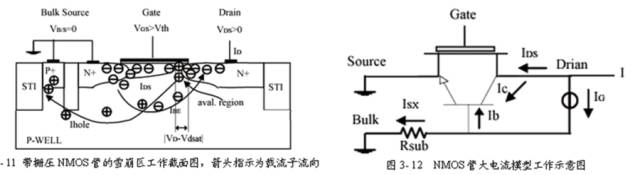
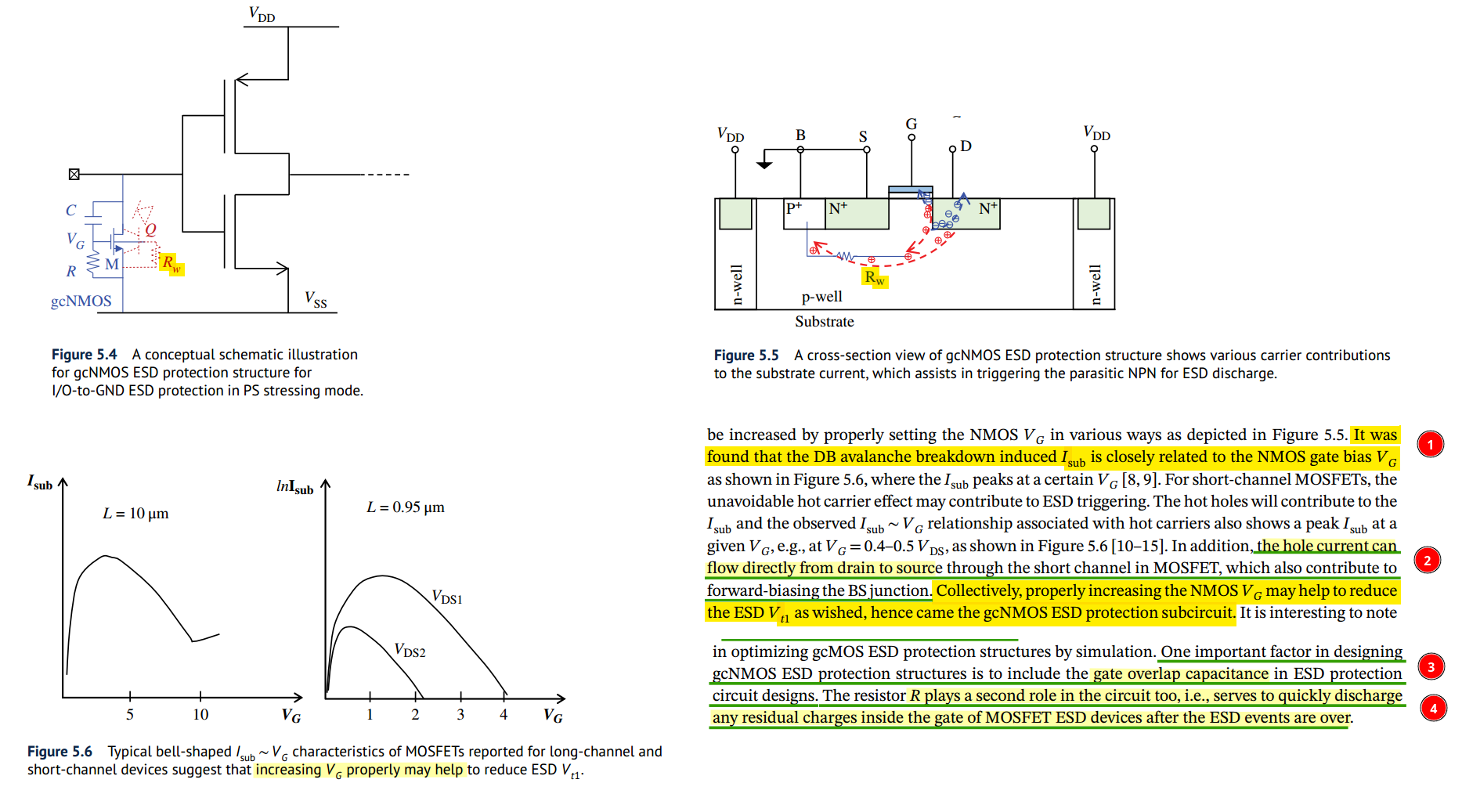
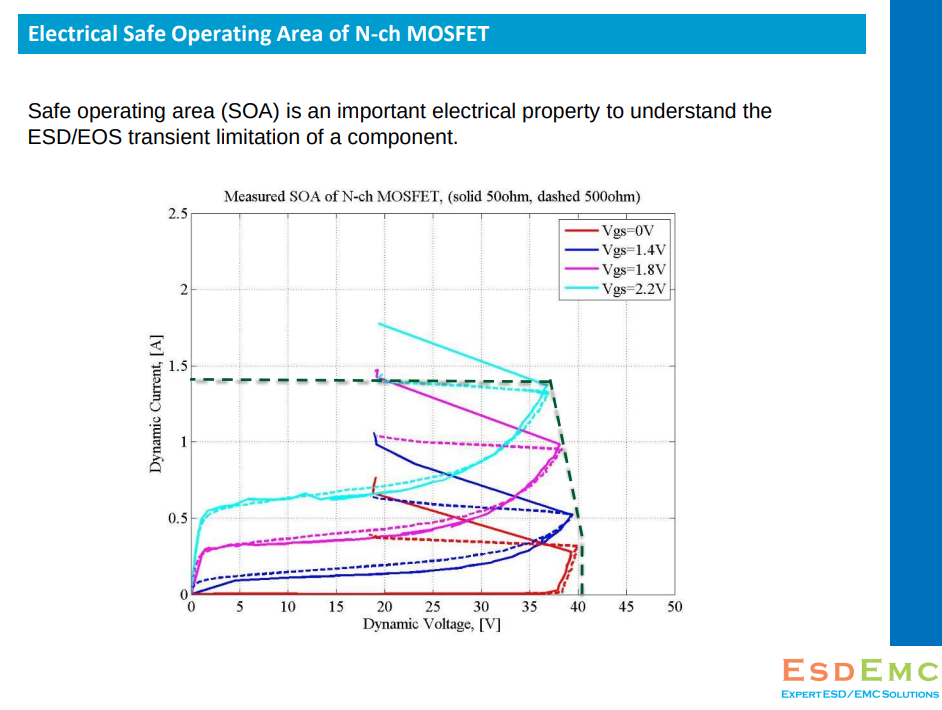
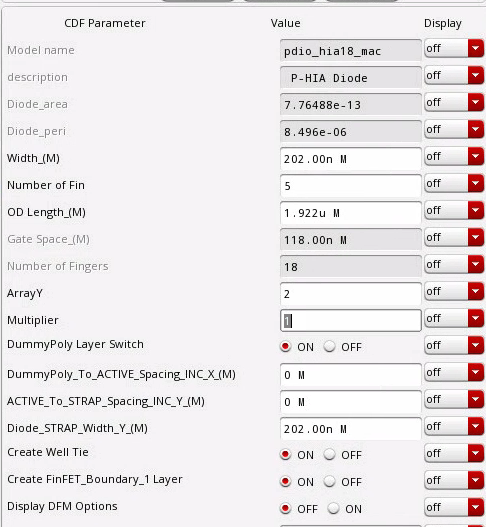
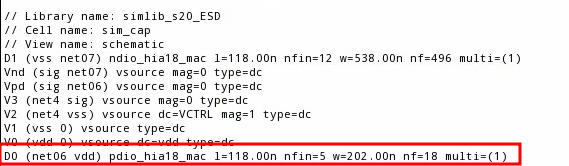
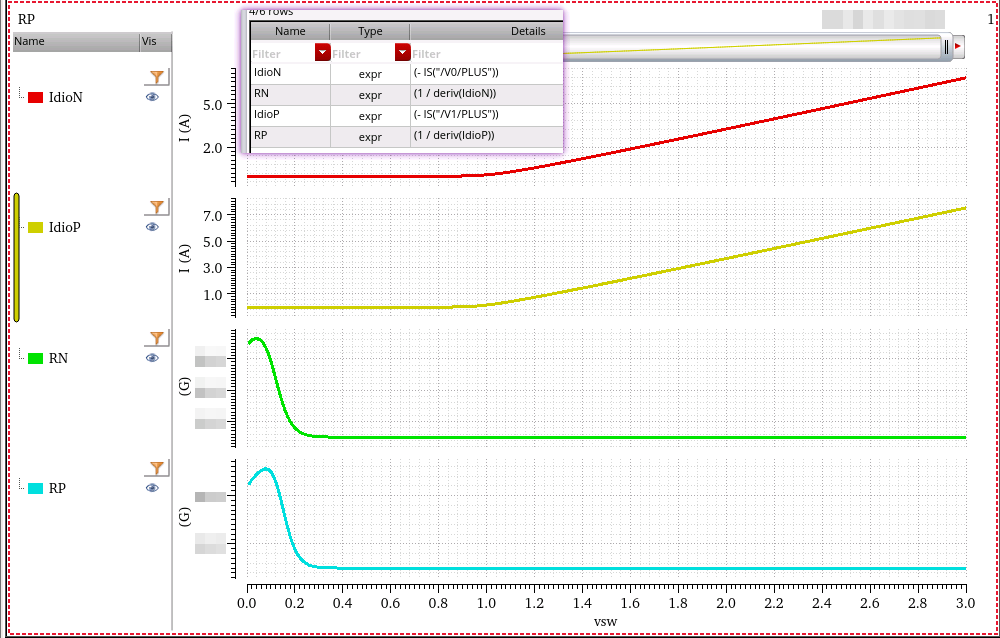
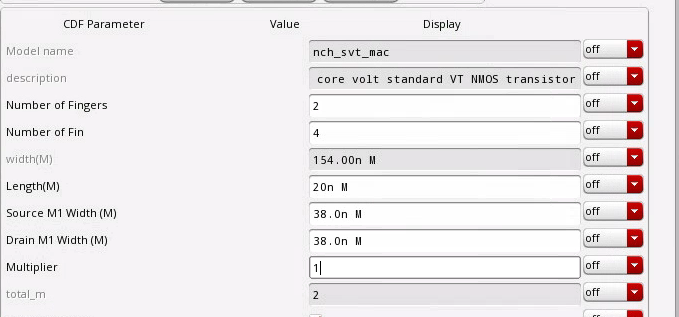
Device Current Components
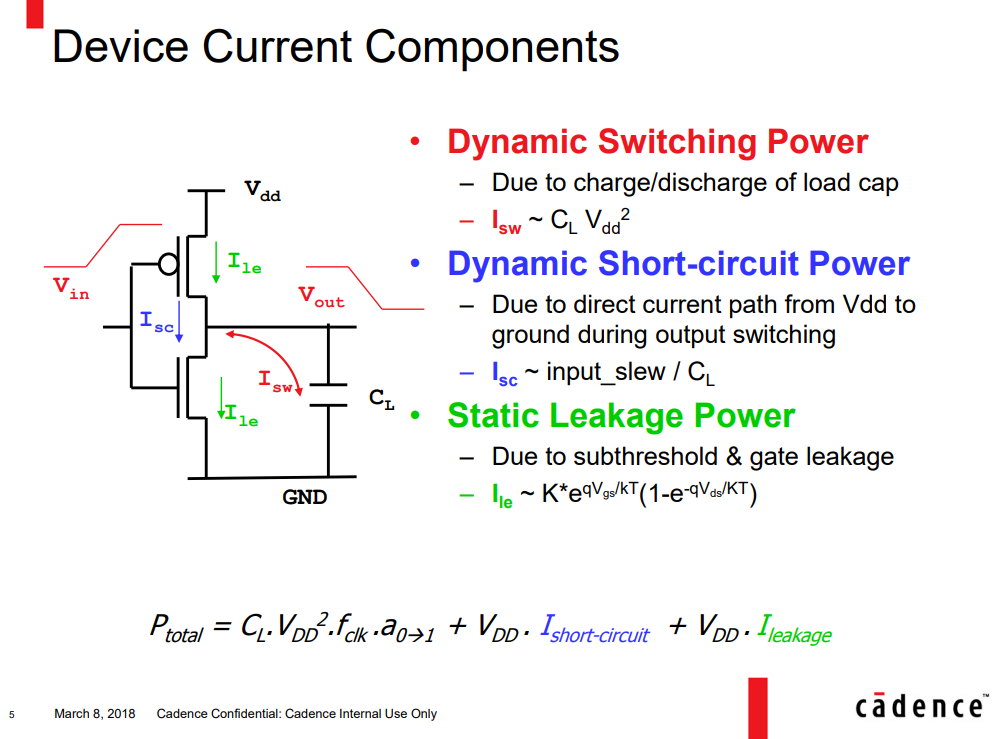
common gate amplifiers

Shot Noise
Any dc current flowing through a diode generates the so-called "shot noise" due to the random nature of the hole and electron transitions across the pn junction
Shot noise is not relevant in CMOS devices since it is only present in bipolar transistors and junction diodes
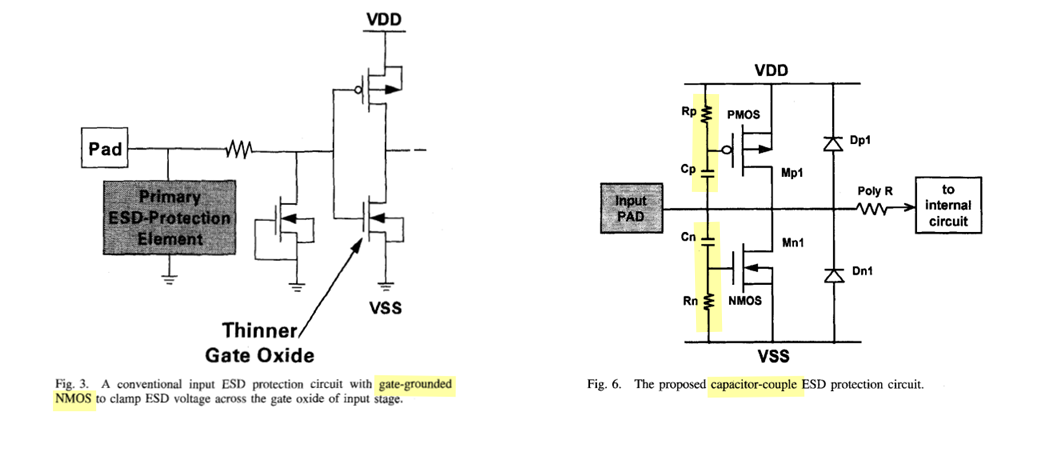
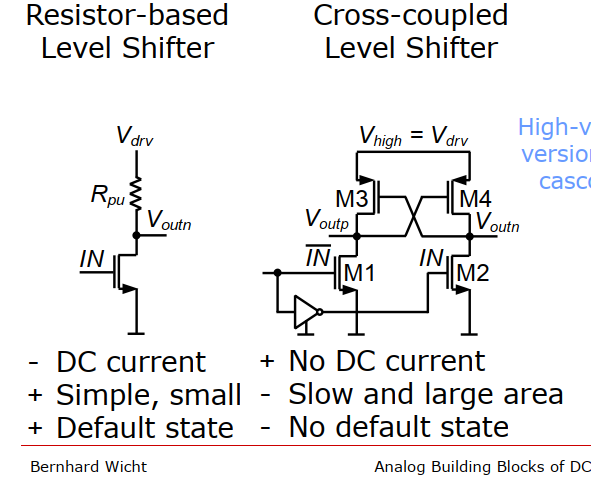
Level Shifter
TIA
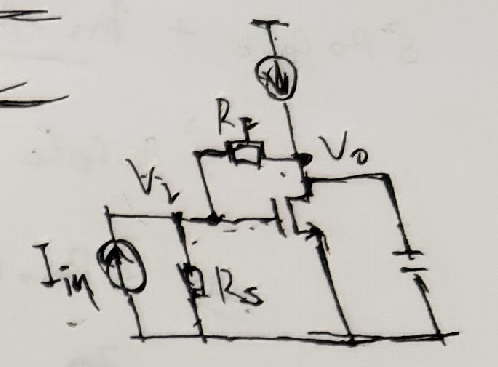
\[\begin{align} I_{in} &= \frac{V_i}{R_S} + \frac{V_i - V_o}{R_F} \\ \frac{V_i - V_o}{R_F} &= g_m V_i \end{align}\]
Then
\[\begin{align} V_o &= \frac{I_{in}R_F}{\frac{R_S+R_F}{R_S}\frac{1}{1-g_mR_F}- 1} \\ V_i &= \frac{I_{in}R_F}{\frac{R_F}{R_S}+g_mR_F} \end{align}\] If \(R_S \gg R_F\) \[\begin{align} V_o &= \frac{I_{in}}{g_m}(1-g_mR_F) \\ V_i &= \frac{I_{in}}{g_m} \end{align}\]
linearity
TIA stage allows for improved gain with better linearity, as mostly signal current passes through \(R_F\) TODO 📅 ??? Quantitative analysis
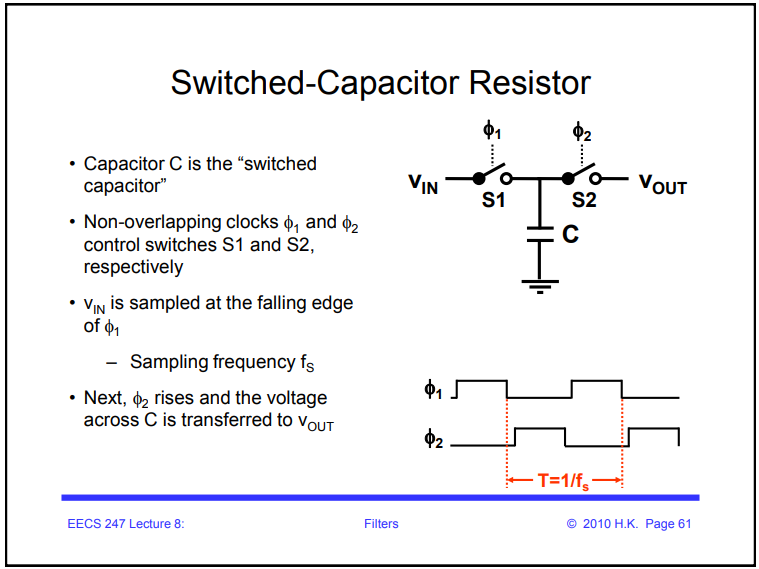
Switched-Capacitor Resistor
\[ R_{eq} = \frac{1}{f_sC} \]
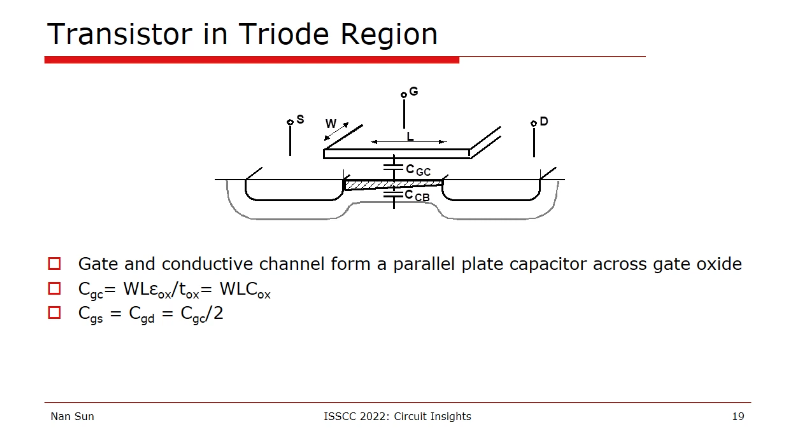
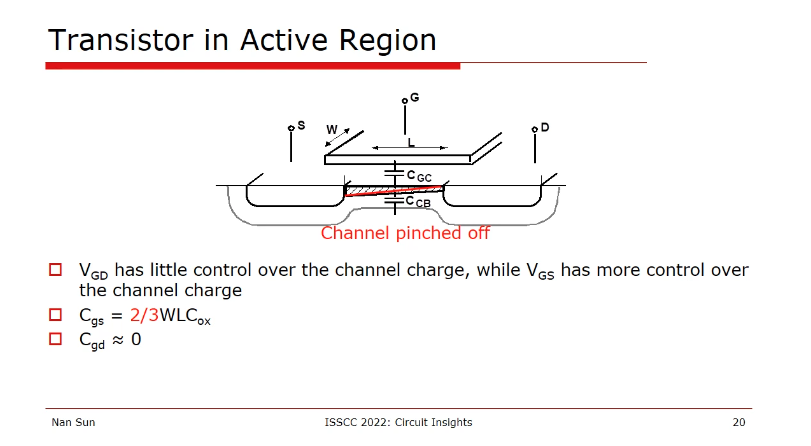
Channel-Length Modulation & Pinched off
- \(\lambda \propto \frac{1}{L_g}\)
- \(\lambda \propto \frac{1}{V_{DS}}\)
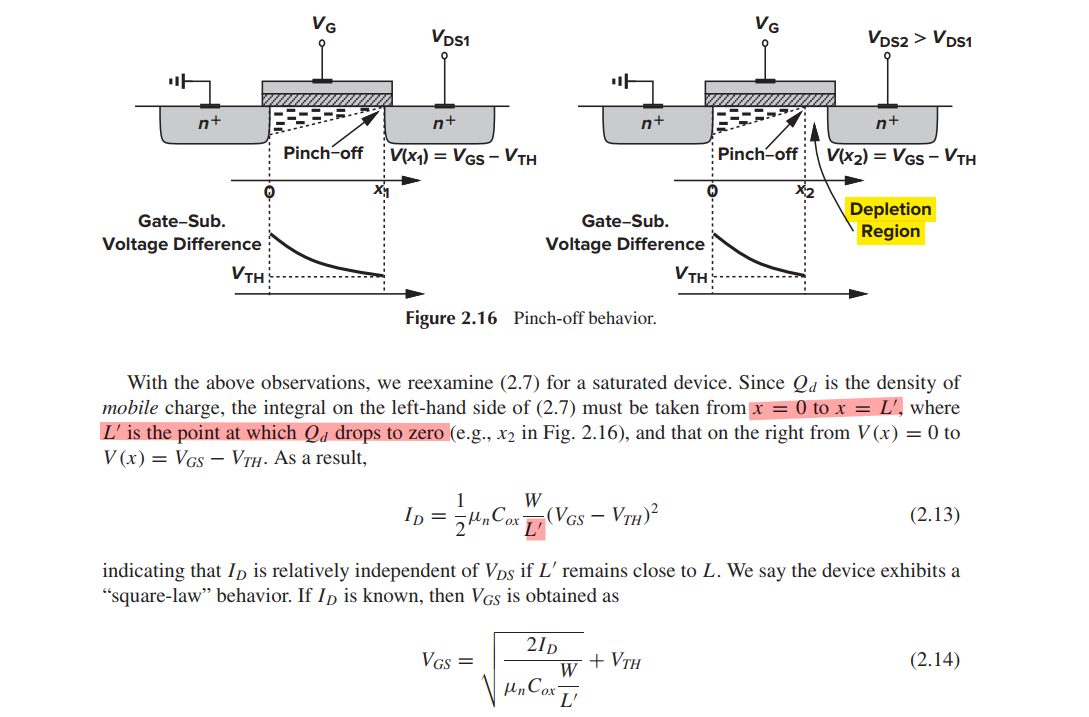
- If \(V_{DS}\) is slightly greater than \(V_{GS} - V_{TH}\), then the inversion layer stops at \(x \leq L\), and we say the channel is "pinched off"
- Upon passing the pinchoff point, the electrons simply shoot through the depletion region near the drain junction and arrive at the drain terminal
\(L^{'}\) is the function of \(V_{DS}\)
with \(\frac{1}{L^{'}} = \frac{1}{L-\Delta L}=\frac{L+\Delta L}{L^2-\Delta L^2}\approx \frac{1}{L}\left(1+\frac{\Delta L}{L}\right)\), we have \[ I_D \approx \frac{1}{2}\mu_n C_{ox}\frac{W}{L}\left(1+\frac{\Delta L}{L}\right)(V_{GS}-V_{TH})^2 = \frac{1}{2}\mu_n C_{ox}\frac{W}{L}(V_{GS}-V_{TH})^2 (1+\lambda V_{DS}) \] assuming \(\frac{\Delta L}{L} = \lambda V_{DS}\)
\(\lambda\) represents the relative variation in length for a given increment in \(V_{DS}\). Thus, for longer channels, \(\lambda\) is smaller
In reality, however, \(r_O\) varies with \(V_{DS}\). As \(V_{DS}\) increases and the pinch-off point moves toward the source, the rate at which the depletion region around the source becomes wider decreases, resulting in a higher incremental output impedance.
Early Voltage indicator
\[ g_m r_o = \frac{g_m}{I_D}I_D \cdot \frac{V_A}{I_D} = \frac{g_m}{I_D} \cdot V_A \]
$g_m r_o $ is the indicator of \(V_A\), if \(\frac{g_m}{I_D}\) is same
Resonator
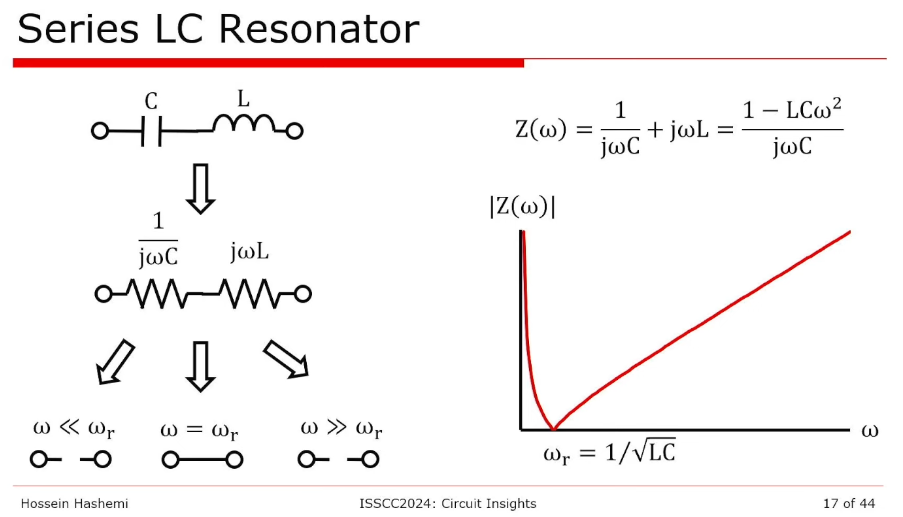
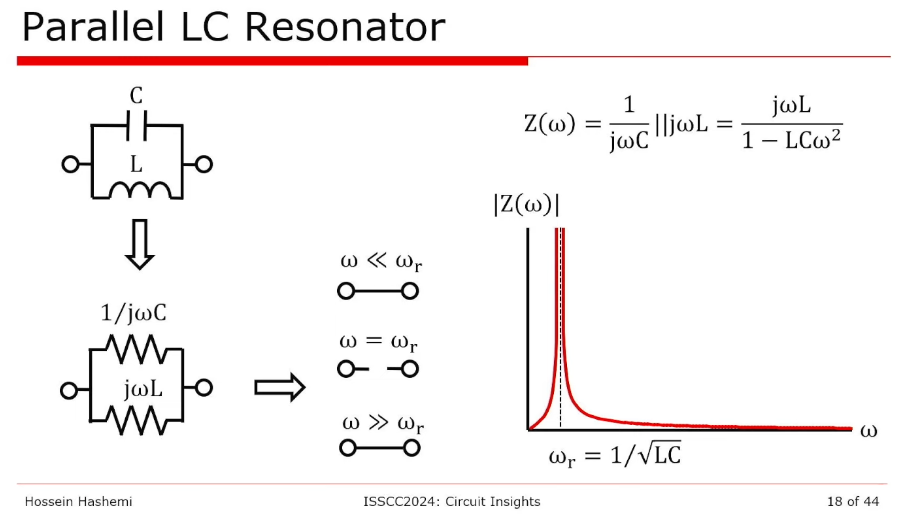
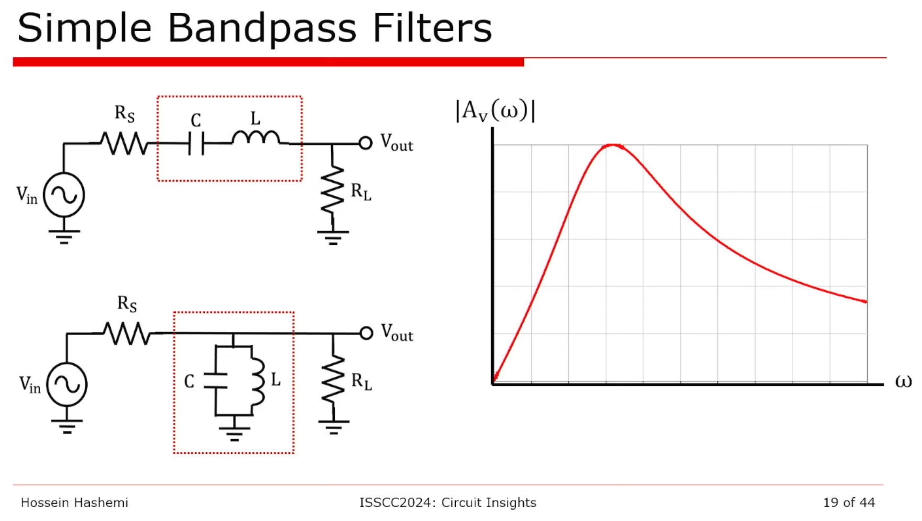
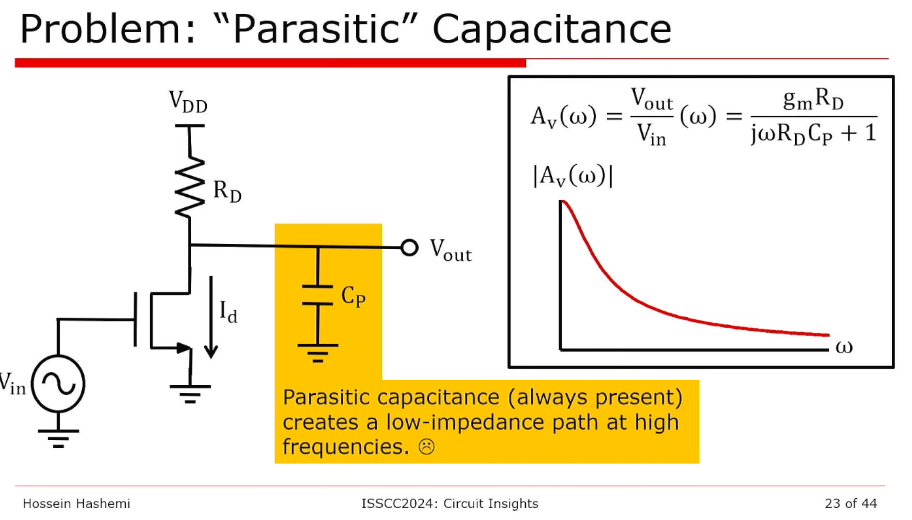
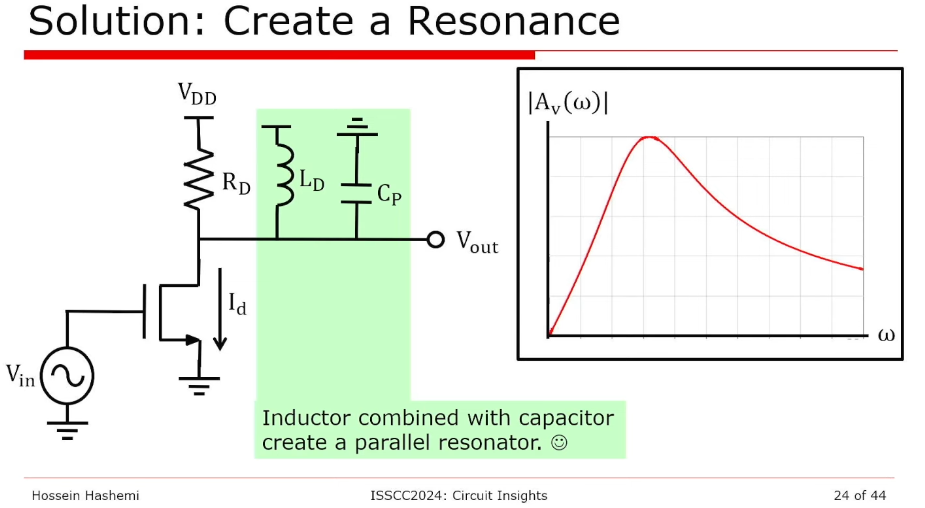
bandpass filter
Hossein Hashemi, RF Circuits, [https://youtu.be/0f3yZMvD2Jg?si=2c1Q4y6WJq8Jj8oN]
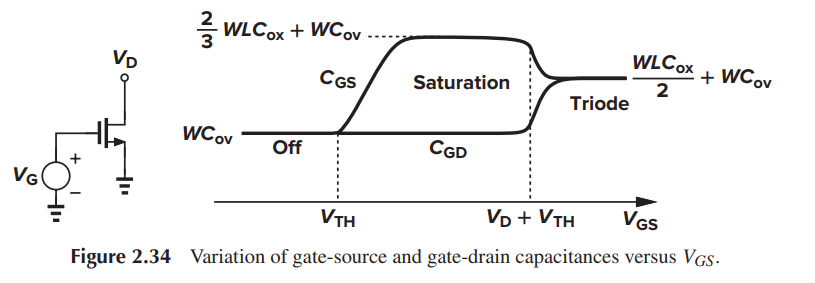
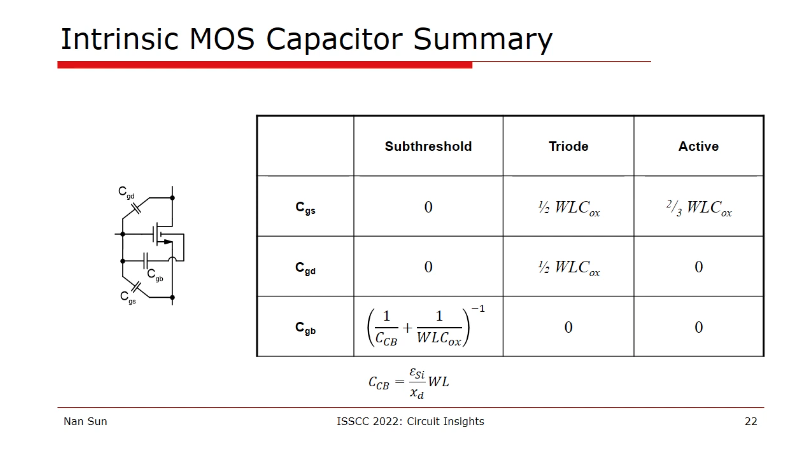
Cgd of Common-Source Stage
Miller effect of Cgd during layout
Nonlinearity of Differential Circuits
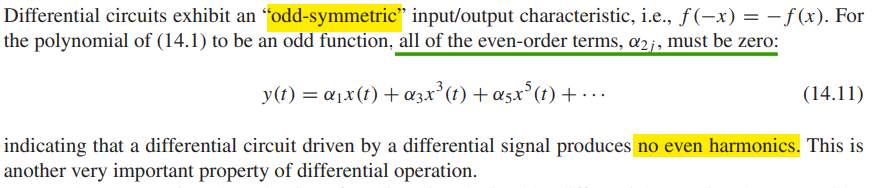
\[ \cos^3\omega t = \frac{3\cos \omega t + \cos(3\omega t)}{4} \]
Zero in differential pair with active current mirror
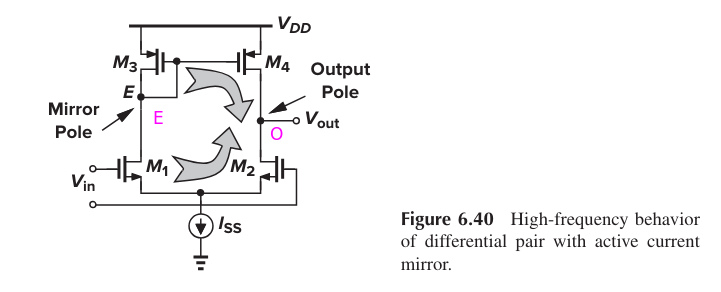
Noting the circuit consists of a "slow path" (M1, M3, M4) in parallel with a "fast path" (M2)
"slow path" \[ H_\text{slow}(s) = \frac{A_0}{(1+s/\omega _{pE})(1+s/\omega _{pO})} \]
"fast path" \[ H_\text{fast}(s) = \frac{A_0}{1+s/\omega _{pO}} \]
Then \[\begin{align} \frac{V_\text{out}}{V_\text{in}} &= H_\text{slow}(s) + H_\text{fast}(s) \\ &= \frac{A_0}{1+s/\omega _{pO}}\left(\frac{1}{1+s/\omega _{pE}} + 1 \right) \\ &= \frac{A_0(1+s/2\omega _{pE})}{(1+s/\omega _{pO})(1+s/\omega _{pE})} \end{align}\]
That is, the system exhibits a zero at \(2\omega_{pE}\)
signals traveling through two paths within an amplifier may cancel each other at one frequency, creating a zero in the transfer function
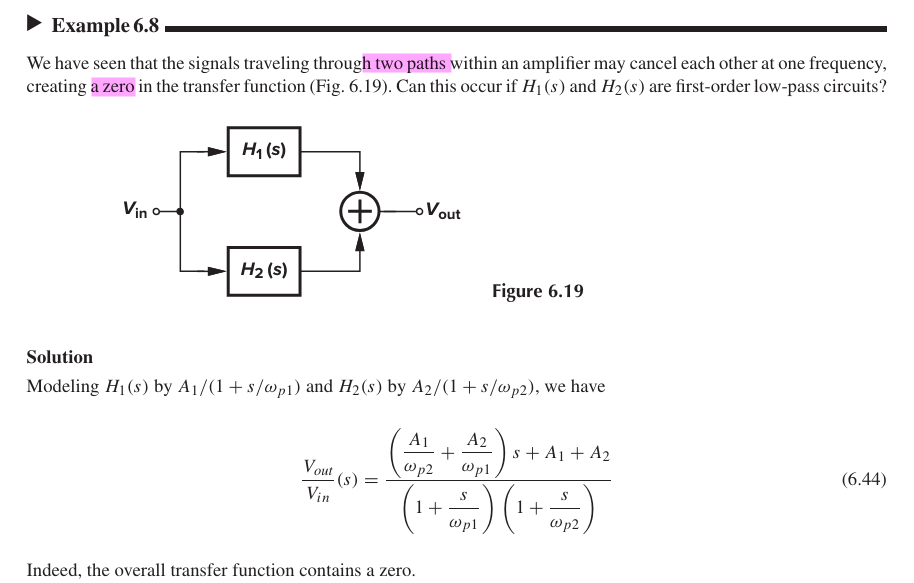
\[ \omega_z = \frac{(A_1+A_2)\omega_{p1}\omega_{p2}}{A_1\omega_{p1}+A_2\omega_{p2}} \] noting \(\omega_{p1}\lt \omega_z \lt \omega_{p2}\)
"Zero" by Inspection
a method to predict the existence of "zero" by inspection, based on the concept of "Analog Phase Interpolation"
TODO 📅
Debashis Dhar, How to Recognize "Zero" by Inspection (Utilizing Analog Phase Interpolation) [https://www.linkedin.com/posts/debashis-dhar-12487024_how-to-recognize-zero-by-inspection-activity-7163364364329160704-9qOq?utm_source=share&utm_medium=member_desktop]
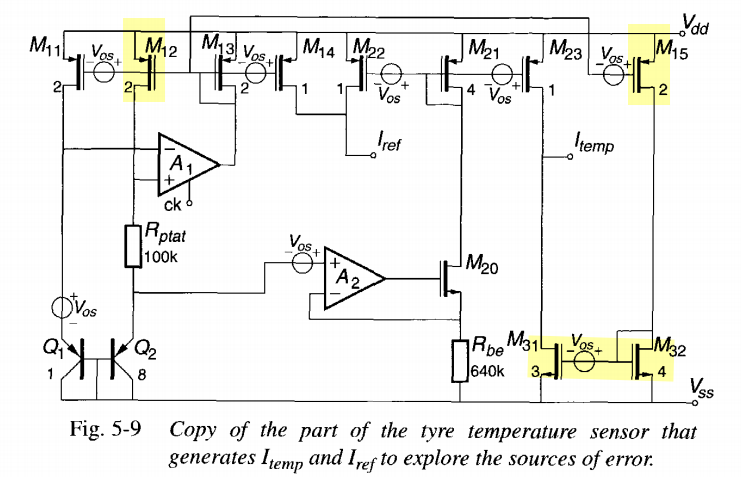
Random offset
The dependence of offset voltage and current mismatches upon the overdrive voltage is similar to our observations for corresponding noise quantities
differential pair
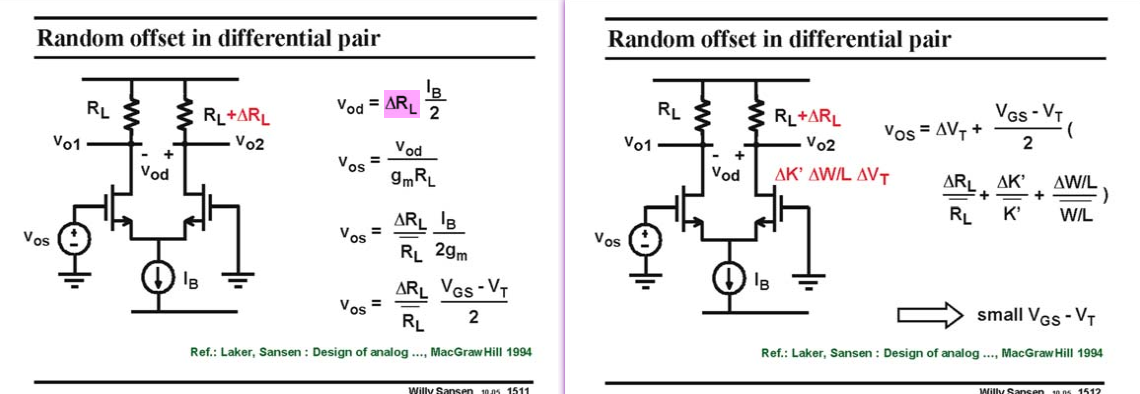
In reality, since mismatches are independent statistical variables

Above shows that the input transistors must be designed for high gain (\(g_mr_o = \frac{2}{V_{OV}\lambda}\)), which means they must be designed for small \(V_{GS}-V_{TH}\).
It is desirable to minimize \(V_{GS}-V_{TH}\) by lowering the tail current or increasing the transistor widths
For \(\frac{\Delta K}{K}\)
\[\begin{align} v_{os} g_m &= \Delta K \frac{W}{L}(V_{GS}-V_{TH})^2 \\ v_{os} 2K\frac{W}{L}(V_{GS}-V_{TH}) &= \Delta K \frac{W}{L}(V_{GS}-V_{TH})^2 \\ v_{os} &= \frac{V_{GS}-V_{TH}}{2} \frac{\Delta K}{K} \end{align}\]
The derivation for \(\frac{\Delta W/L}{W/L}\) is same with \(\frac{\Delta K}{K}\)
alternative derivation
\[\begin{align} \Delta V_\beta \cdot g_m &= \frac{\partial I_D}{\partial \beta} \Delta \beta \\ &= I_D \frac{\Delta \beta}{\beta} \end{align}\]
That is \(\Delta V_\beta = \frac{I_D}{g_m}\frac{\Delta \beta}{\beta}\)
\[ \Delta V_R \cdot g_m R = I_D \cdot \Delta R \]
That is \(\Delta V_R = \frac{I_D}{ g_m} \cdot \frac{\Delta R}{R}\)
current mirror
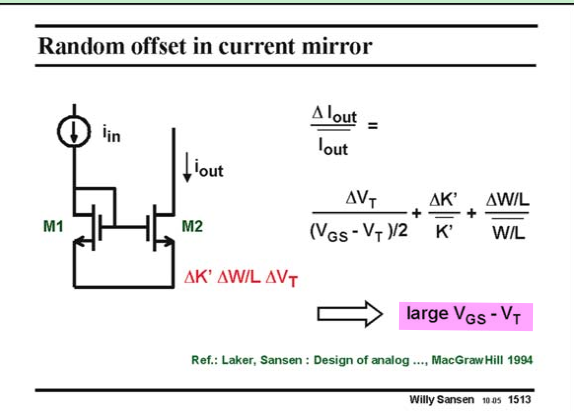
To minimize current mismatch, the overdrive voltage must be maximized, a trend opposite to that in differential pair.
This is because as \(V_{GS}-V_{TH}\) increases, threshold mismatch has a lesser effect on the device currents
\(\Delta I_D= g_m \Delta V_{TH} = \frac{2I_D}{V_{OV}}\Delta V_{TH}\)
Effect of Feedback on Noise
Feedback does not improve the noise performance of circuits.
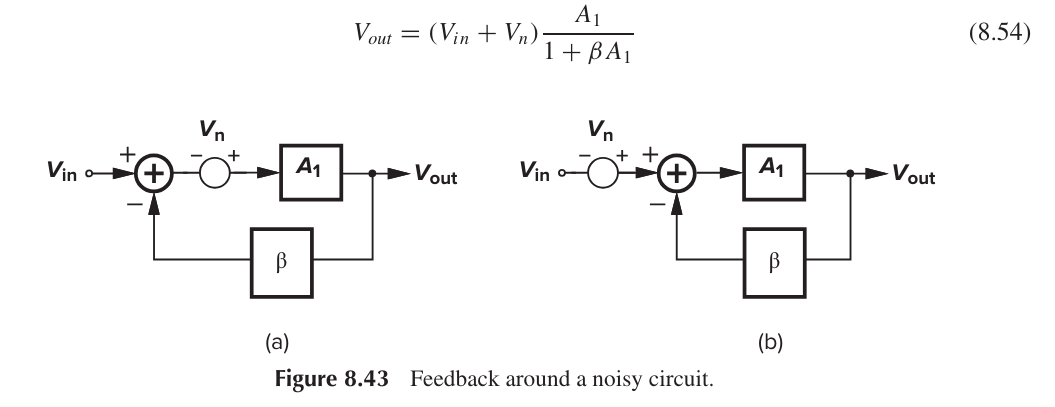
The input-referred noise voltage and current remain the same if the feedback network introduces no noise.
RC charge & discharge
charge: \[ V_o(t) = V_{X}(1-e^{-\frac{t}{\tau}}) + V_{o,0}\cdot e^{\frac{-t}{\tau}} \]
discharge: \[ V_o(t) = V_{o,0}\cdot e^{-\frac{t}{\tau}} + V_{o,\infty}\cdot(1-e^{-\frac{t}{\tau}}) \]
- \(e^{-\frac{t}{\tau}}\) item determine the initial state
- \((1-e^{-\frac{t}{\tau}})\) item determine the final state
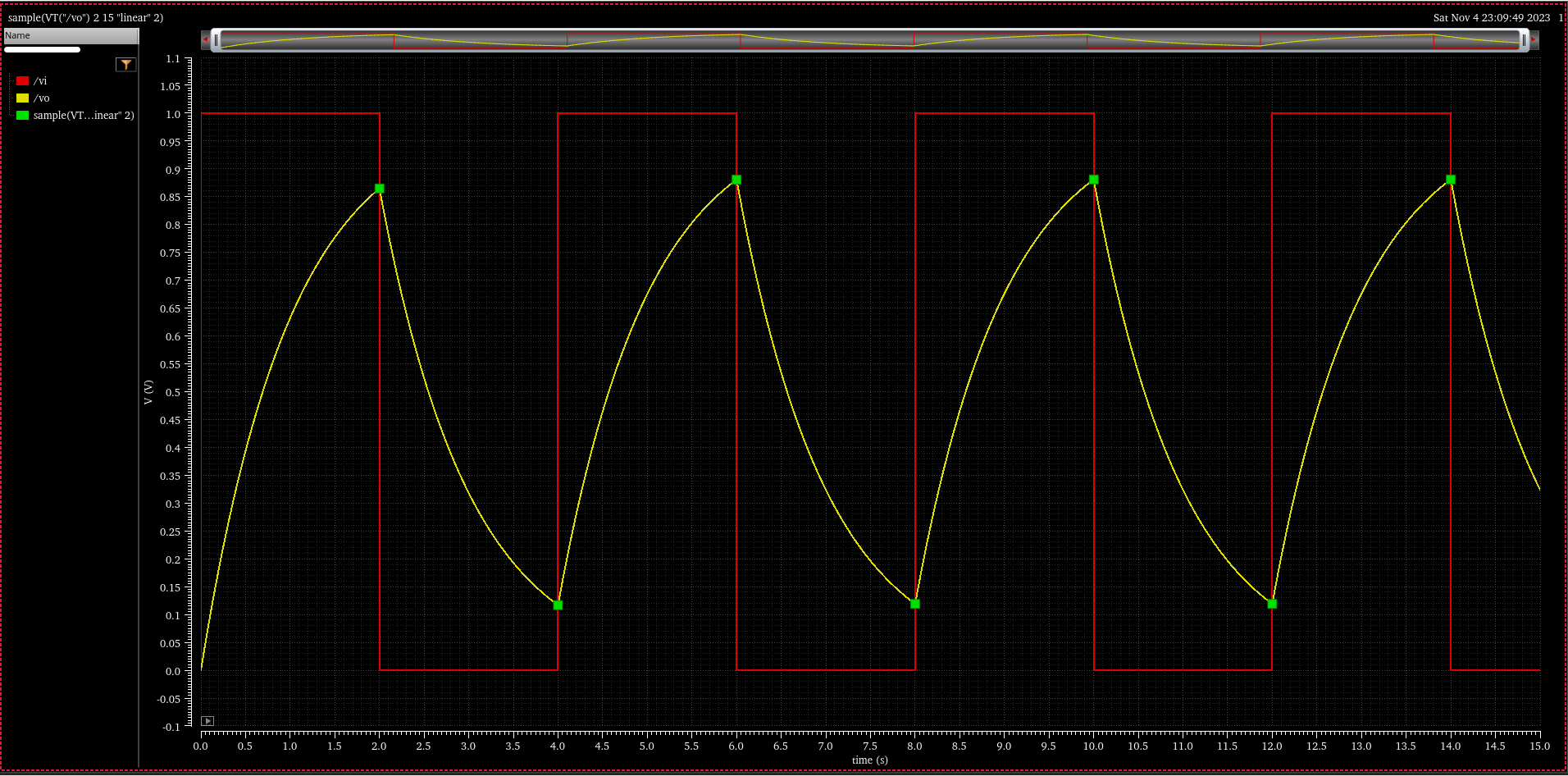
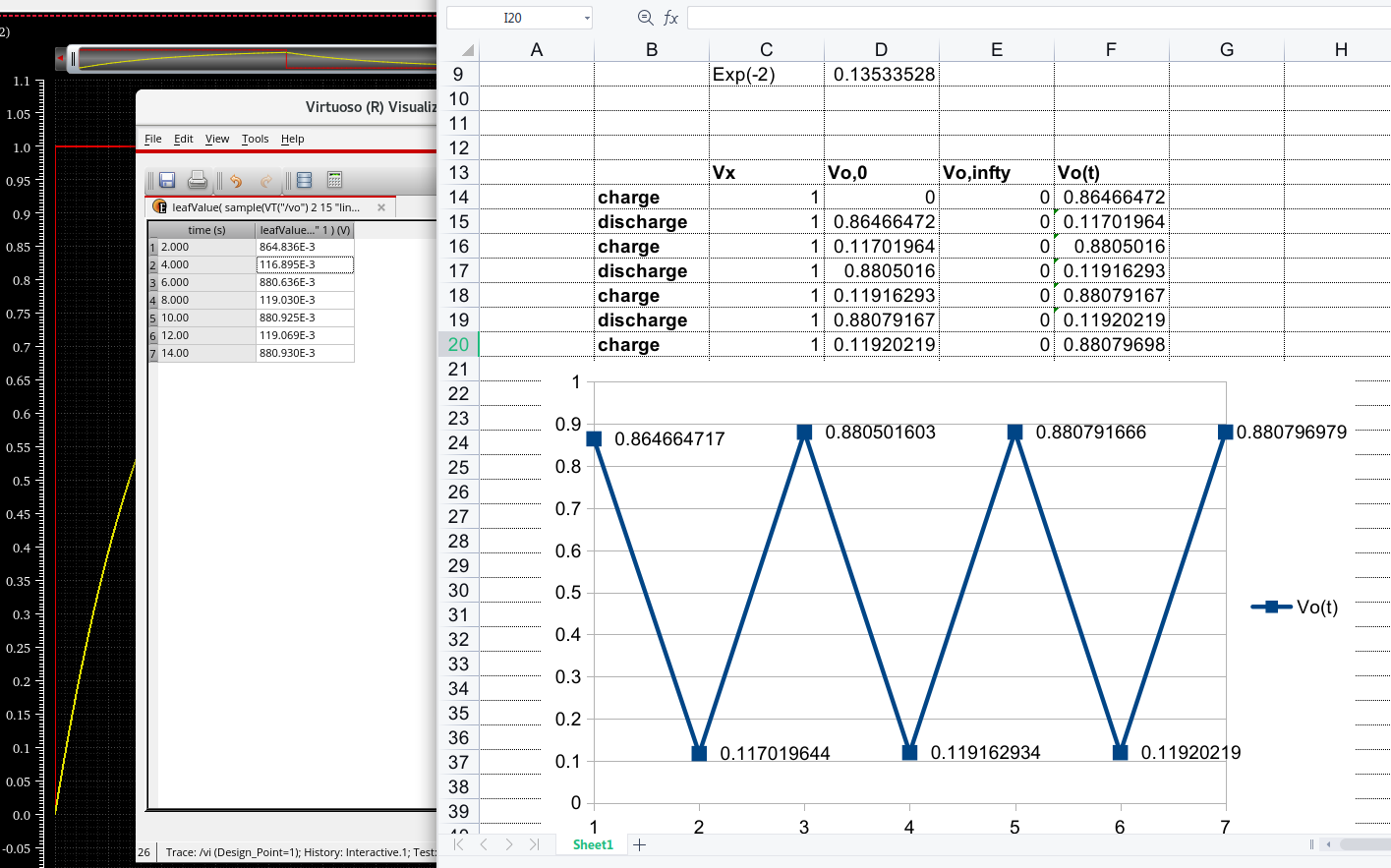
AC coupling
\(V_m=\frac{1}{4},\space \frac{3}{4}\) and its common voltage \(\frac{1}{2}\)
\(V_o=-\frac{1}{4},\space \frac{1}{4}\) and its common voltage \(0\)

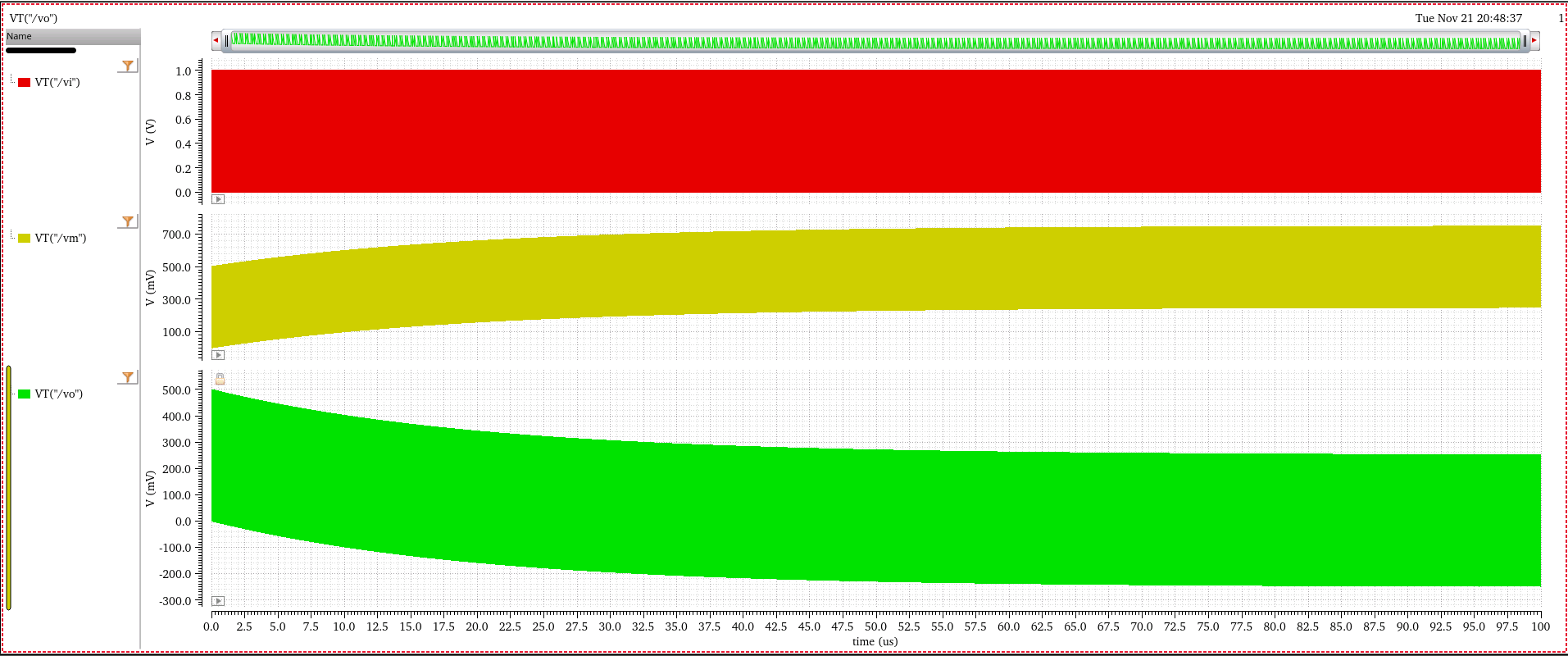
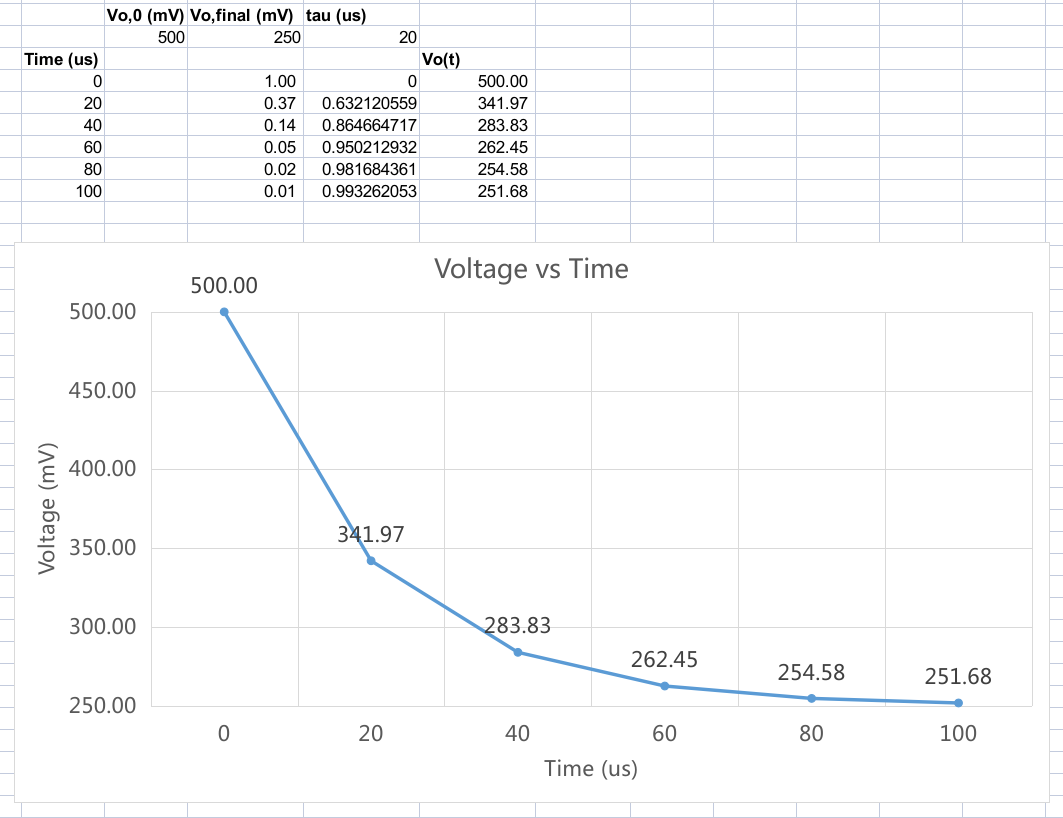
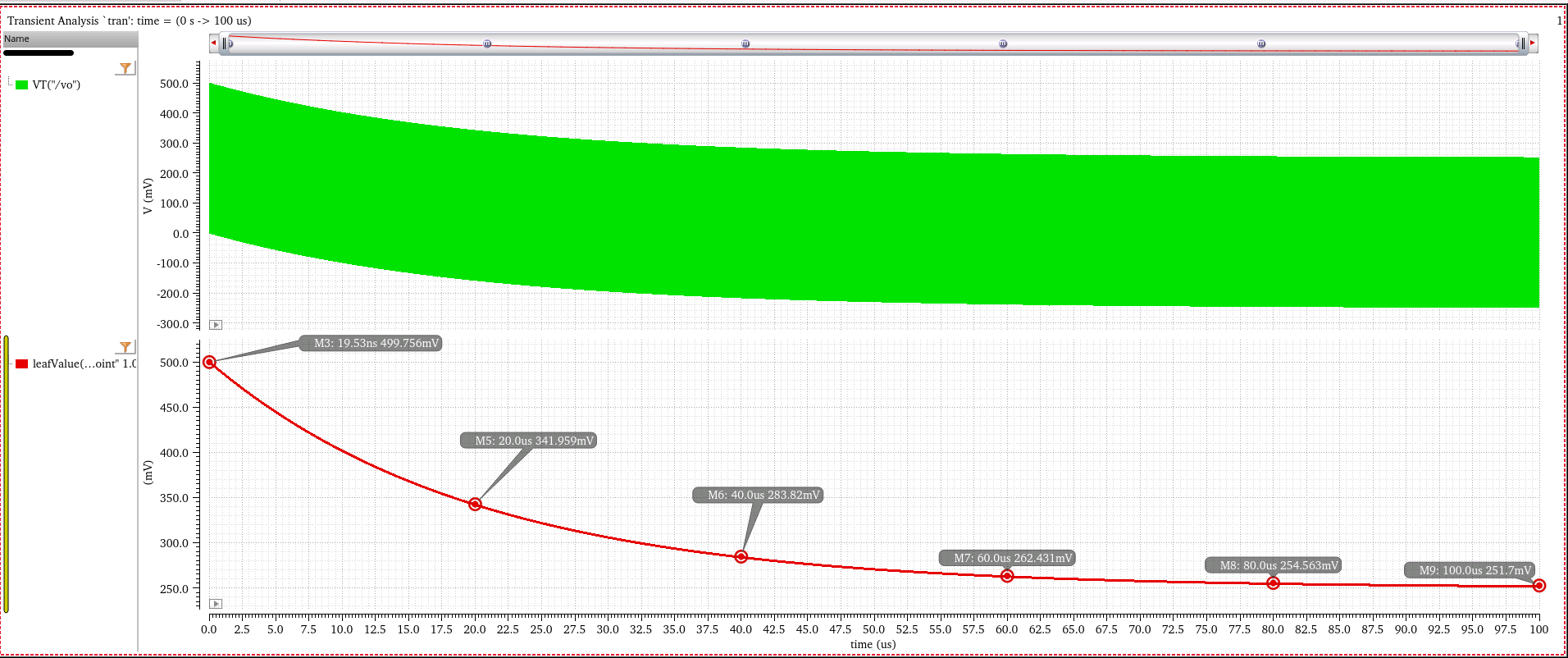
\[ \tau = 200 \text{nF} \times (50+50)\text{ohm} = 20 \mu s \]
high level envelope:
Current mirror with source degeneration
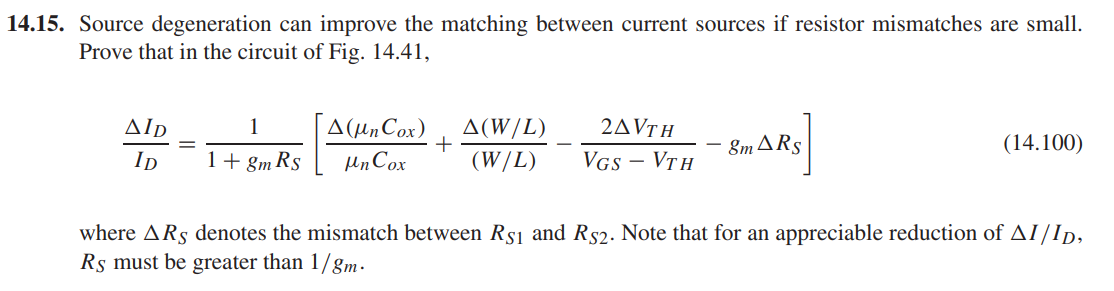
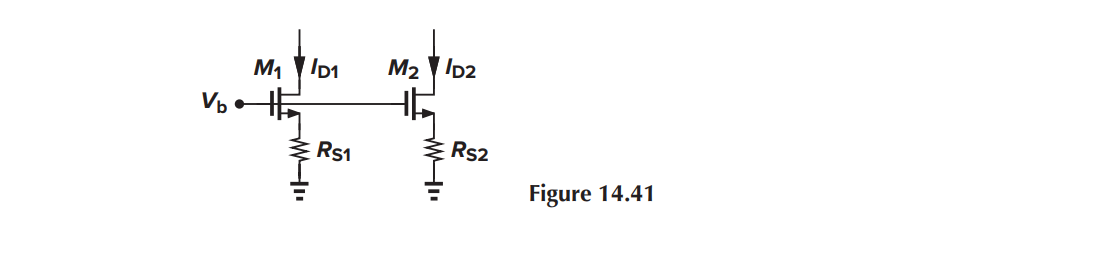

Razavi 2nd, problem 14.15
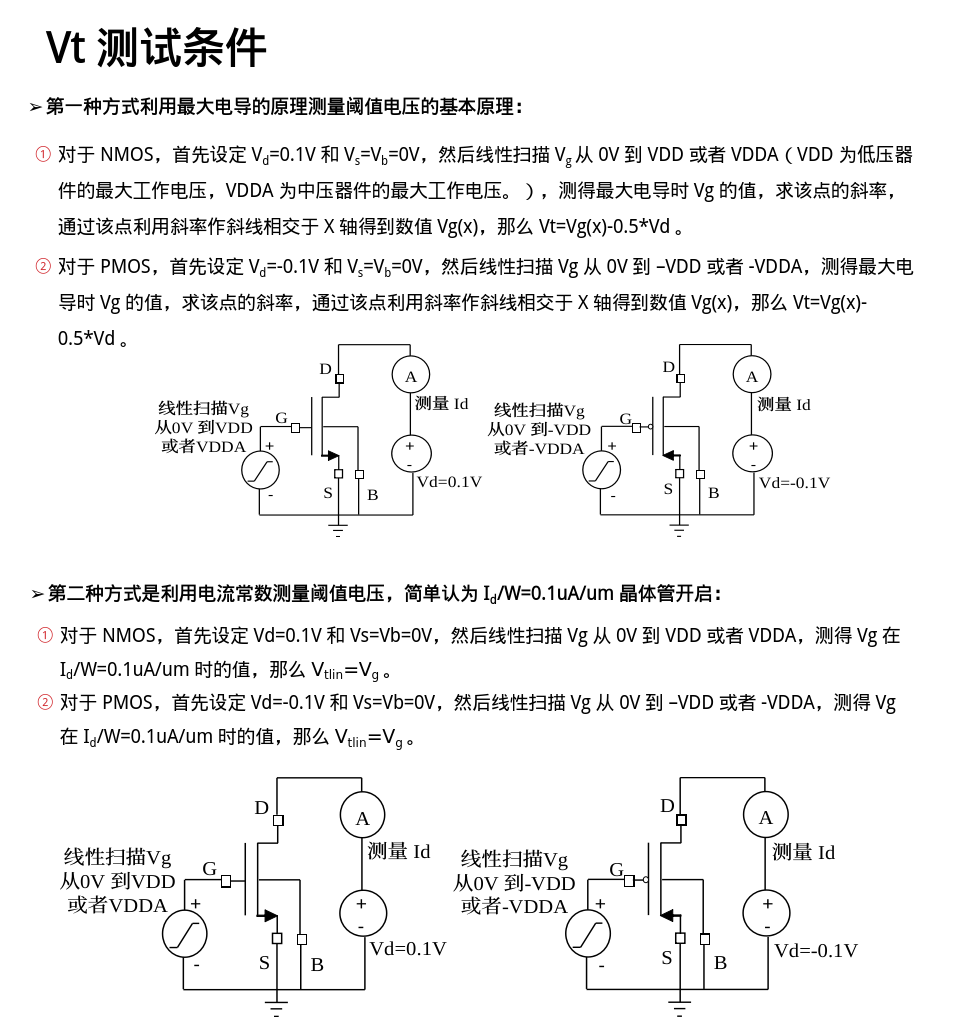
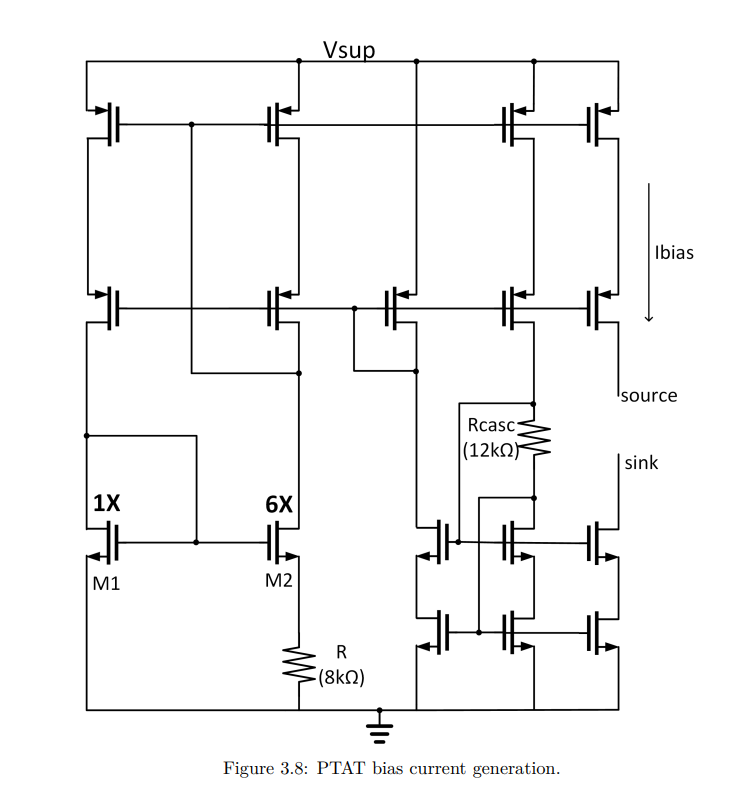
Monitored Analog Critical Parameters
Parameter Definition:
\[\begin{align} I_{\text{D,lin}} &= I_D \mid _{V_G=V_{DD},V_D=0.05V} \\ I_{\text{D,sat}} &= I_D \mid _{V_G=V_D=V_{DD}} \\ V_{\text{t,lin}} &= V_G \mid _{I_D=I_{\text{thx}}\cdot \frac{W}{L}@\{V_D=0.05V\}} \end{align}\]
\(I_{\text{thx}}\) could be different for technologies. (For N16, \(I_{\text{thx}}=10\)nA)
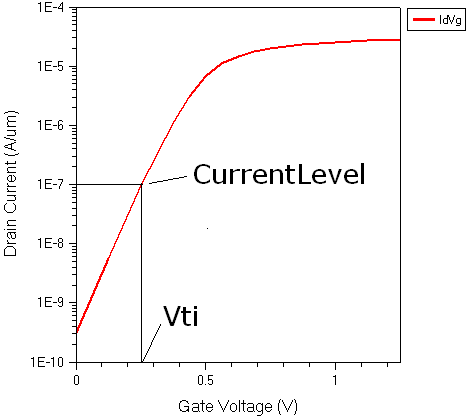
Constant Current Threshold Voltage
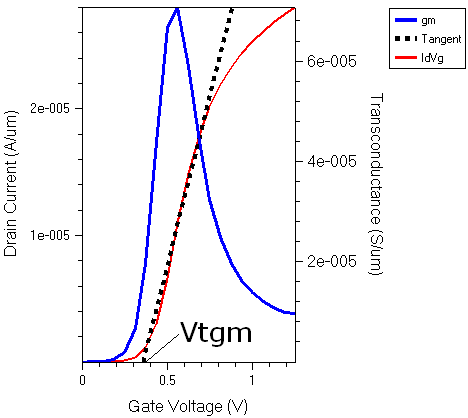
gm-Maximum Method
STB and PSTB in Spectre/RF
All credits to my colleague, Zhang Wenpian. > F. Wiedmann, "Loop gain simulation," Online:[https://sites.google.com/site/frankwiedmann/loopgain]
STB analysis
Spectre stb's "loopgain" is negative of "T" in paper[1] \[ T = \frac{2(AD-BC) - A + D}{2(AD-BC)-A+D-1} \]
AC simulation testbench, shown as below,
\(I_{inj}\) = 0, \(V_{inj}\) = 1
B = if, D = ve
\(I_{inj}\) = 1, \(V_{inj}\) = 0
A = if, C = ve
PSTB analysis
Spectre pstb is similar to stb, just set pac as 1 instead of ac in current source and voltage source.
This analysis just use harmonic 0 transfer function in pac analysis, which has limitation.
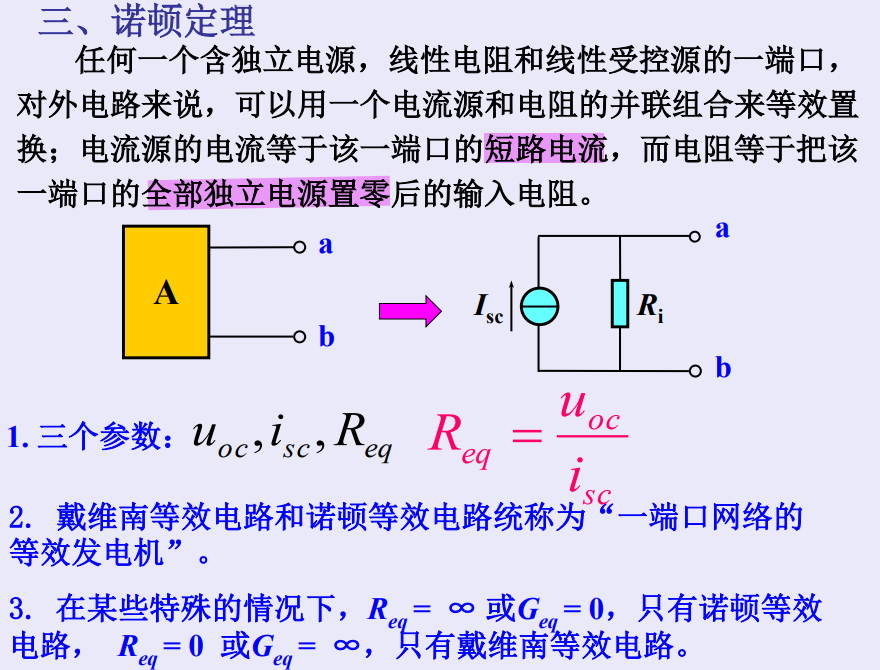
Thevenin and Norton Equivalent Circuits
戴维南定理

等效电阻的计算方法
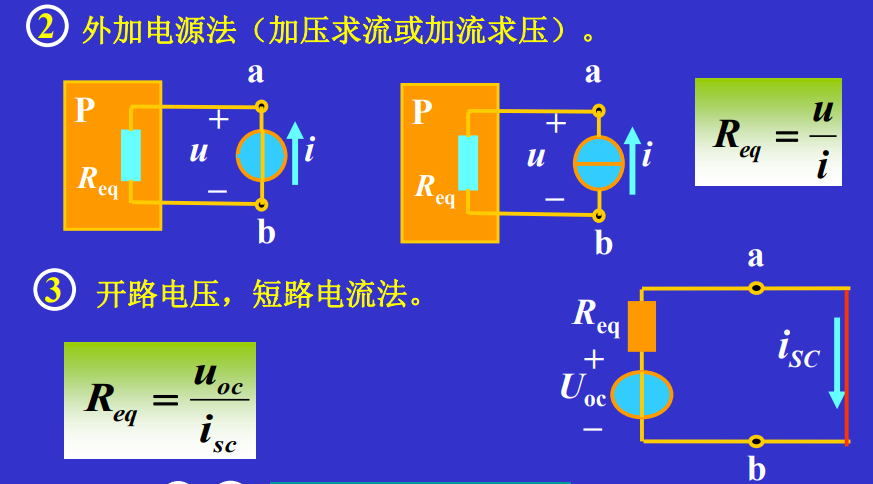
使用外加电源法时, 全部独立电源需要置零
诺顿定理
Lemma of Razavi
\[ A_V = -G_m R_{out} \]
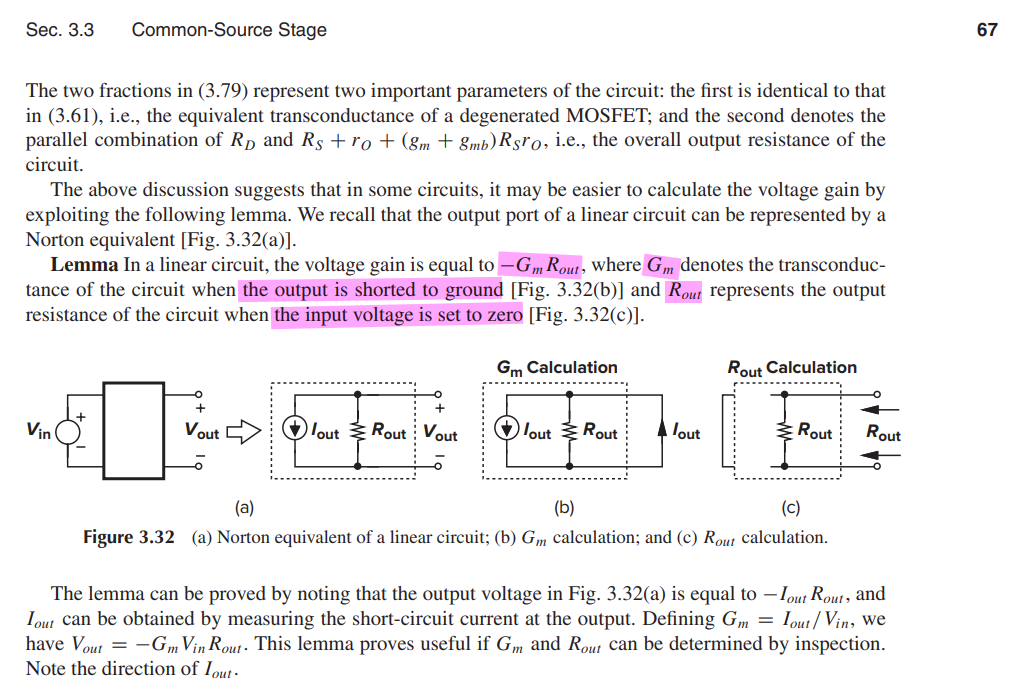
Design of Analog CMOS Integrated Circuits, Second Edition - Behzad Razavi
Miller's Approximation: right-half-plane zero

A quick inspection of this circuit reveals that a zero lies at a frequency where the current through \(C_{12}\) becomes equal to \(g_2V_1\).
When this occurs, the current through the parallel combination of \(C_2\) and \(R_2\) becomes zero, creating a zero in the transfer function.
In other words, we can write
\[\begin{align} g_2V_1 &= V_1sC_{12} \\ s &= \frac{g_2}{C_{12}} \end{align}\]
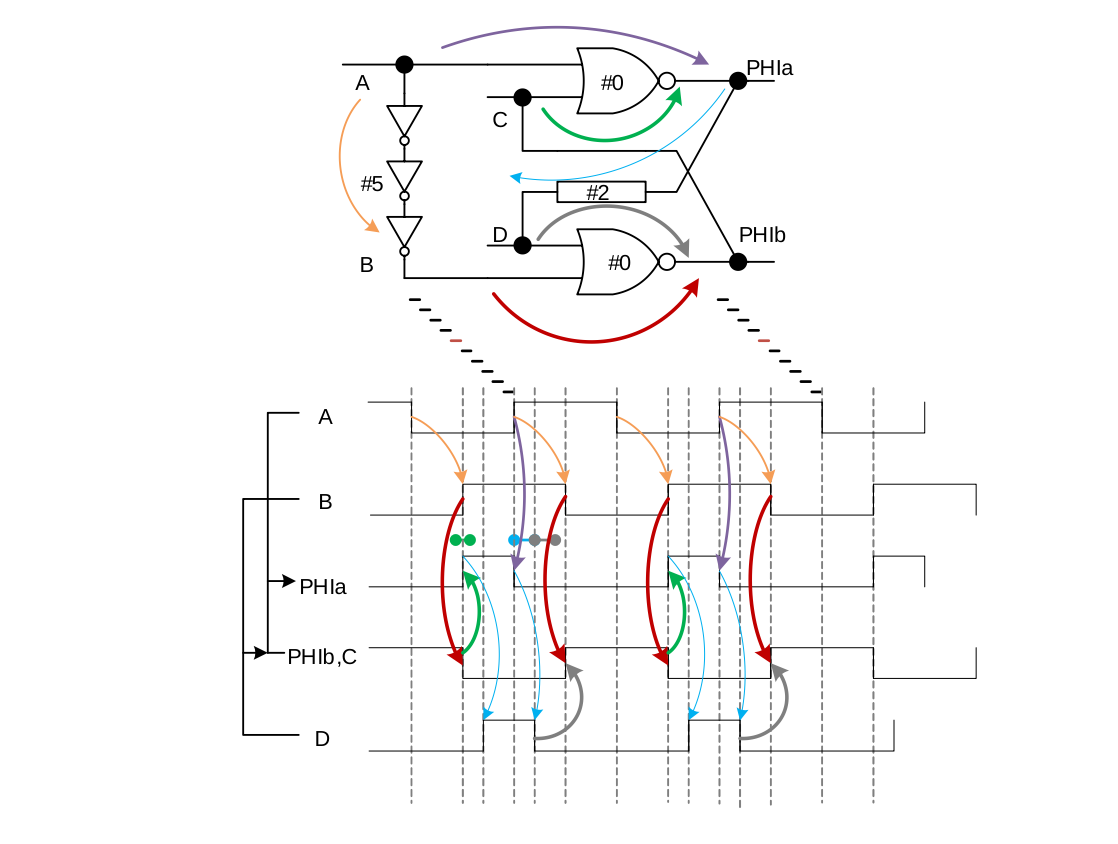
Nonoverlapping clock
Classical
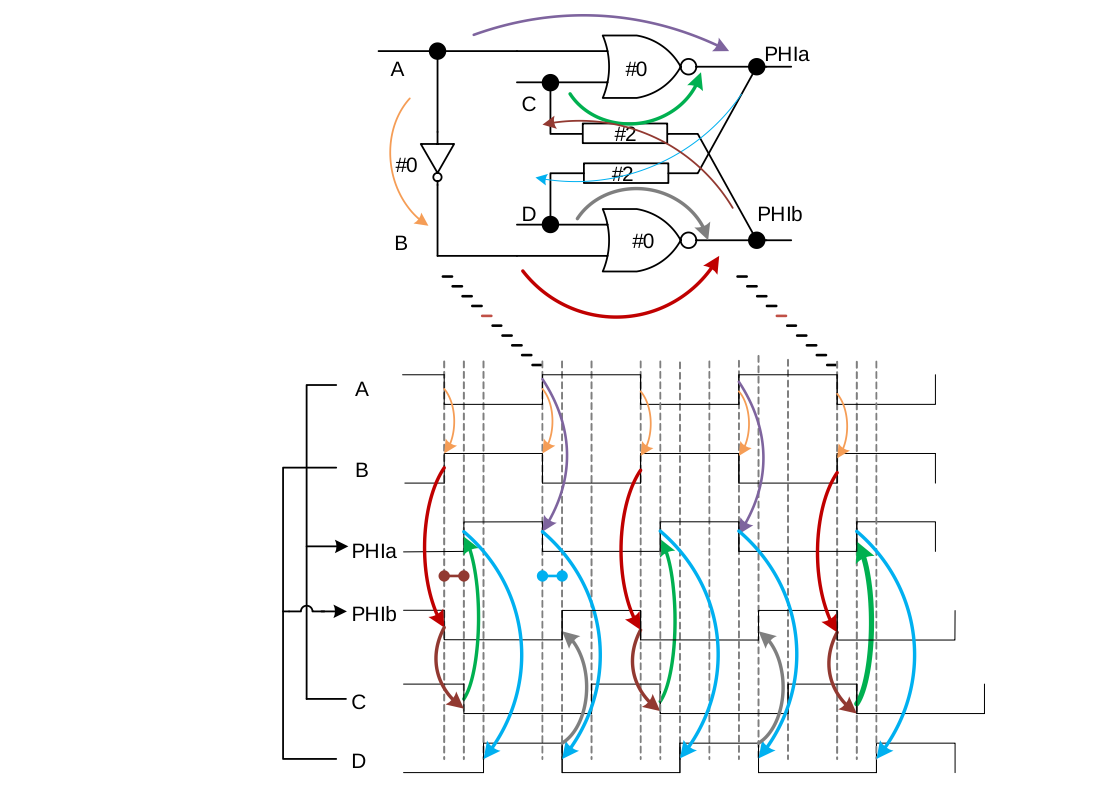
DWC
C2PHIa is important to ensure nonoverlapping and DelayA2B is due to level shifter
Single ended Amplifier Offset Voltage
unity gain buffer

\[\begin{align} V_o &= V_{o,dc}+A(V_p-V_m) \\ V_o' &= V_{o,dc}+A(V_p+V_{os}-V_m') \end{align}\]
Then, we get \[ V_{os}=\frac{V_o'-V_o}{A}+(V_m'-V_m) \] Due to \(V_o=V_m\) and \(V_o'=V_m'\) \[ V_{os}=(1/A+1)\Delta{V_m} \] or \[ V_{os}=(1/A+1)\Delta{V_o} \] if \(A \gg 1\) \[ V_{os}=\Delta{V_o} \]
non-inverting amplifier
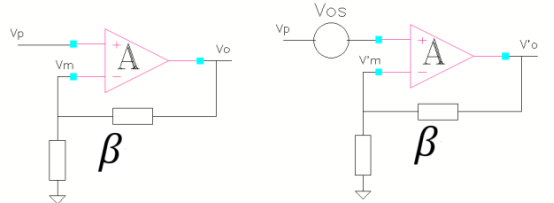 \[\begin{align}
V_o &= V_{o,dc}+A(V_p-V_m) \\
V_o' &= V_{o,dc}+A(V_p+V_{os}-V_m') \\
V_m &= \beta V_o \\
V_m' &= \beta V_o'
\end{align}\]
\[\begin{align}
V_o &= V_{o,dc}+A(V_p-V_m) \\
V_o' &= V_{o,dc}+A(V_p+V_{os}-V_m') \\
V_m &= \beta V_o \\
V_m' &= \beta V_o'
\end{align}\]
we get \[ V_{os}=\frac{V_o'-V_o}{A}+(V_m'-V_m) \] or \[ V_{os}=\frac{\Delta V_o}{A}+\beta \Delta V_o \] if \(A \gg 1\) \[ V_{os}=\beta \Delta V_o \] or \[ V_{os}=\Delta V_m \]
Lecture 22 Variability and Mismatch of Dr. Hesham A. Omran's Analog IC Design
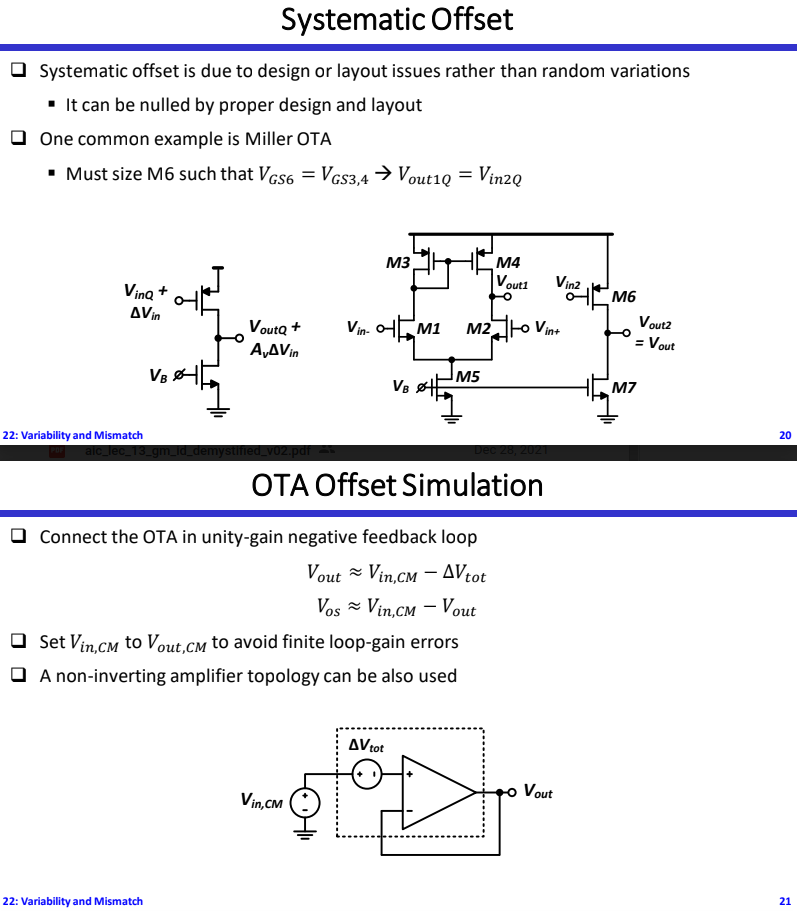
URL: https://www.master-micro.com/professional-courses/analog-ic-design/course-resources
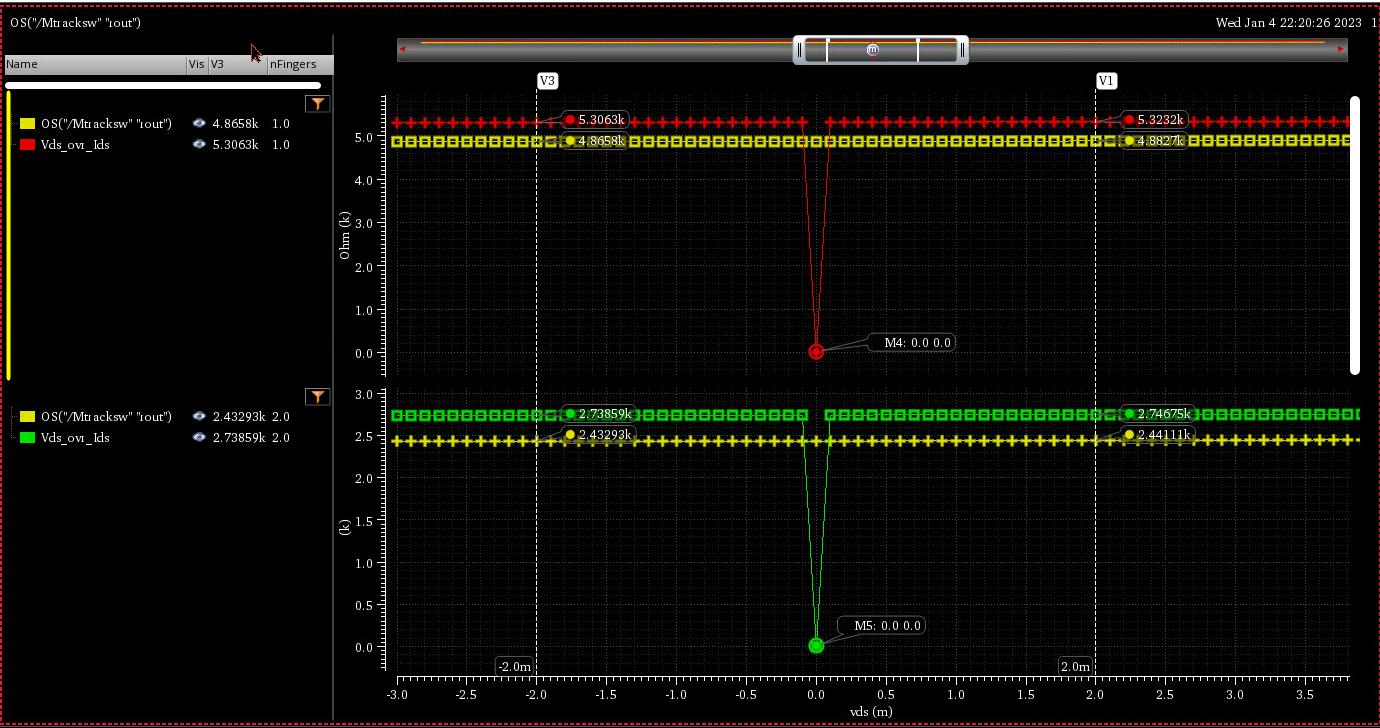
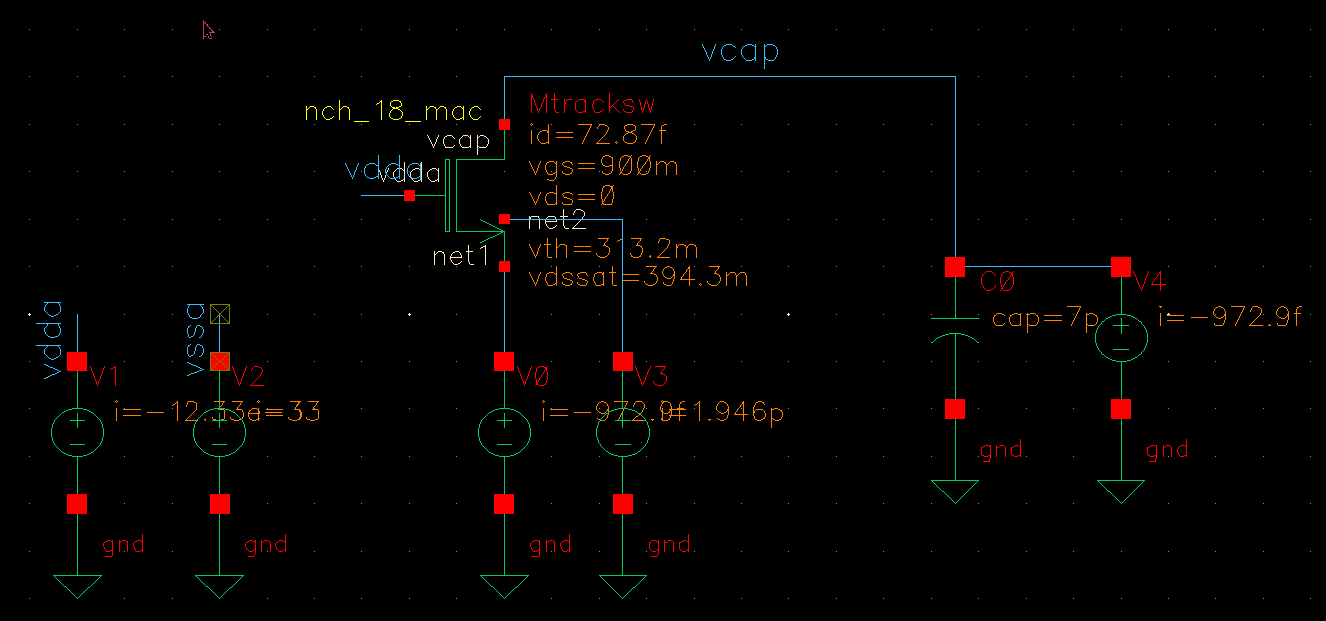
Gotcha MOS ron
There is discrepancy between model operating point and \(V_{ds}/I_{ds}\)
I believe that the equation \(V_{ds}/I_{ds}\) is more appropriate where mos is used as switch, though \(V_{ds}=0\) is an outlier.
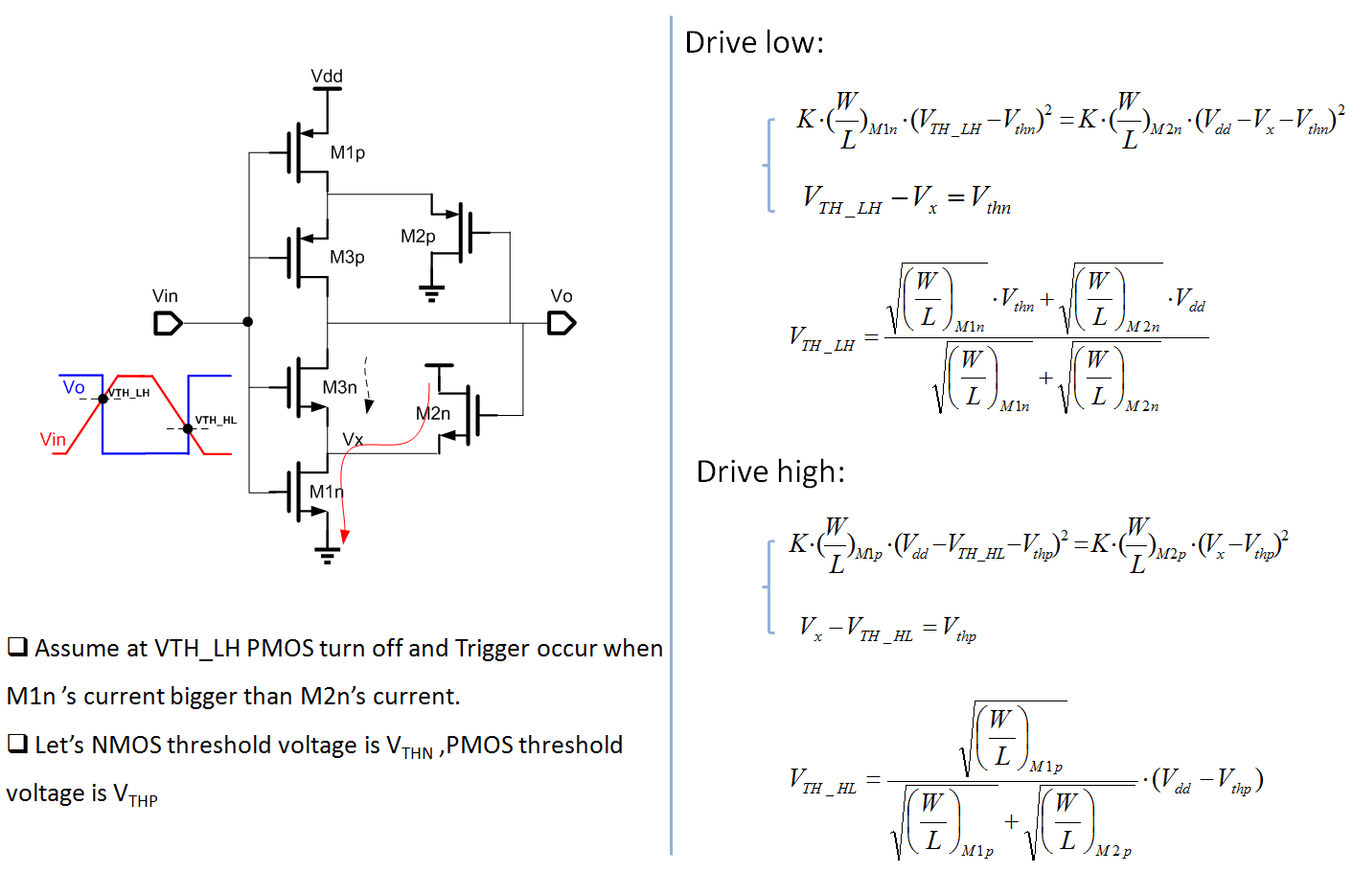
Schmitt Inverter
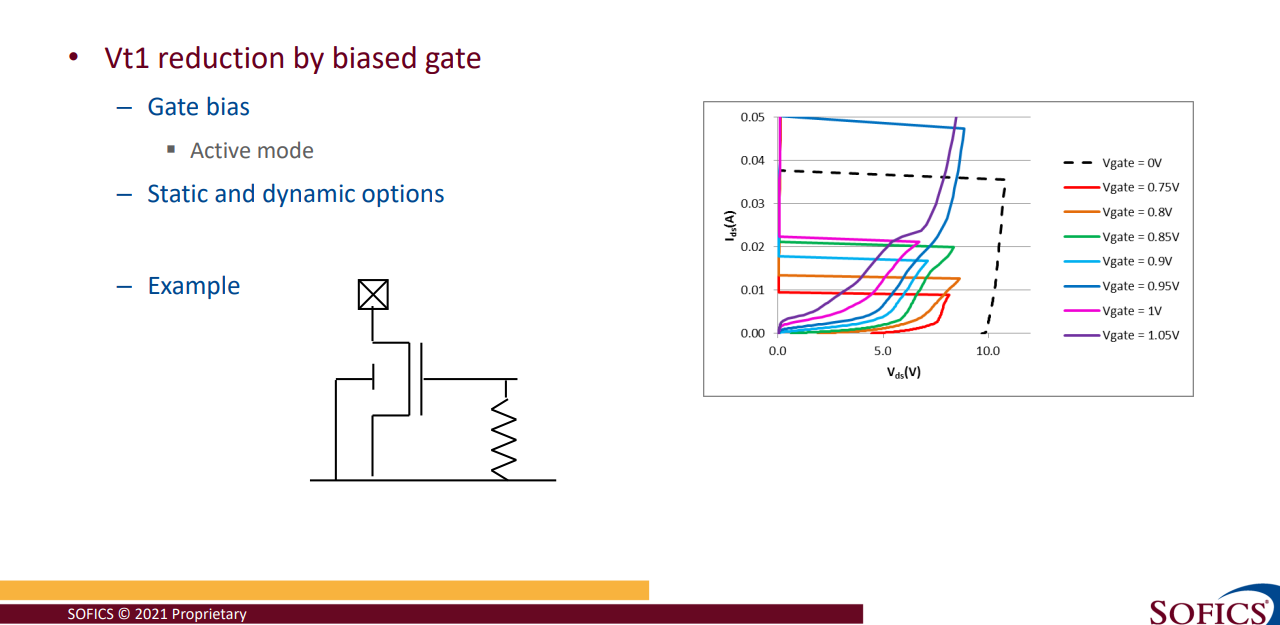
gm/ID Intuition

small gm/ID for High ro, or high Early voltage \(V_A\)
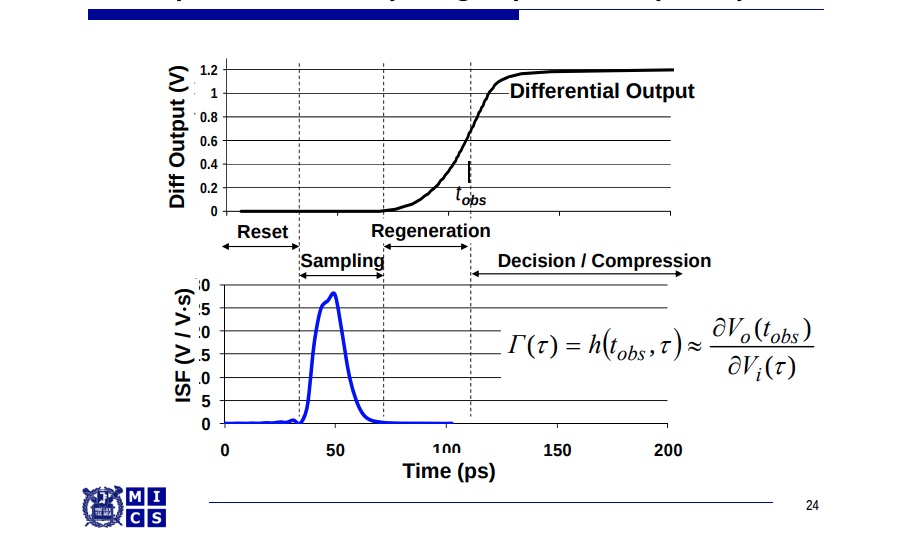
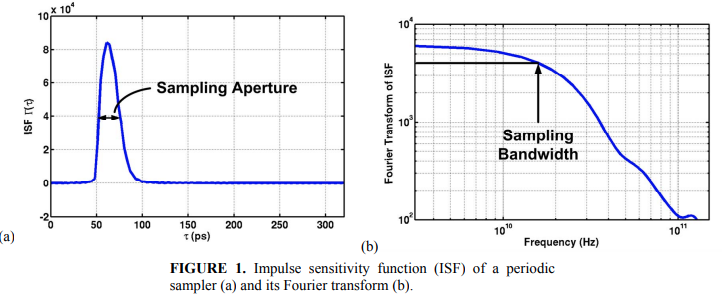
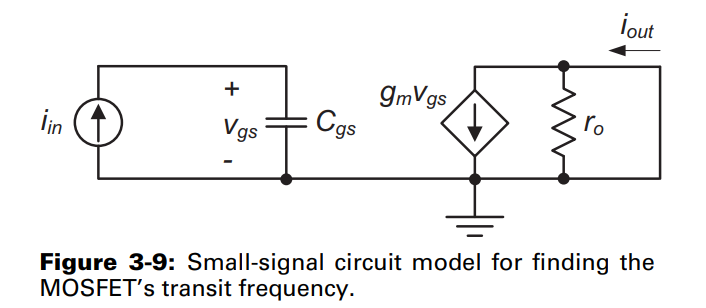
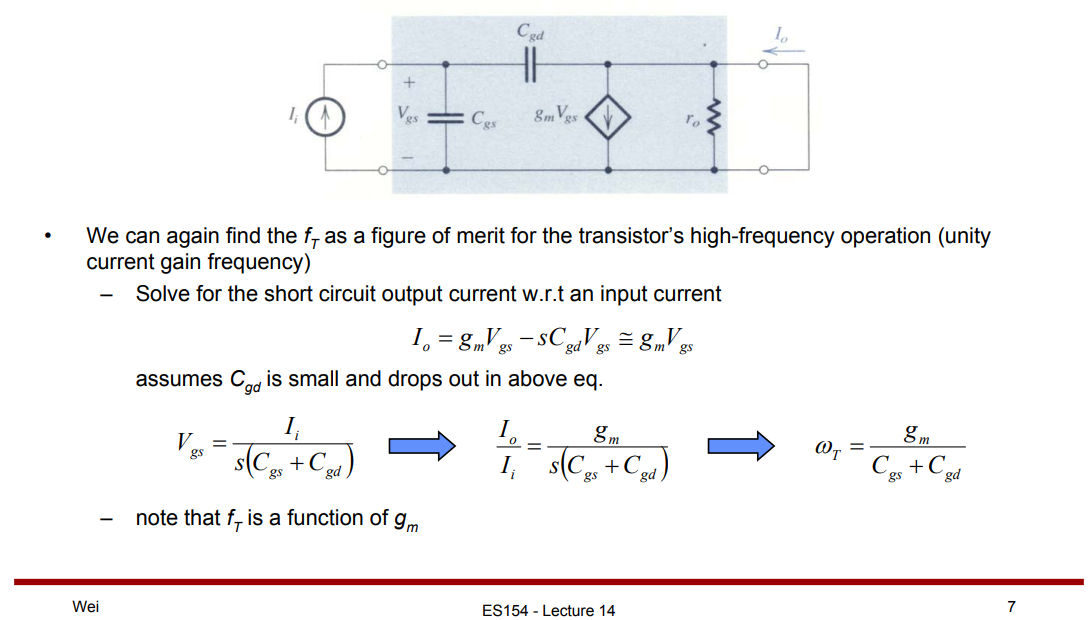
Transit Frequency \(f_T\)
Defined as the frequency at which the small-signal current gain of a device is unity
mag(Ids@ft) = Ig(1mA)
Aditya Varma Muppala. MMIC 08: High Frequency Device Characterization in Cadence - Fmax, Ft, NFmin vs Jd [https://youtu.be/kgEypIA8eus?si=sd4581x2hOuhsJ3P]
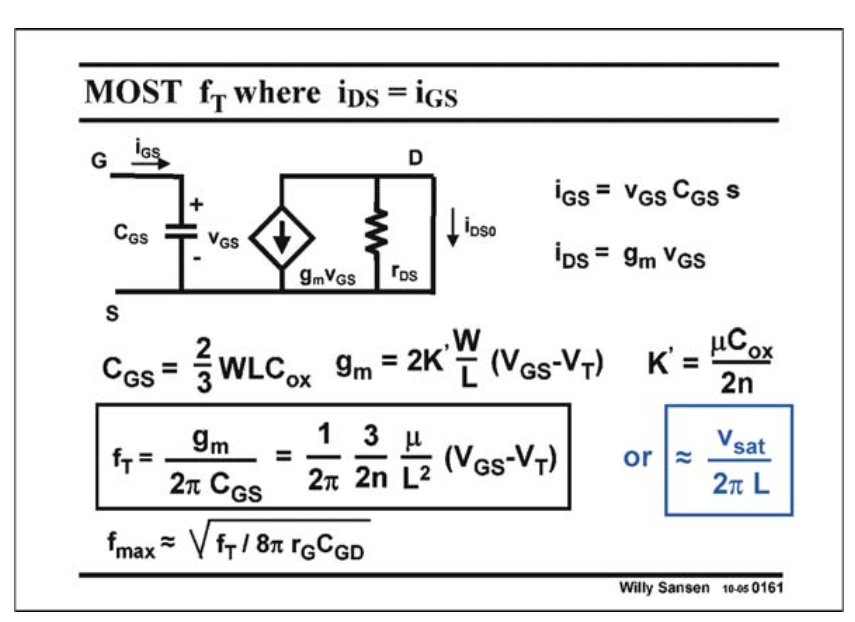
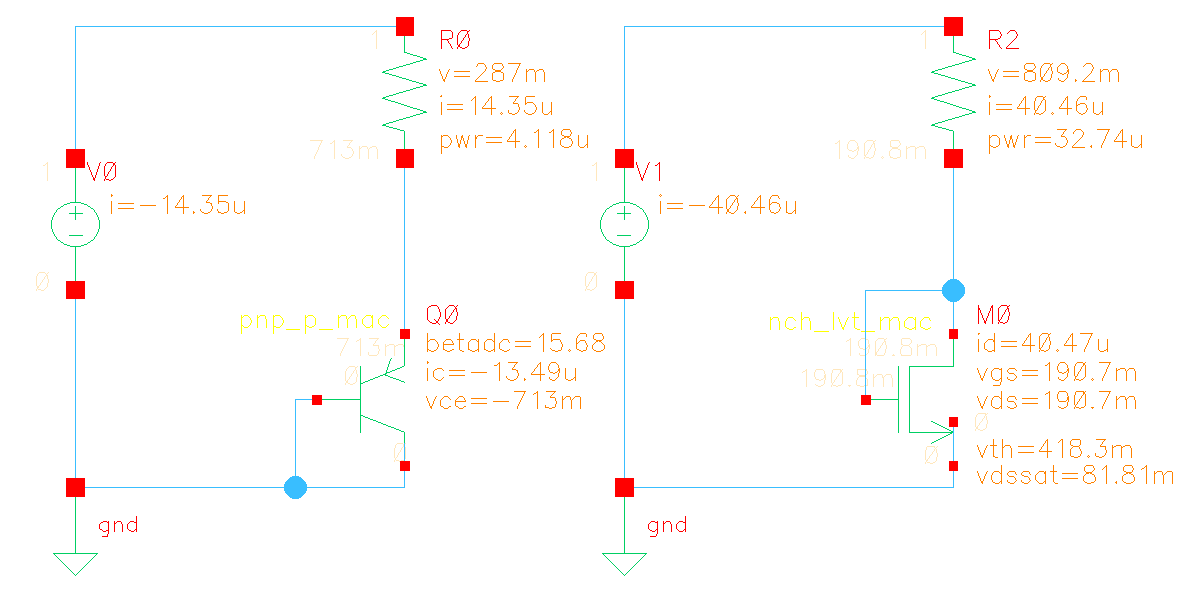
MOSFET ZTC Condition Analysis
M. Coelho et al., "Is There a ZTC Biasing Point in the Leading-Edge FET Intrinsic Gain gmrDS?," 2025 9th International Young Engineers Forum on Electrical and Computer Engineering (YEF-ECE), Caparica / Lisbon, Portugal, 2025
zero temperature coefficient (ZTC)
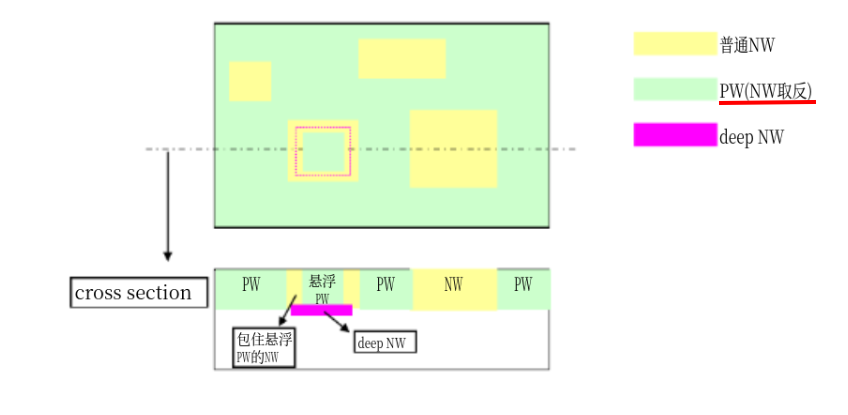
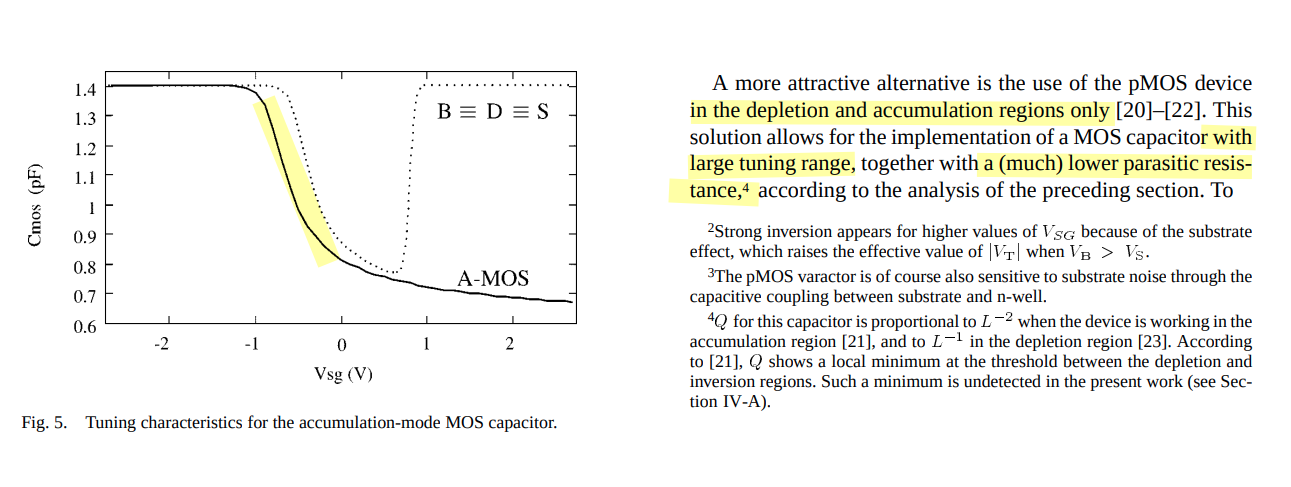
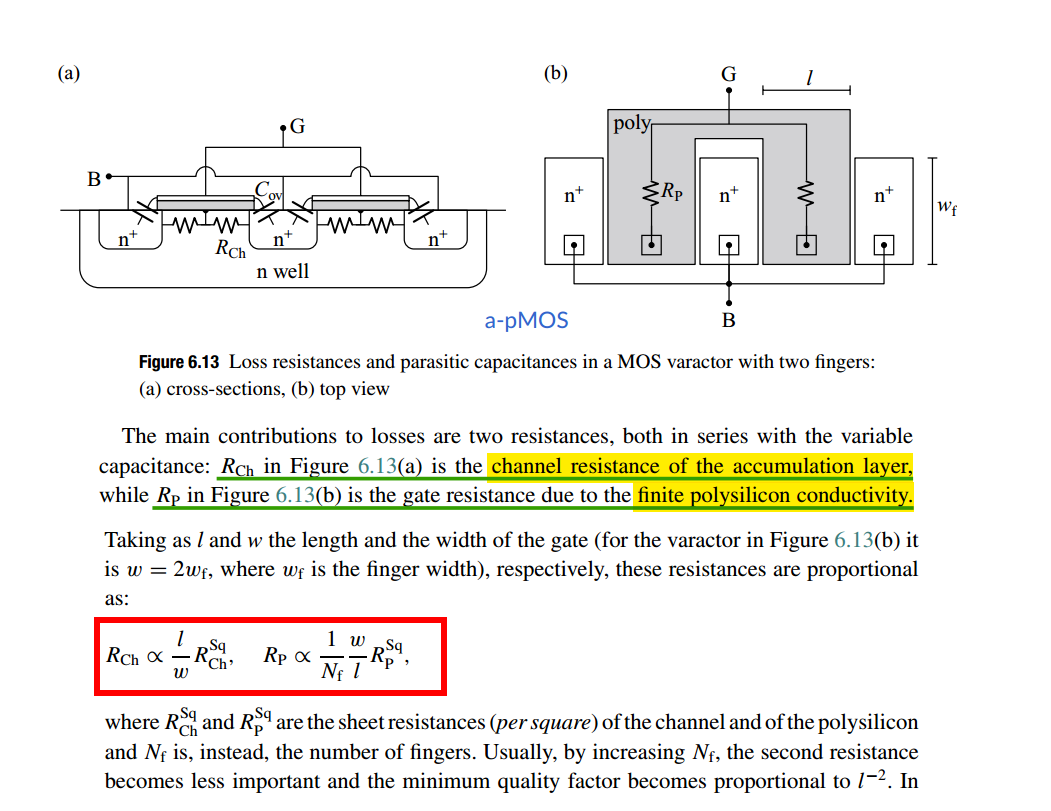
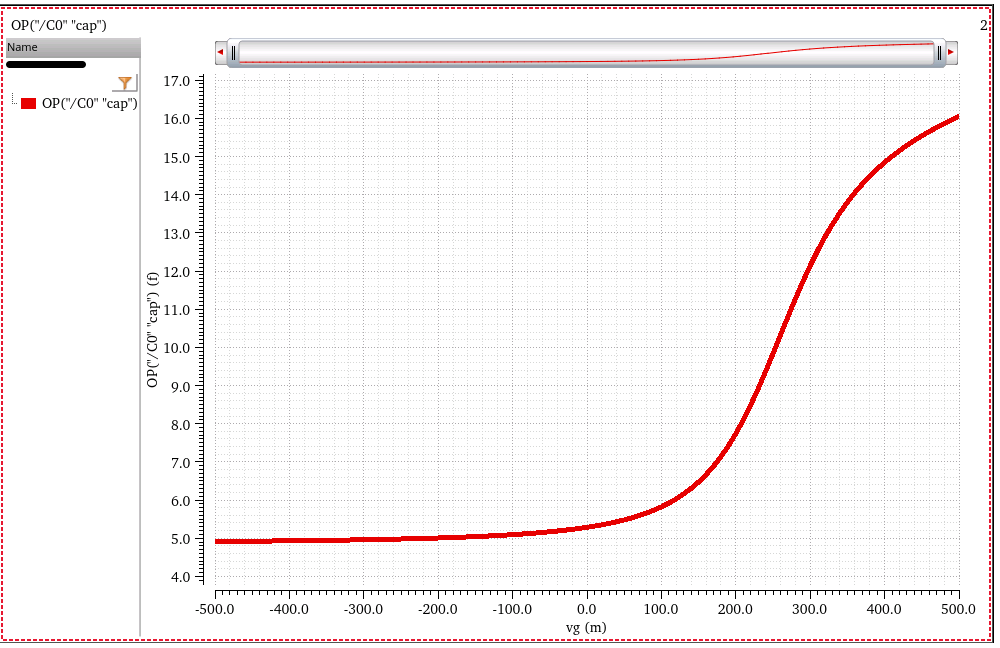
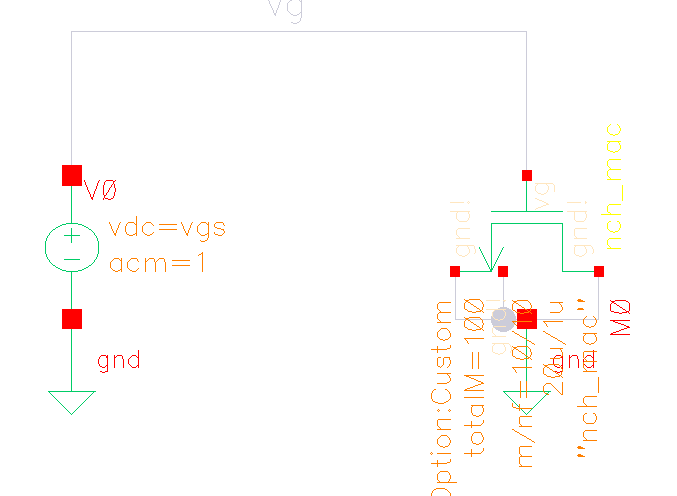
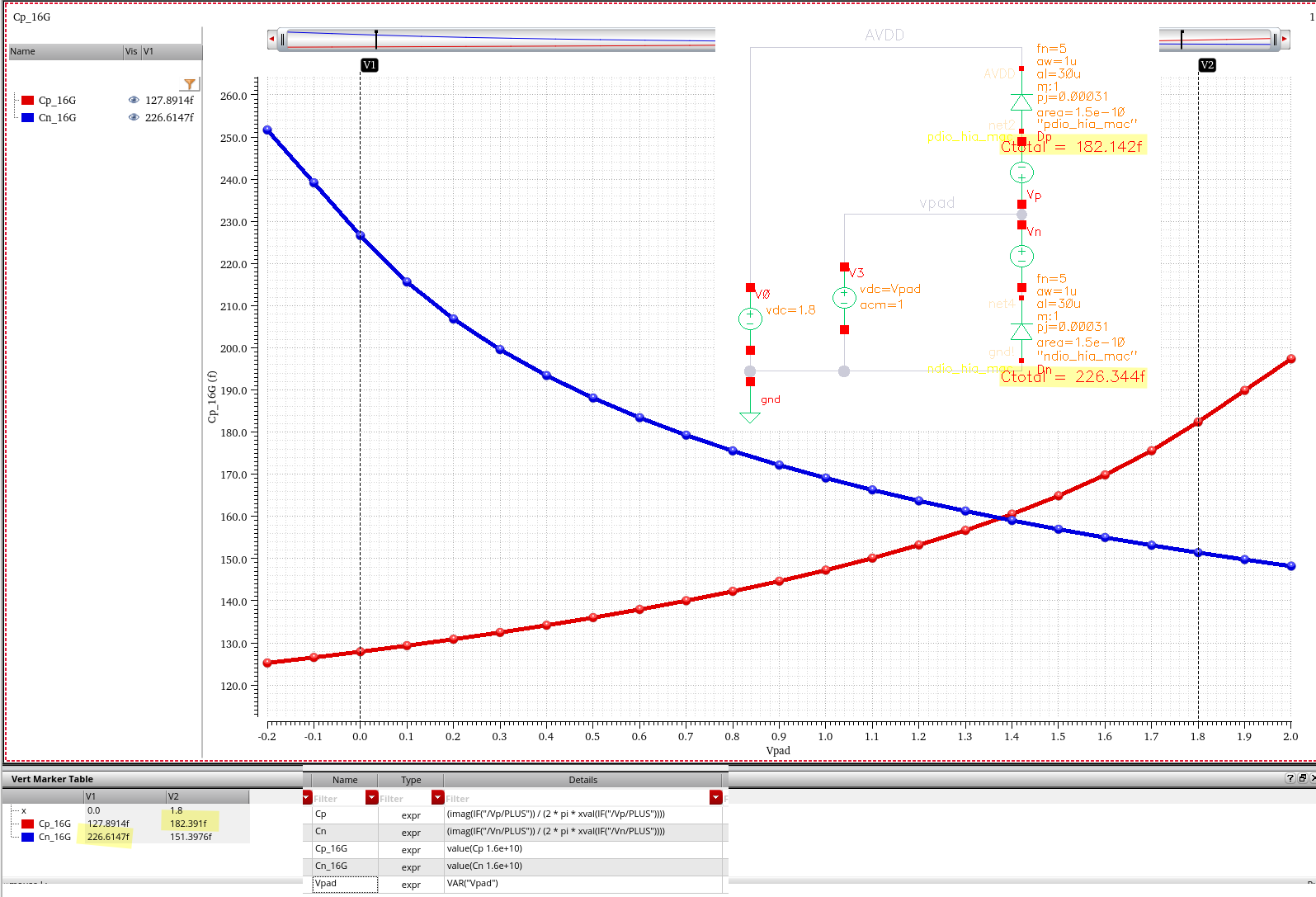
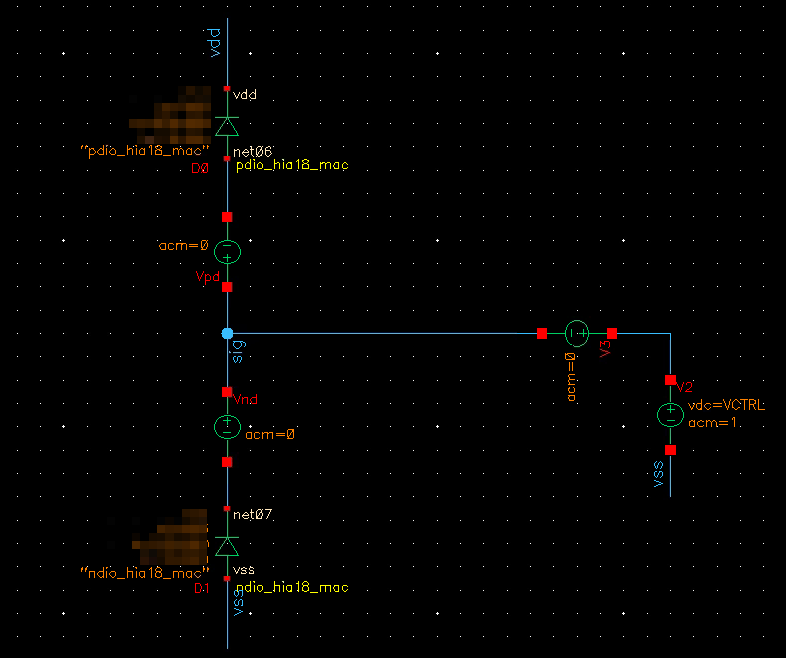
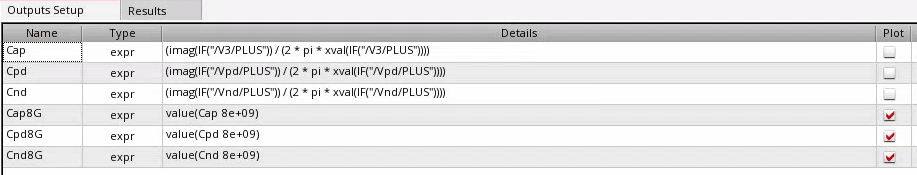
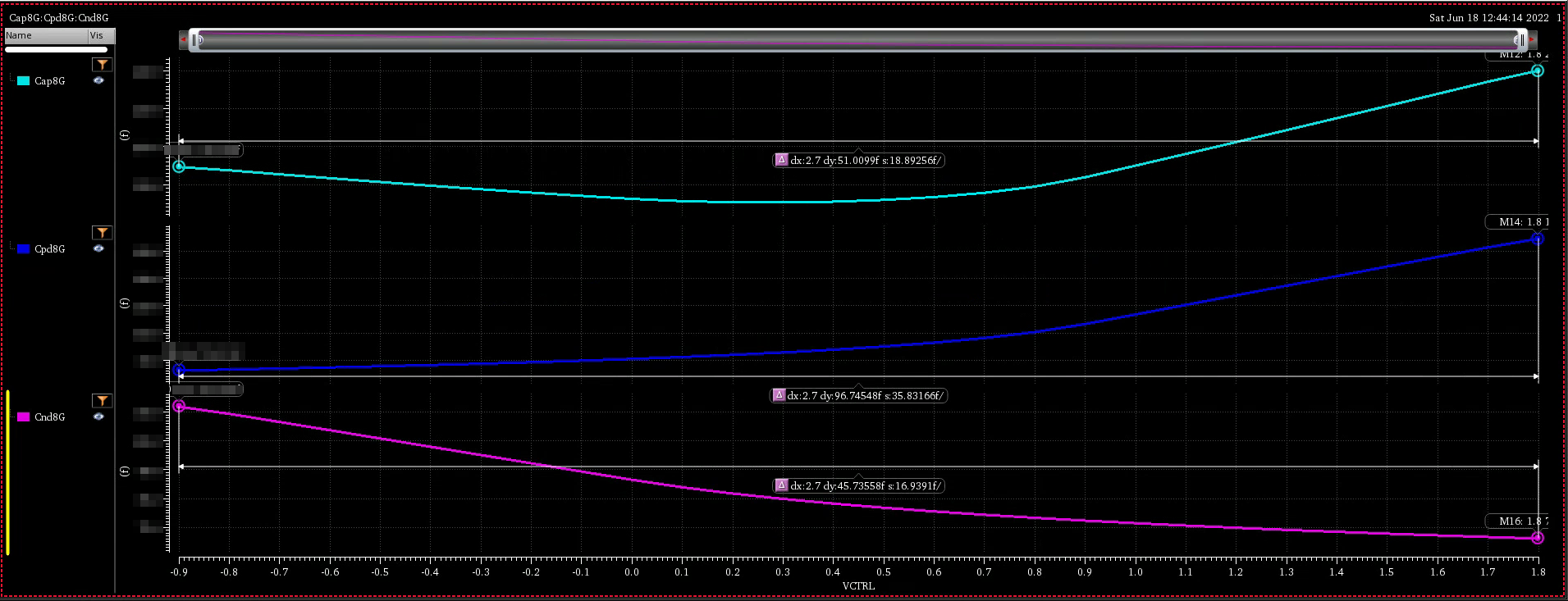
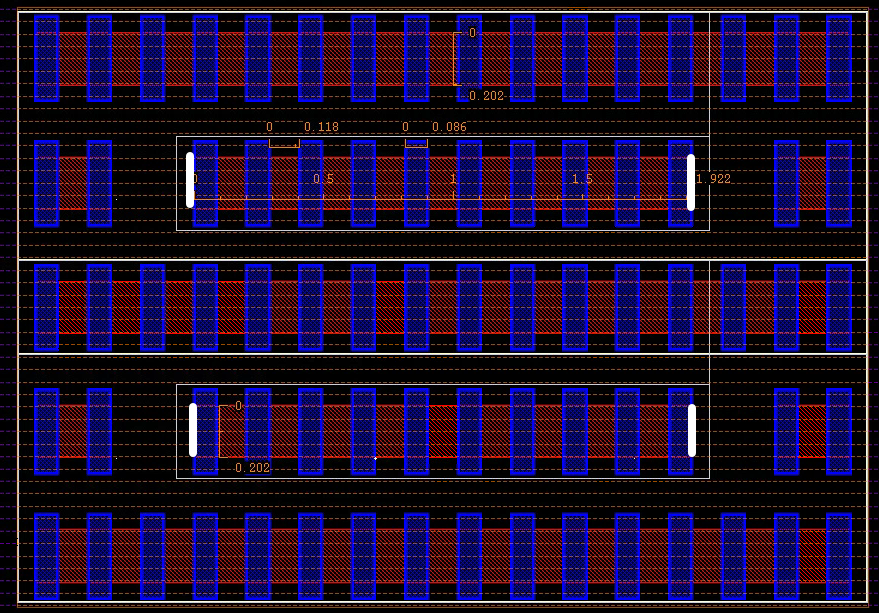
MOM cap of wo_mx
Monte Carlo model:
- \(C_{pa}=C_{pa1}\), \(C_{pb}=C_{pb1}\) for each iteration during Process Variation
- different variation is applied to \(C_{ab}\) and \(C_{a1b1}\) each iteration during Mismatch Variation, though \(C_{pa}\), \(C_{pb}\), \(C_{pa1}\) and \(C_{pb1}\) remain constant


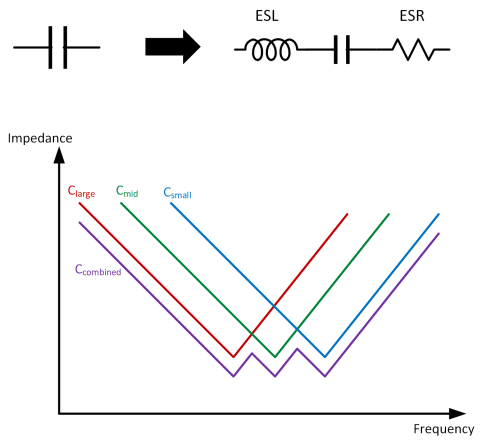
Miller multiplication of Capacitor
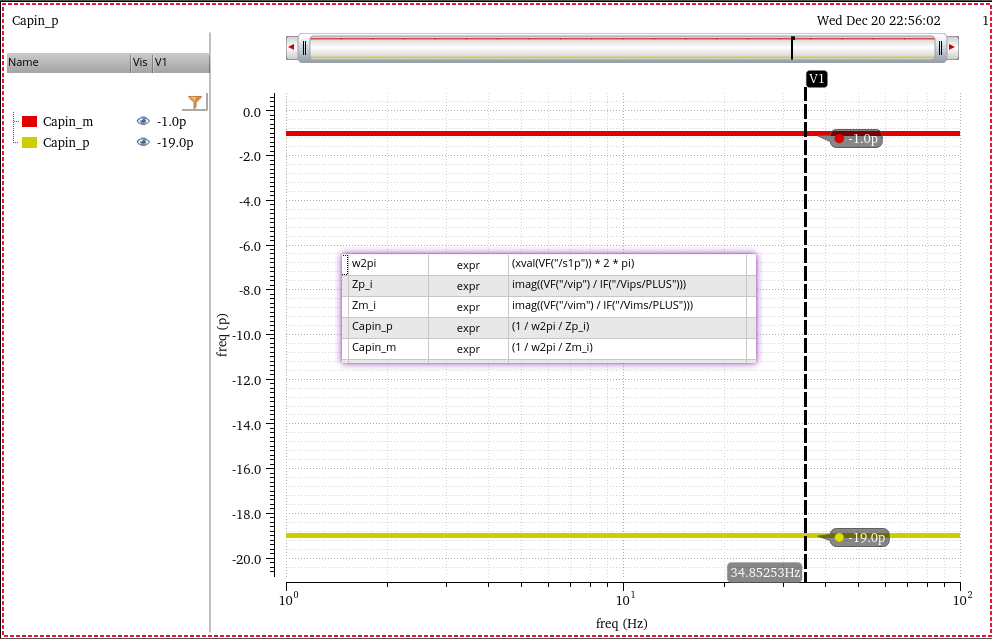
Positive Cap
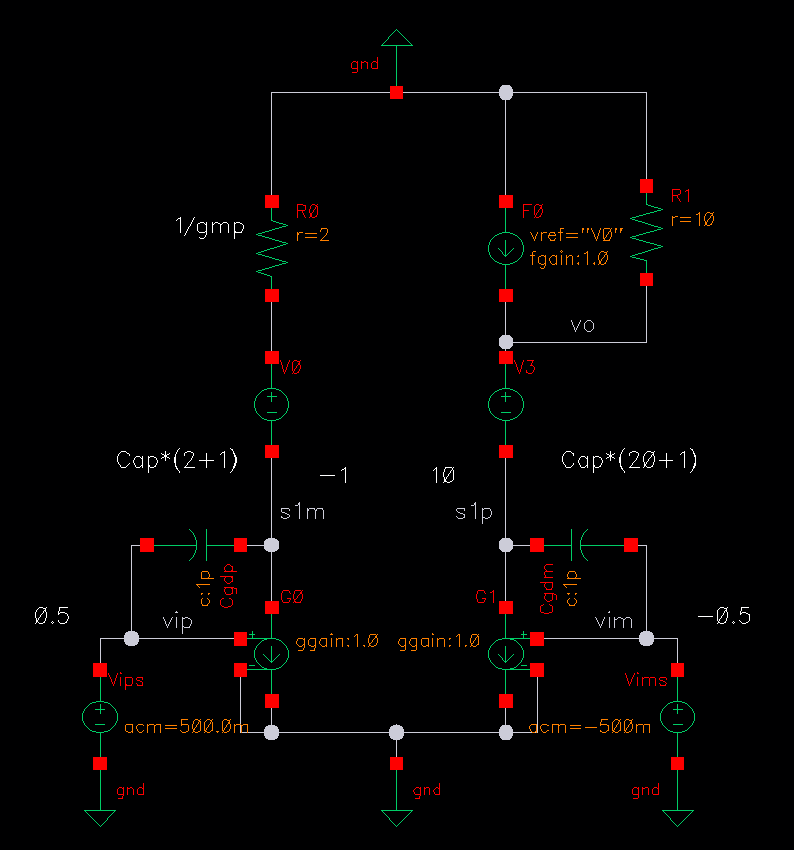
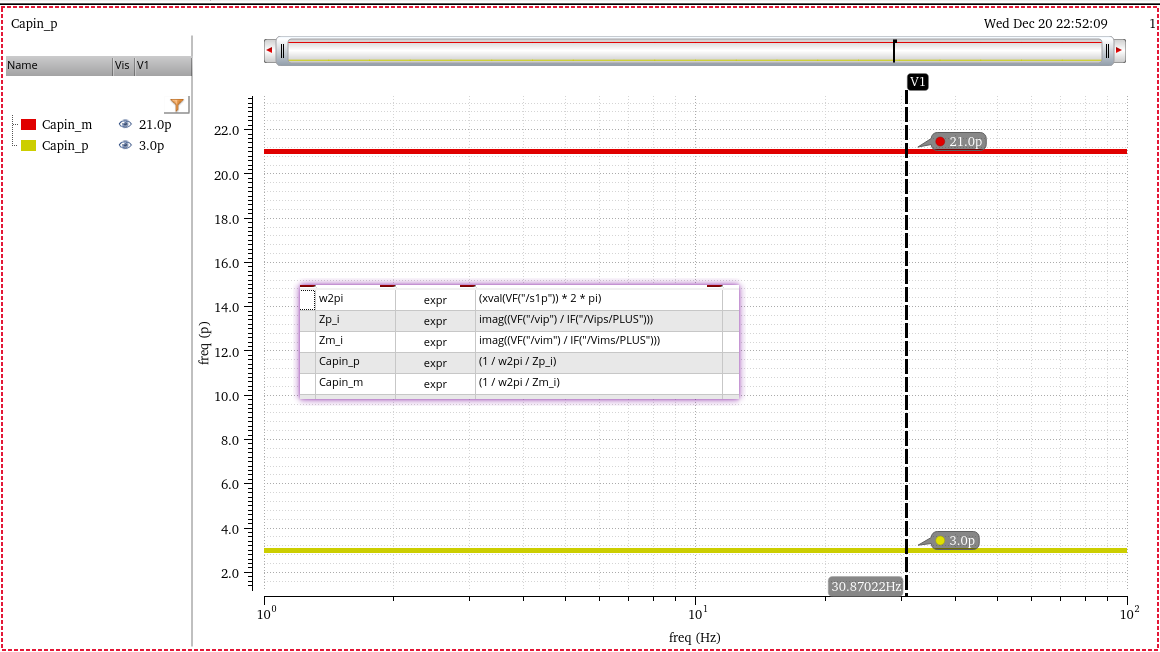
Negative Cap

gain has limited bandwidth
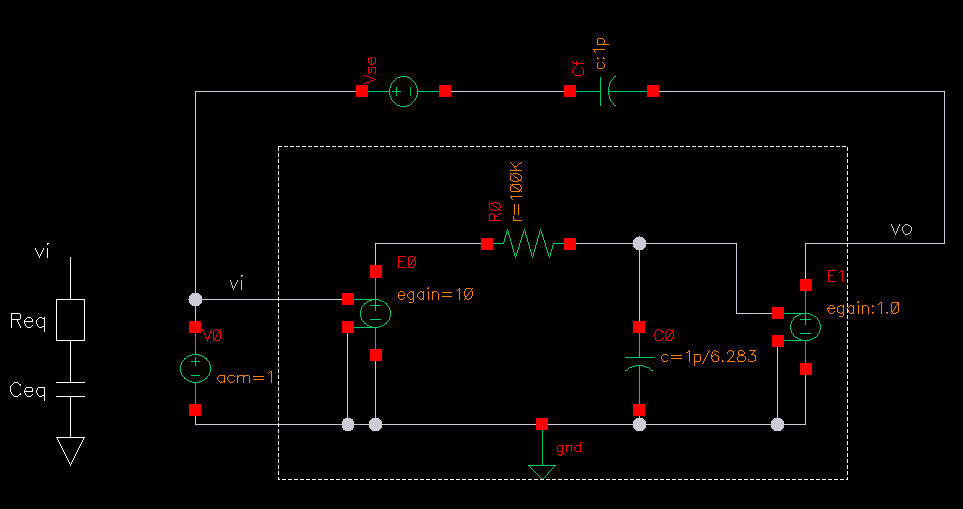
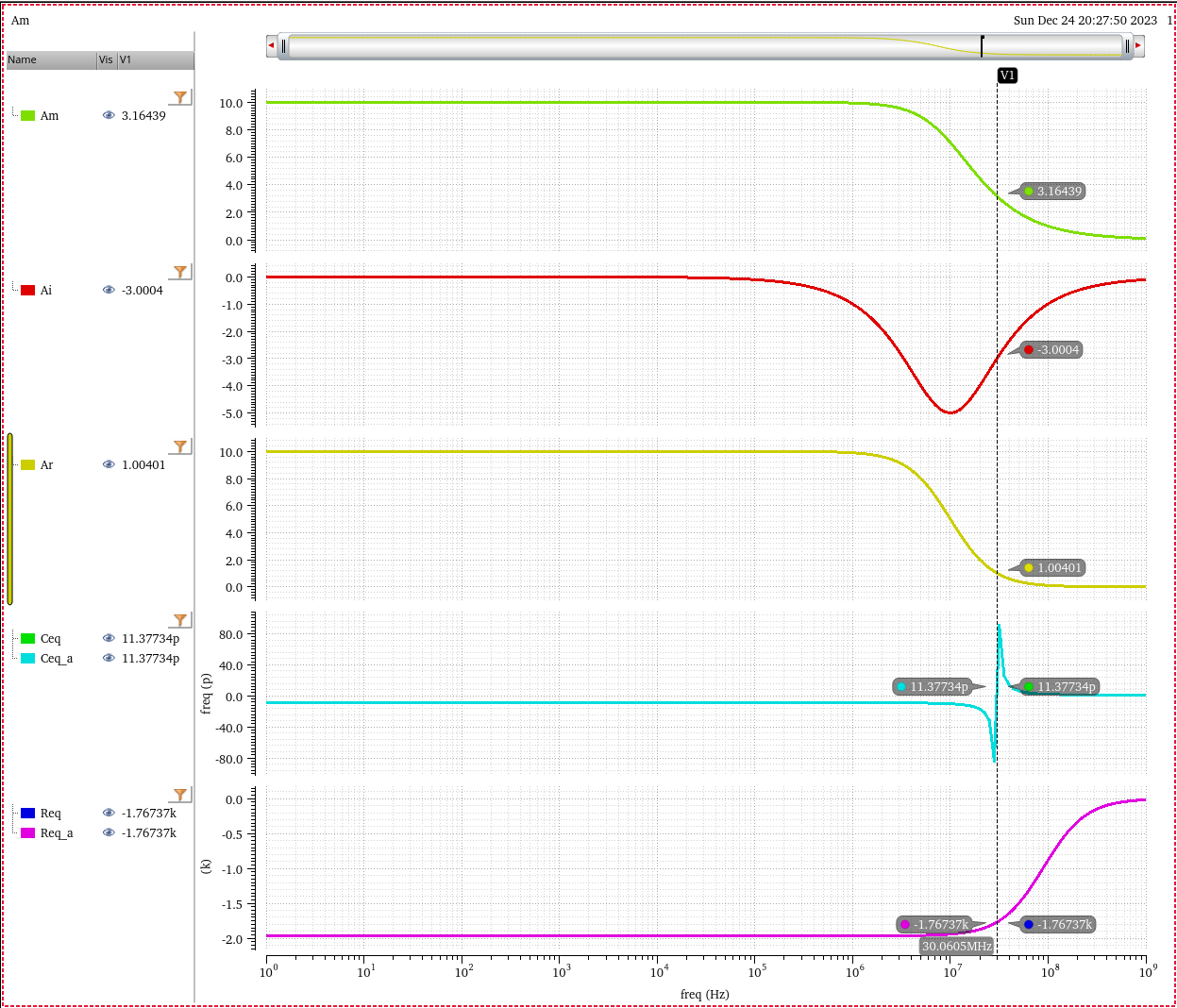
\(V_o = V_i |A|e^{j\theta}\), and \(A_r = |A|\cos\theta\), \(A_i = |A|\sin\theta\)
Then \(I_i = (V_i - V_o)sC_f= V_i(1-|A|e^{j\theta})sC_f\), impedance is shown as below
\[\begin{align} Z &= \frac{V_i}{I_i} \\ &= \frac{1}{(1-|A|e^{j\theta})j\omega C_f} \\ &= -\frac{j}{\omega C_f\frac{1+|A|^2-2|A|\cos\theta}{1-|A|\cos\theta}} + \frac{|A|\sin\theta}{\omega C_f (1+|A|^2-2|A|\cos\theta)} \\ \end{align}\]
\(C_\text{eq}\) and \(R_\text{eq}\) are obtained \[\begin{align} C_\text{eq} &= \frac{1+|A|^2-2A_r}{1-A_r}\cdot C_f \\ R_\text{eq} &= \frac{A_i}{1+|A|^2-2A_r}\cdot \frac{1}{\omega C_f} \end{align}\]
D/S small signal model
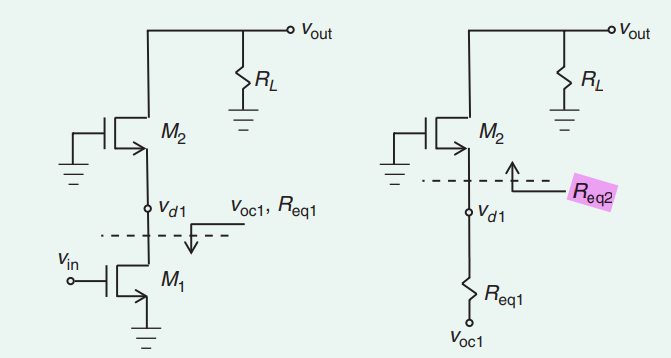
The
DrainandSourceof MOS are determined in DC operating point, i.e. large signal.
That is, top of \(M_2\) is
drain and bottom is source, \[\begin{align}
R_\text{eq2} &= \frac{r_\text{o2}+R_L}{1+g_\text{m2}r_\text{o2}} \\
& \simeq \frac{1}{g_\text{m2}}
\end{align}\]
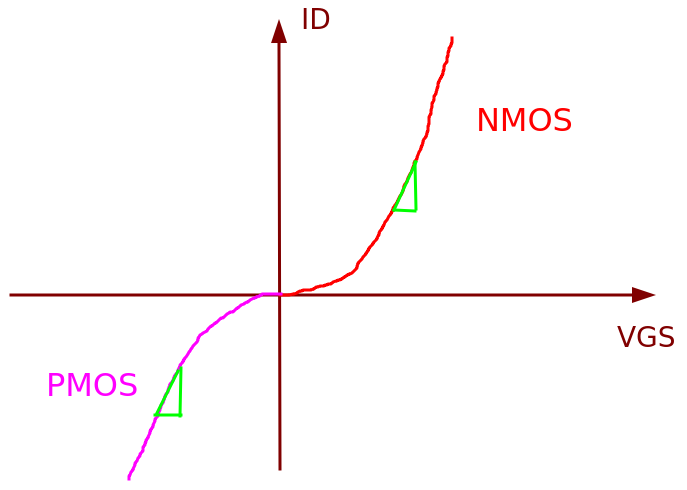
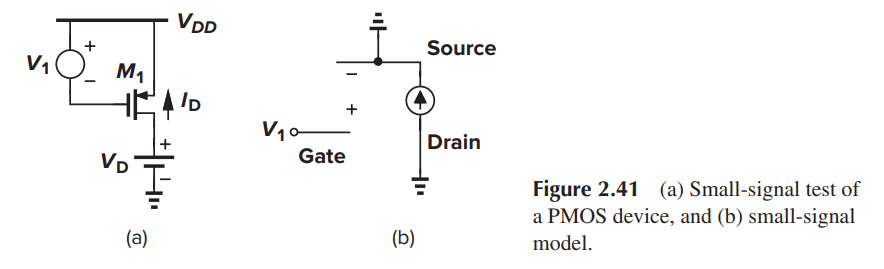
PMOS small signal model polarity
The small-signal models of NMOS and PMOS transistors are identical
A negative \(\Delta V_\text{GS}\) leads to a negative \(\Delta I_D\).
Recall that \(I_D\), in the direction shown here, is negative because the actual current of holes flows from the source to the drain.
Conversely, a positive \(\Delta V_\text{GS}\) produces a positive \(\Delta I_D\), as is the case for an NMOS device.
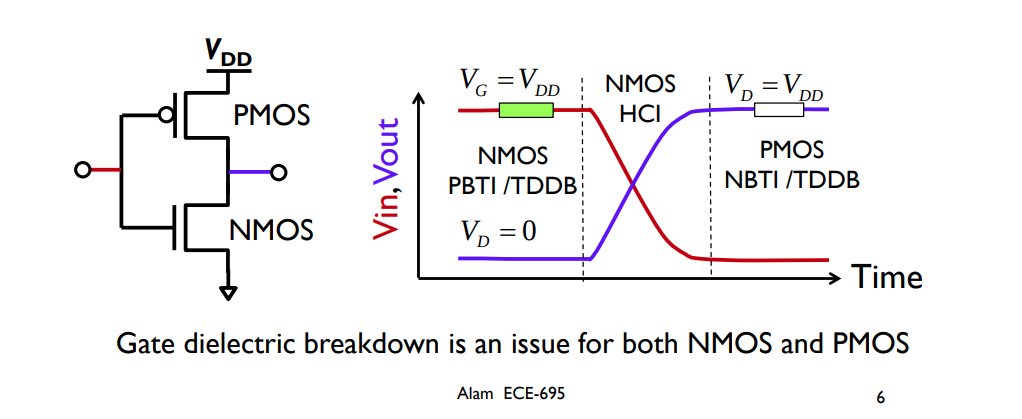
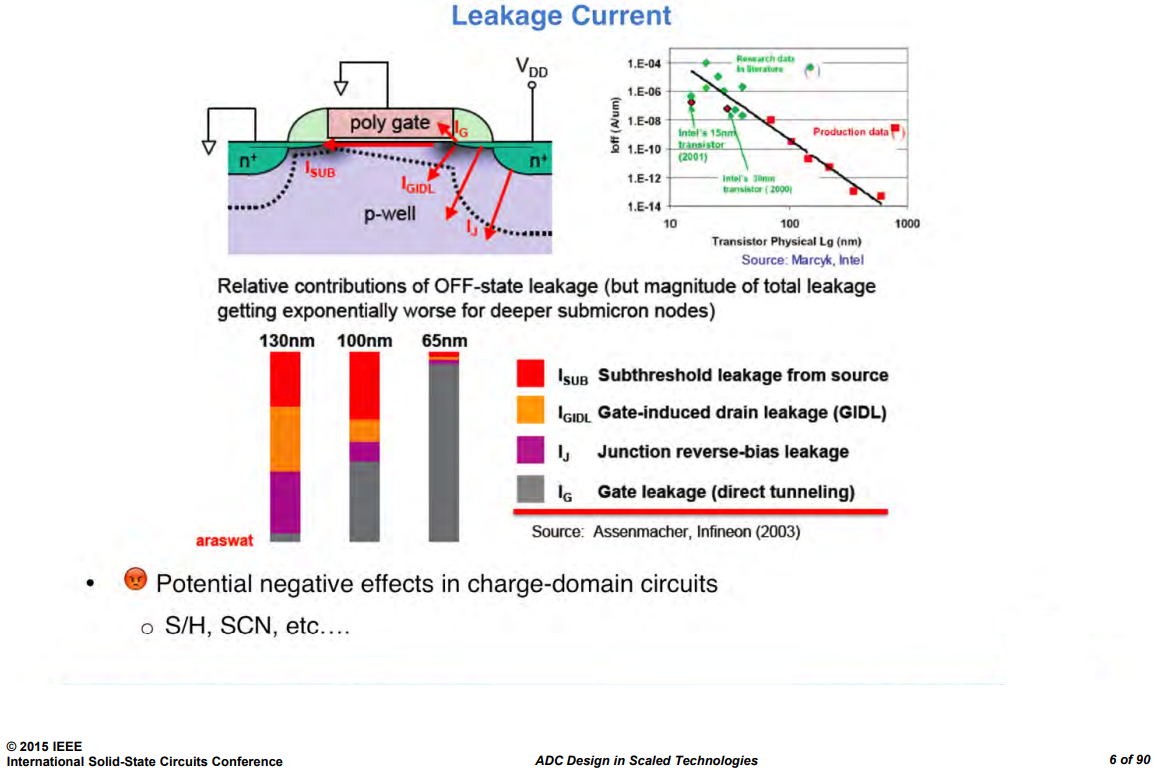
Leakage in MOS
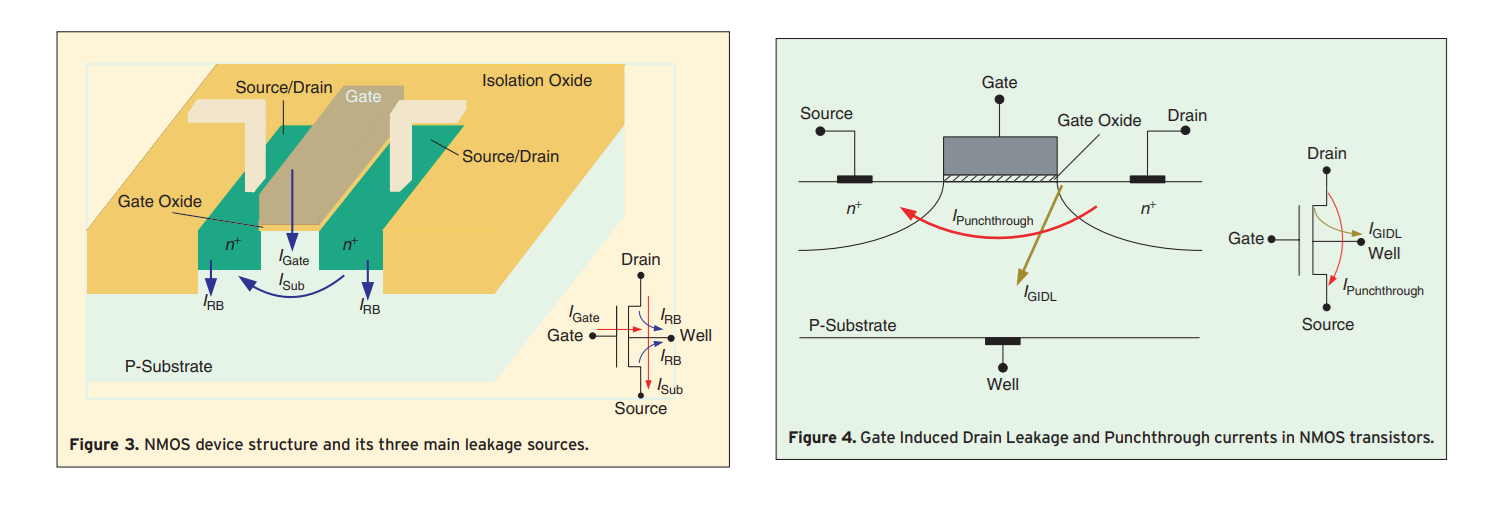
- Subthreshold leakage
- Drain-Induced Barrier Lowering (DIBL)
- Reverse-bias Source/Drain junction leakages
- Gate leakage
- two other leakage mechanisms
- Gate Induced Drain Leakage (GIDL)
- Punchthrough
W. M. Elgharbawy and M. A. Bayoumi, "Leakage sources and possible solutions in nanometer CMOS technologies," in IEEE Circuits and Systems Magazine, vol. 5, no. 4, pp. 6-17, Fourth Quarter 2005, doi: 10.1109/MCAS.2005.1550165.
X. Qi et al., "Efficient subthreshold leakage current optimization - Leakage current optimization and layout migration for 90- and 65- nm ASIC libraries," in IEEE Circuits and Devices Magazine, vol. 22, no. 5, pp. 39-47, Sept.-Oct. 2006, doi: 10.1109/MCD.2006.272999.
P. Monsurró, S. Pennisi, G. Scotti and A. Trifiletti, "Exploiting the Body of MOS Devices for High Performance Analog Design," in IEEE Circuits and Systems Magazine, vol. 11, no. 4, pp. 8-23, Fourthquarter 2011, doi: 10.1109/MCAS.2011.942751.
Andrea Baschirotto, ISSCC2015 "ADC Design in Scaled Technologies"
Joachim Assenmacher Infineon Technologies, "BSIM4 Modeling and Parameter Extraction" [https://ewh.ieee.org/r5/denver/sscs/References/2003_03_Assenmacher.pdf]
Stefan Rusu, Intel ISSCC 2008 Tutorial: "Leakage Reduction Techniques" [https://www.nishanchettri.com/isscc-slides/2008%20ISSCC/Tutorials/T06_Pres.pdf]
Drain-Induced Barrier Lowering (DIBL)
As a result of DIBL, threshold voltage is reduced with shorter channel lengths and, consequently, the subthreshold leakage current is increased
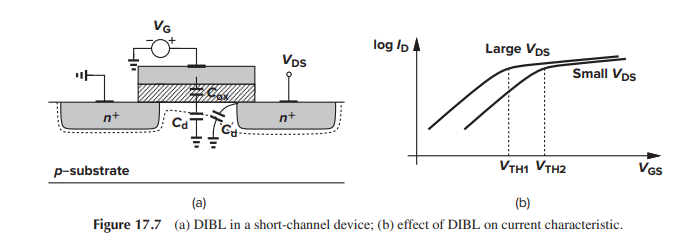
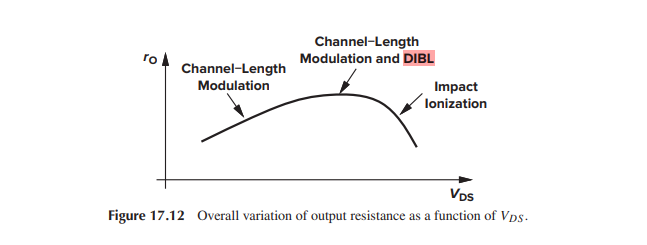
impact on output impedance
The principal impact of DIBL on circuit design is the degraded output impedance.
In short-channel devices, as \(V_{DS}\) increases further, drain-induced barrier lowering becomes significant, reducing the threshold voltage and increasing the drain current
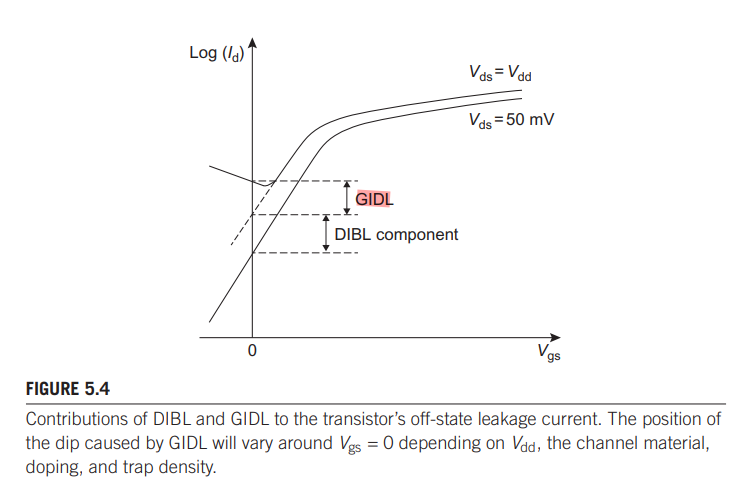
Impact Ionization and GIDL are different, however both increase drain current, which flowing from the drain into the substrate
Gate induced drain leakage (GIDL)
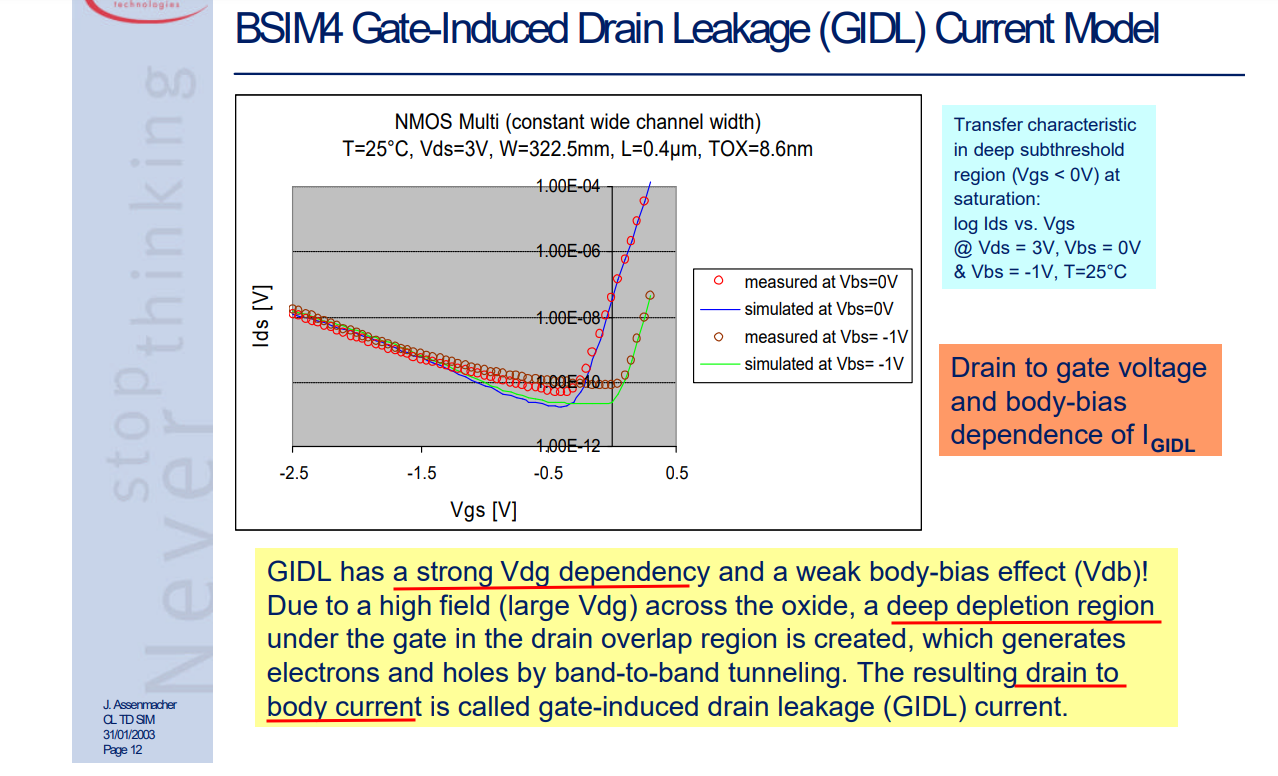
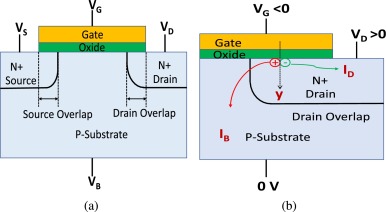
The large current flows from the drain to bulk and this drain leakage current is named gate-induced drain leakage (GIDL) since it is due to a gate-induced high electric field present in the gate-to-drain overlap region
gate-induced drain leakage (GIDL) increases exponentially due to the reduced gate oxide thickness
Chauhan, Yogesh Singh, et al. FinFET modeling for IC simulation and design: using the BSIM-CMG standard. Academic Press, 2015.
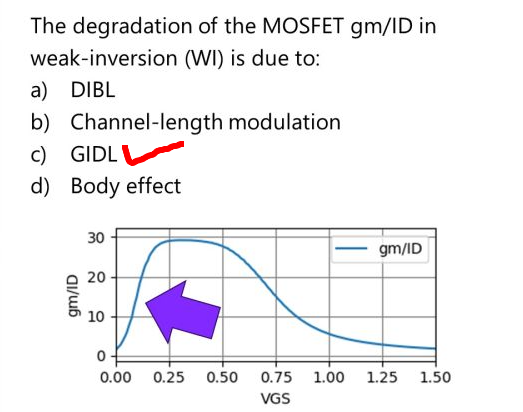
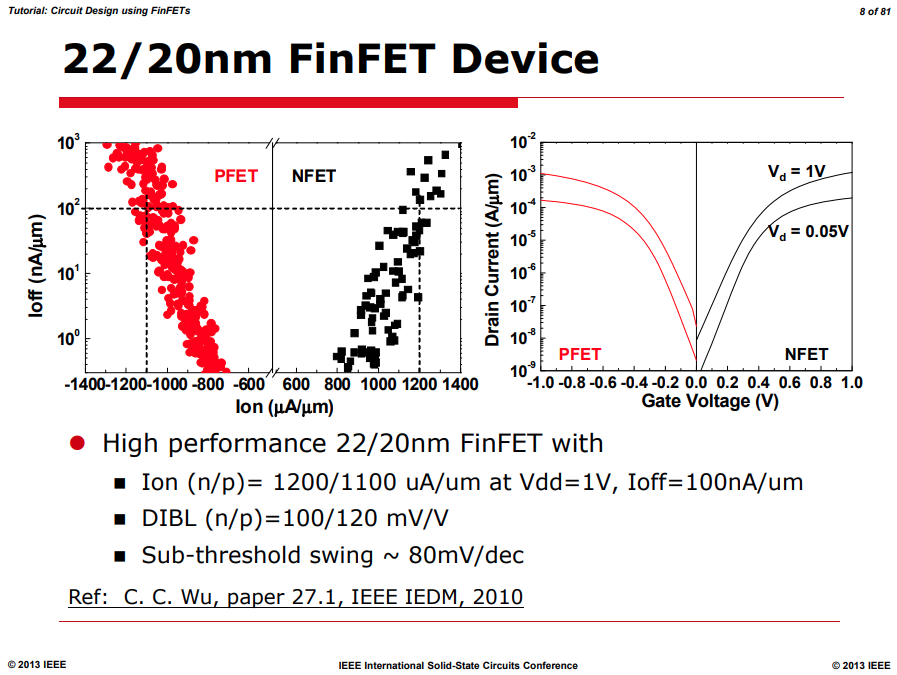
\[ \frac{g_m}{I_D} = \frac{2}{V_{GS}-V_{TH}} \] Decrease of gm/Id results from decrease in VT.
GIDL (Gate induced drain leakage) as at weak inversion may results in a weak lateral electric field causing leakage current between drain and bulk, which degrade the efficiency of the transistor (gm/ID).
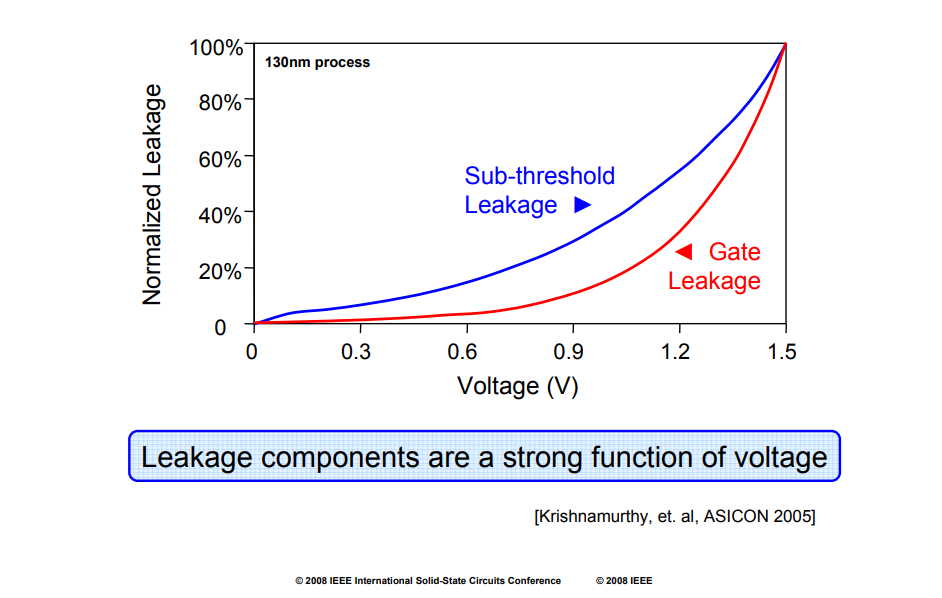
Voltage Dependence
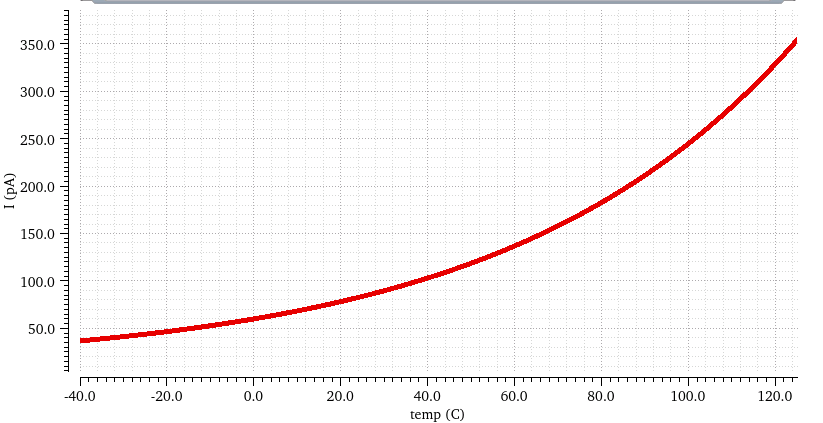
Temperature Dependence
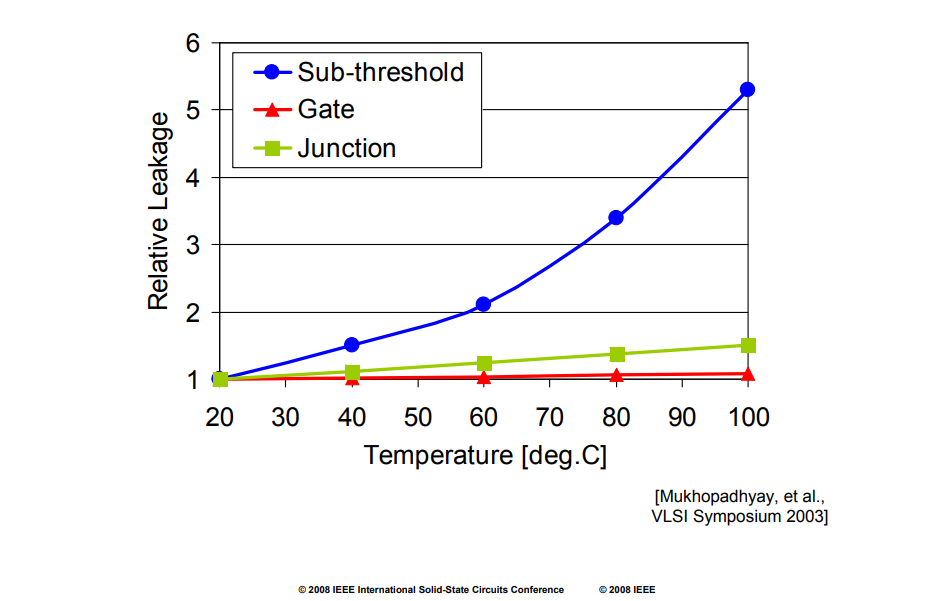
In advanced node, gate leakage is also a strong function of temperature
signal detection circuit
phase I
\[\begin{align} Q_a &= (V_{a0} - 0.5*(V_{ip} + V_{im}))*C + (V_{a0} - V_{th})*C \\ Q_b &= (V_{b0} - 0.5*(V_{ip} + V_{im}))*C + V_{b0}*C \end{align}\]
Phase II
\[\begin{align} Q_a &= (V_{a} - V_{ip})*C + (V_{a} - V_{b})*0.5C \\ Q_b &= (V_{b} - V_{im})*C + (V_{b} - V_{a})*0.5C \end{align}\]
With the law of charge conservation, we get
\[\begin{equation} V_a - V_b = (V_{a0} - V_{b0}) + 0.5*(V_{ip} - V_{im} - V_{th}) \end{equation}\]
REF: D. A. Yokoyama-Martin et al., "A Multi-Standard Low Power 1.5-3.125 Gb/s Serial Transceiver in 90nm CMOS," IEEE Custom Integrated Circuits Conference 2006, 2006, pp. 401-404, doi: 10.1109/CICC.2006.320970.
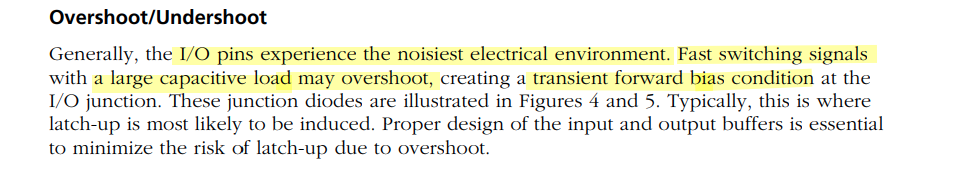
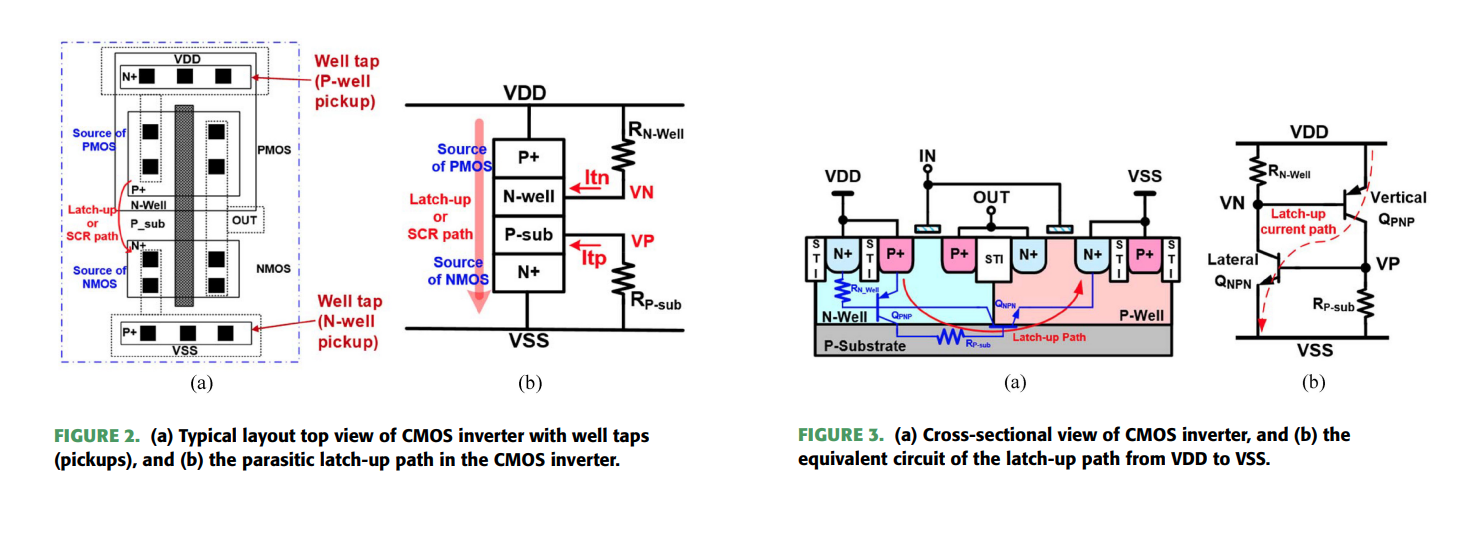
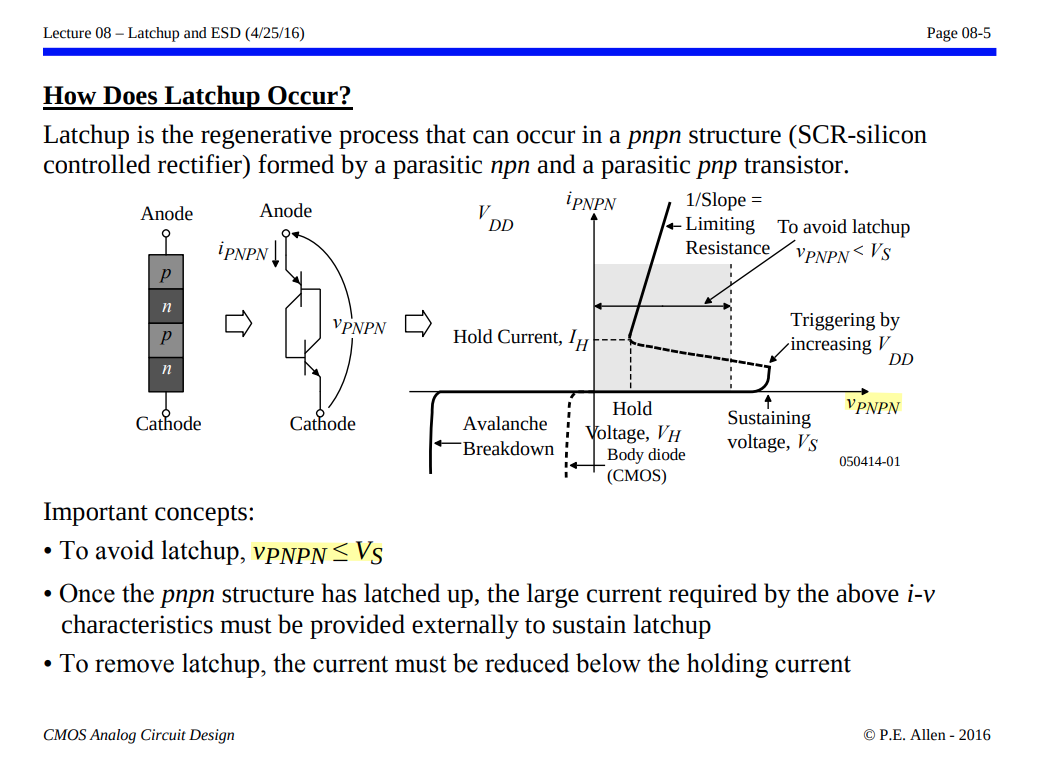
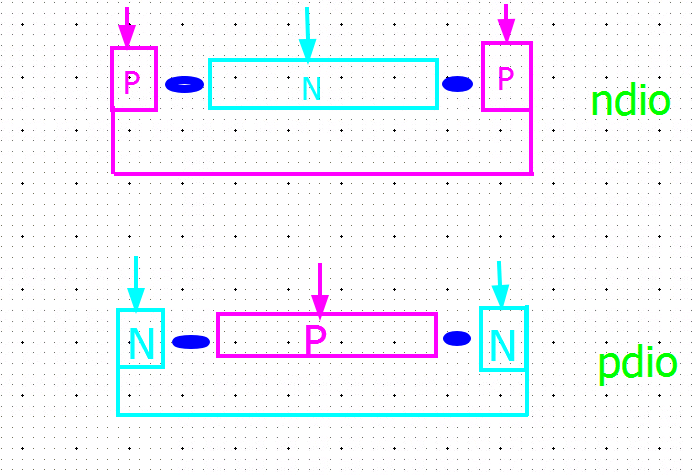
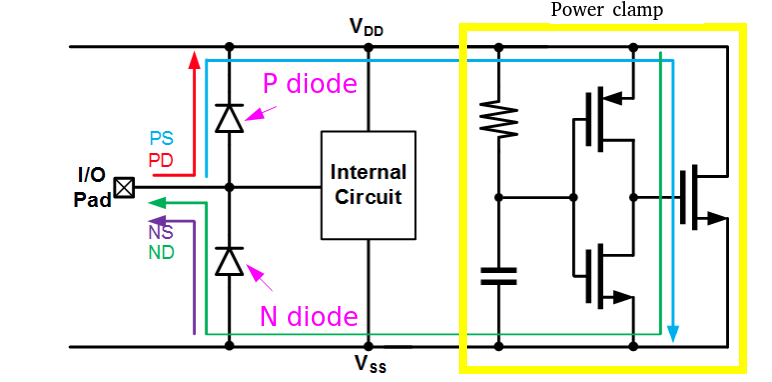
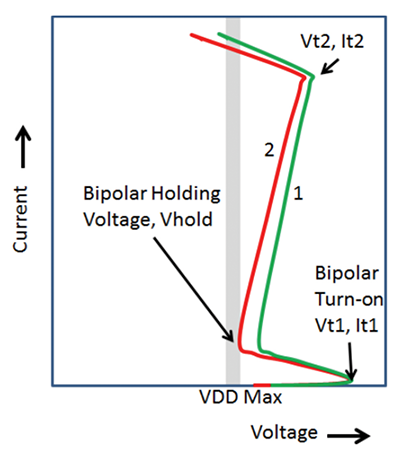
Power/Ground and I/O Pins
Power / Ground Pin Information
In both digital and analog I/O, power and ground pins appear at the sub-circuit definiton, allowing user to use the I/O in voltage islands. They follow certain naming conventions.
- digital I/O sub-circuit
- VDD: pre-driver core voltage (supplied by PVDD1CDGM)
- VSS: pre-driver ground and also global ground (supplied by PVDD1CDGM)
- VDDPST: I/O post-driver voltage, i.e. 1.8V (supplied by PVDD2CDGM or PVDD2POCM)
- VSSPOST: I/O post-driver ground (supplied by PVDD2CDGM or PVDD2POCM)
- POCCTRL: POCCTRL signal (supplied by PVDD2POCM)
- analog I/O placed in a core voltage domain, the convention is
- TACVDD: analog core voltage (supplied by PVDD3ACM)
- TACVSS: analog core ground (supplied by PVDD3ACM)
- VSS: global core ground
- analog I/O placed in an I/O voltage domain, the convention is:
- TAVDD: analog I/O voltage, i.e. 1.8V (supplied by PVDD3AM)
- TAVSS: analog I/O ground (supplied by PVDD3AM)
- VSS: global core ground
Power/Ground Combo Cells
| power/ground combo pad cell | pins to be connected to bump | to core side pin name |
|---|---|---|
| PVDD1CDGM | VDD VSS | VDD VSS |
| PVDD2CDGM PVDD2POCM | VDDPST VSSPST | N/A |
| PVDD3AM | TAVDD TAVSS | AVDD AVSS |
| PVDD3ACM | TACVDD TACVSS | AVDD AVSS |
Note for the retention mode
- At initial state, IRTE must be 0 when VDD is off.
- IRTE must be kept >= 10us after VDD turns on again (from the retention mode to the normal operation mode).
- IRTE can be switched only when both VDD and VDDPST are on.
When the rention function is needed, IRTE signal must come from an "always-on" core power domain. If you don't need the rention function, it is required to tie IRTE to ground. In other words, no matter the rention feature is needed or not, it is required to have PCBRTE in each domain.
Note: PCBRTE does not need PAD connection.
Internal Pins
There are 3 internal global pins, i.e. ESD, POCCTRL, RTE, in all digital domain cells.
In real application,
- ESD pin is an internal signal and active in ESD event happening
- POCCTRL is an internal signal and active in Power-on-control event.
However, these special events (i.e. ESD event and Power-on-control event) are not modeled in NLDM kit (.lib), only normal function is covered, so ESD and POCCTRL pins are simply defined as ground in NLDM kit (.lib).
These 3 global pins will be connected automatically after cell-to-cell abutting in physical layout.
Power-Up sequence in Digital Domain
Power up the I/O power (VDDPST) first, then the core power (VDD)
- PVDDD2POCM cell would generate Power-On-Control signal (POCCTRL) to have the post-driver NMOS and PMOS off, so that the crowbar current would not occur in the post-driver fingers when the I/O voltage is on while the core voltage remains off. As such, I/O cell would be in the Hi-Z state. when POCCTRL is on, the pll-up/down resistor is disabled and C is 0.
- The POCCTRL signal is transmitted to I/O cells through cell abutment. There is no need to have routing for POCCTTRL nor give a control signal to the POCCTRL pin any of I/O cells. Note that the POCCTRL signal would be cut if inserting a power-cut (PRCUT) cell.
Power-Down sequence in Digital Domain
It's the reverse of power-up sequence.
Use model in Innovus
1 | set init_gnd_net "vss_core vss DUMMY_ESD DUMMY_POCCTRL" |
1 | set pins [get_object_name [get_ports *]] |
reference
M. Tian, V. Visvanathan, J. Hantgan and K. Kundert, "Striving for small-signal stability," in IEEE Circuits and Devices Magazine, vol. 17, no. 1, pp. 31-41, Jan. 2001, doi: 10.1109/101.900125.
Open loop gain analysis and "STB" method [https://www.linkedin.com/pulse/open-loop-gain-analysis-stb-method-jean-francois-debroux]
The Analog Designer's Toolbox (ADT) | Invited Talk by IEEE Santa Clara Valley Section CAS Society, https://youtu.be/FT6kKC5OdE0
ESSCIRC2023 Circuit Insights Ali Sheikholeslami [https://youtu.be/2xFIZM5_FPw?si=XWwSzDgKWZGB0rX1]
Ali Sheikholeslami, Circuit Intuitions: Thevenin and Norton Equivalent Circuits, Part 3 IEEE Solid-State Circuits Magazine, Vol. 10, Issue 4, pp. 7-8, Fall 2018.
—, Circuit Intuitions: Thevenin and Norton Equivalent Circuits, Part 2 IEEE Solid-State Circuits Magazine, Vol. 10, Issue 3, pp. 7-8, Summer 2018.
—, Circuit Intuitions: Thevenin and Norton Equivalent Circuits, Part 1 IEEE Solid-State Circuits Magazine, Vol. 10, Issue 2, pp. 7-8, Spring 2018.
—, Circuit Intuitions: Miller's Approximation IEEE Solid-State Circuits Magazine, Vol. 7, Issue 4, pp. 7-8, Fall 2015.
—, Circuit Intuitions: Miller's Theorem IEEE Solid-State Circuits Magazine, Vol. 7, Issue 3, pp. 8-10, Summer 2015.
Shanthi Pavan, "Demystifying Linear Time Varying Circuits"
ecircuitcenter. Switched-Capacitor Resistor [http://www.ecircuitcenter.com/Circuits/SWCap/SWCap.htm]
Jørgen Andreas Michaelsen. INF4420 Switched-Capacitor Circuits. [https://www.uio.no/studier/emner/matnat/ifi/INF4420/v13/undervisningsmateriale/inf4420_v13_07_switchedcapacitor_print.pdf]
chembiyan T. OC Lecture 10: A very basic introduction to switched capacitor circuits [https://youtu.be/SaYtemYp4rQ?si=q2qovTKJrLy65pnu
Robert Bogdan Staszewski, Poras T. Balsara. "All‐Digital Frequency Synthesizer in Deep‐Submicron CMOS"
Mayank Parasrampuria, Sandeep Jain, Burn-in 101 [link]
Kevin Zheng. Circuit Artists [https://circuit-artists.com/posts/]