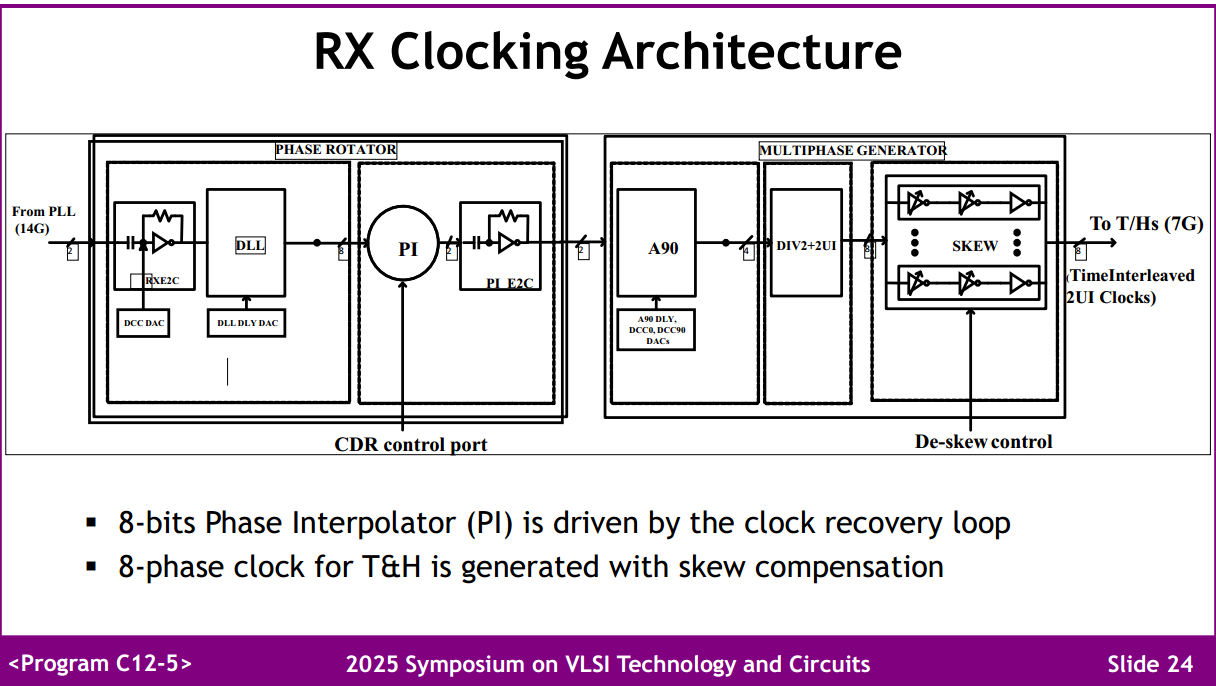
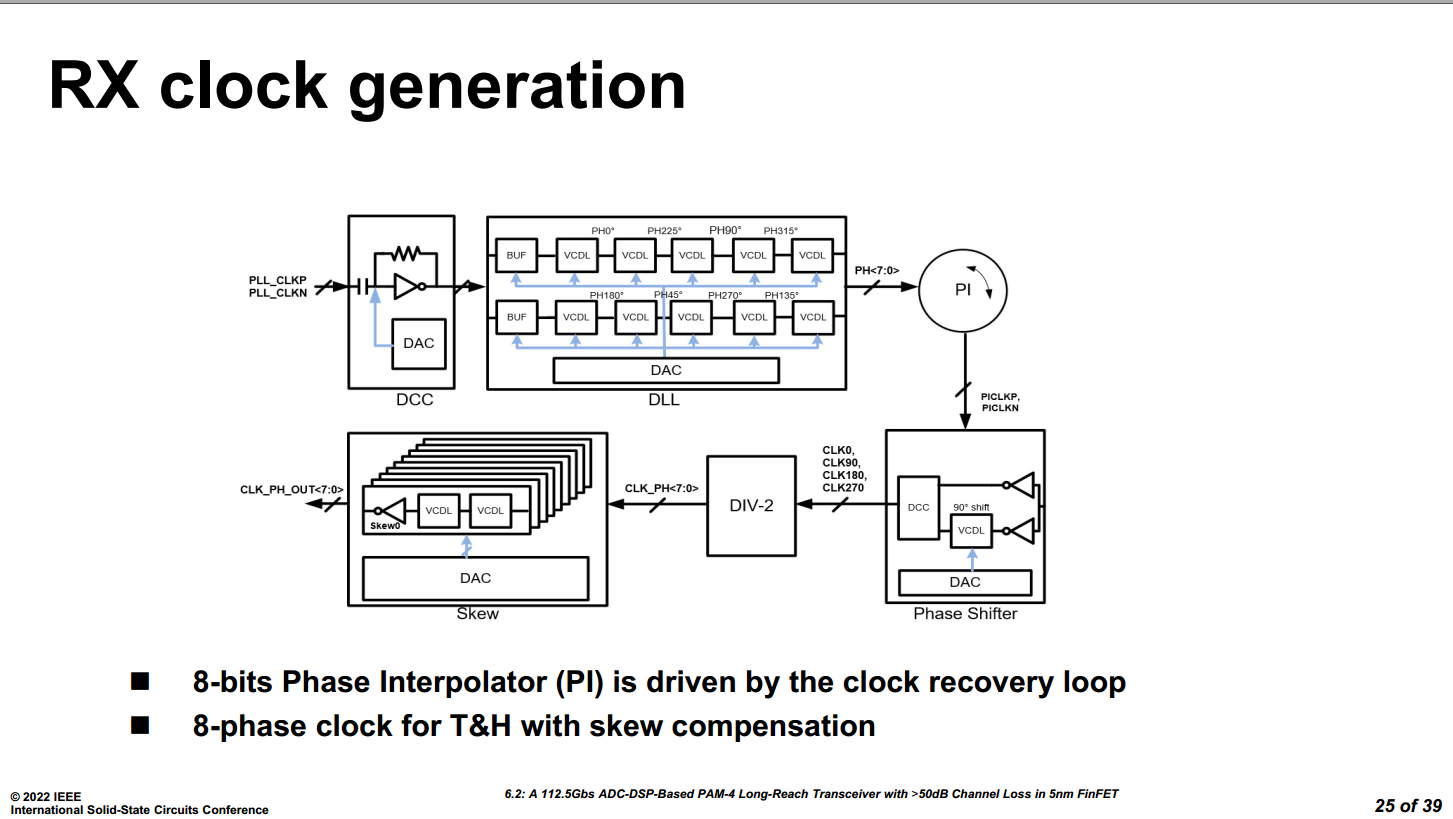
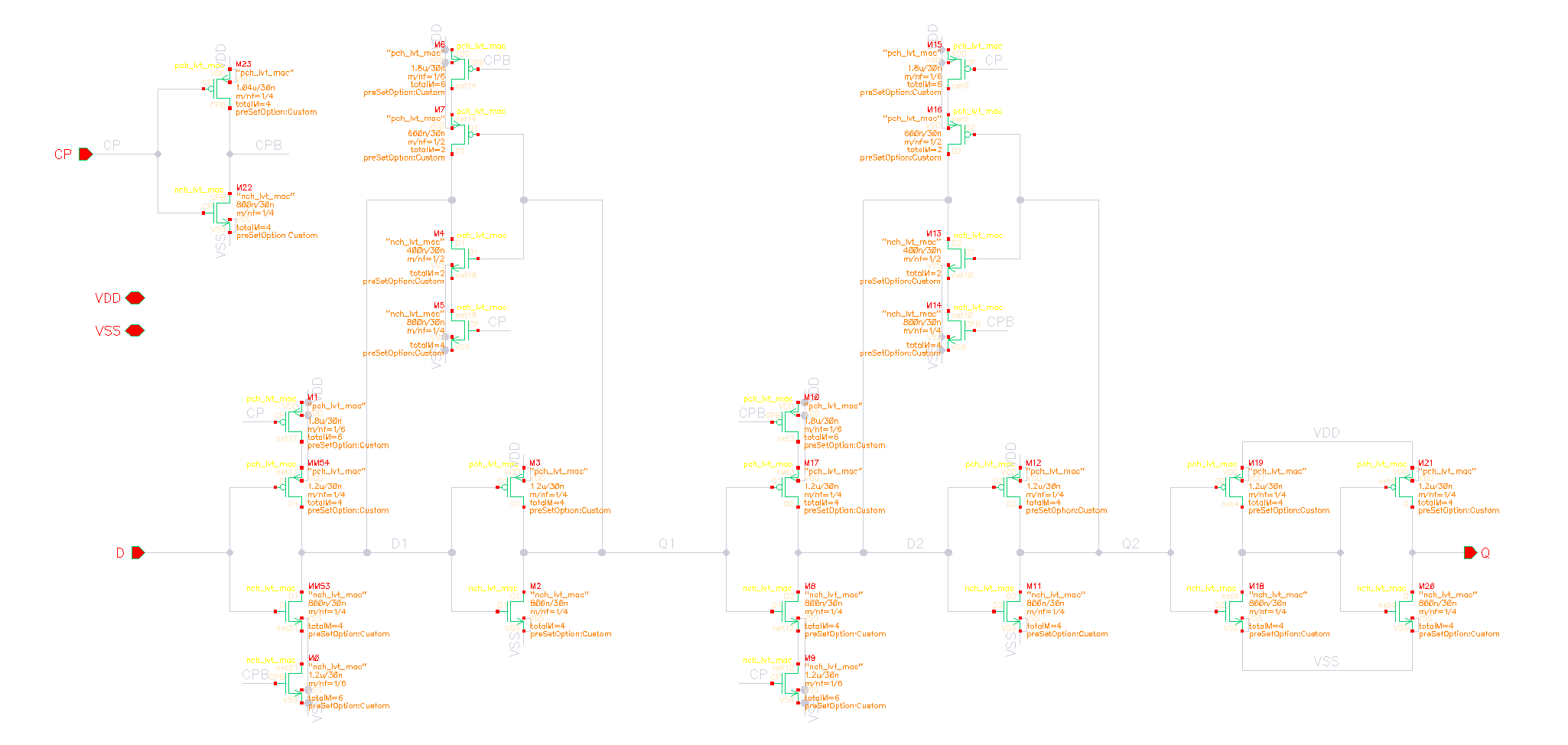
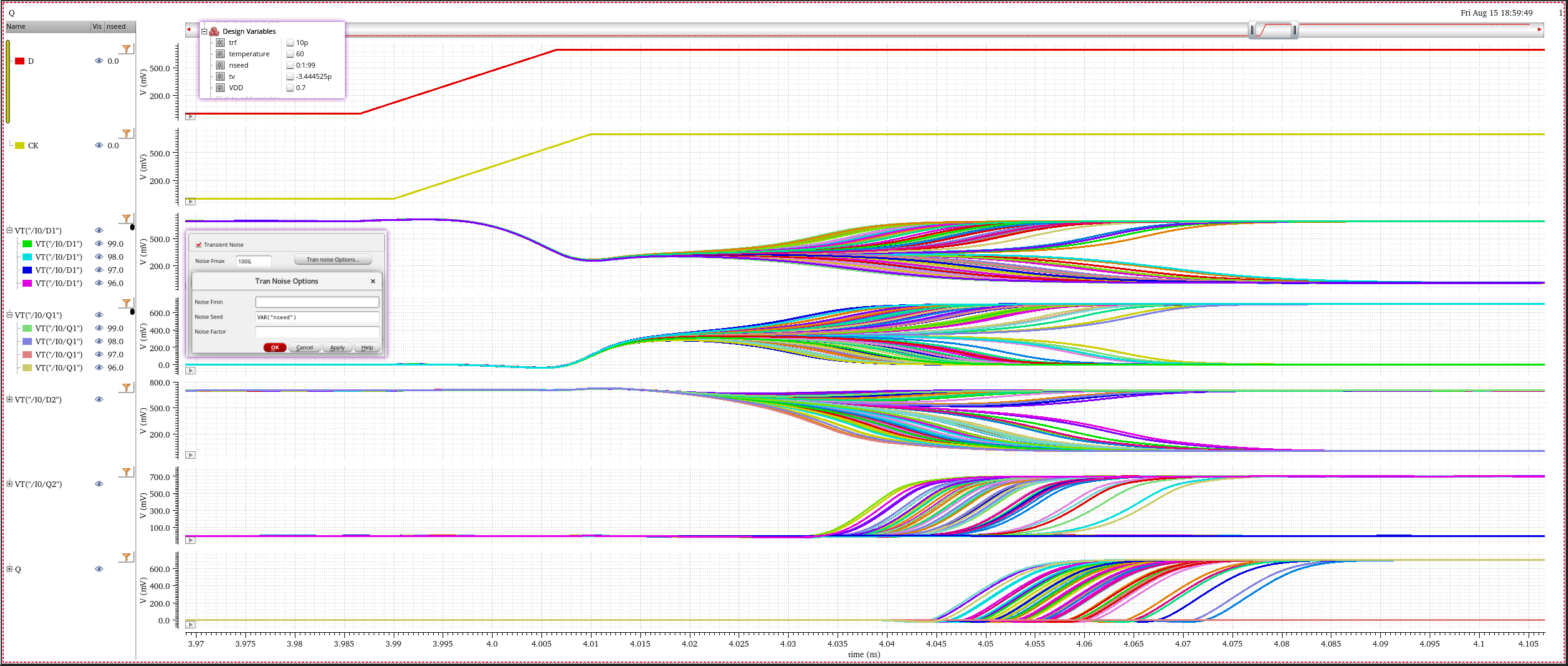
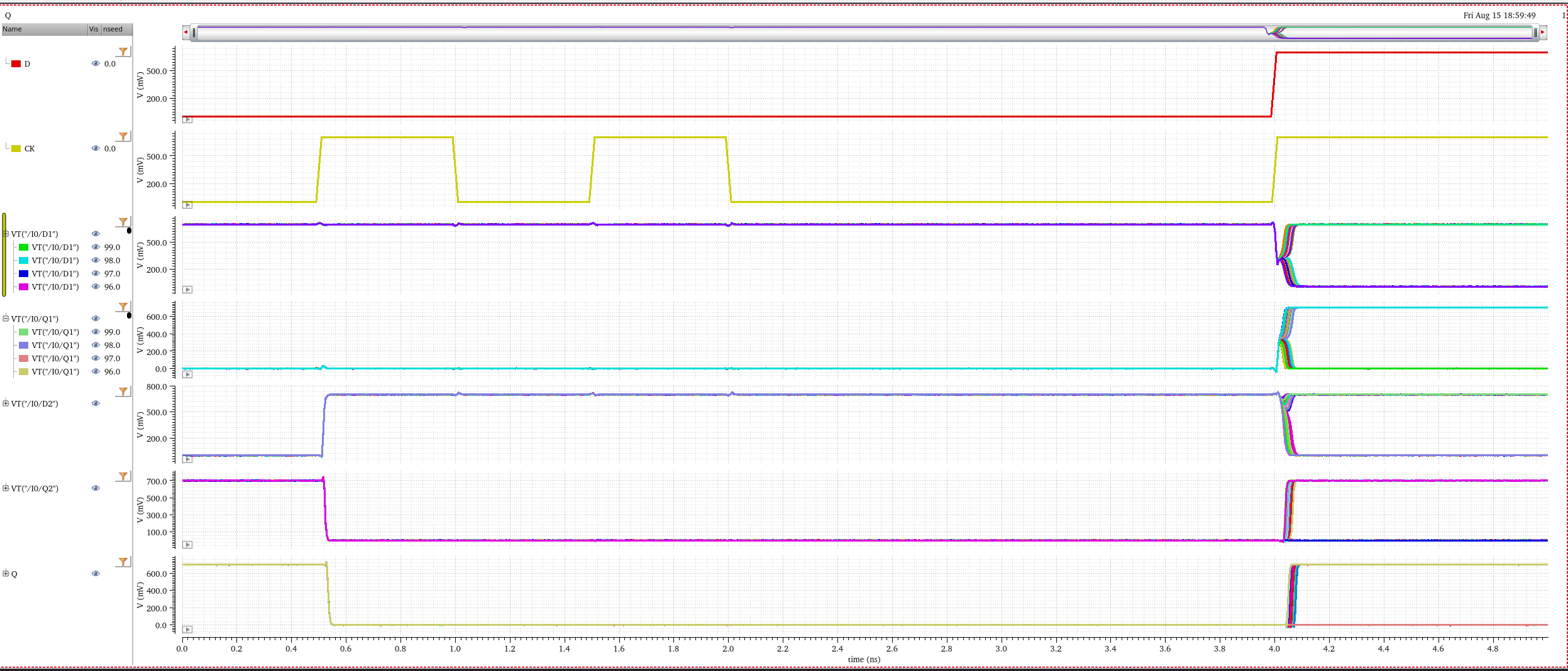
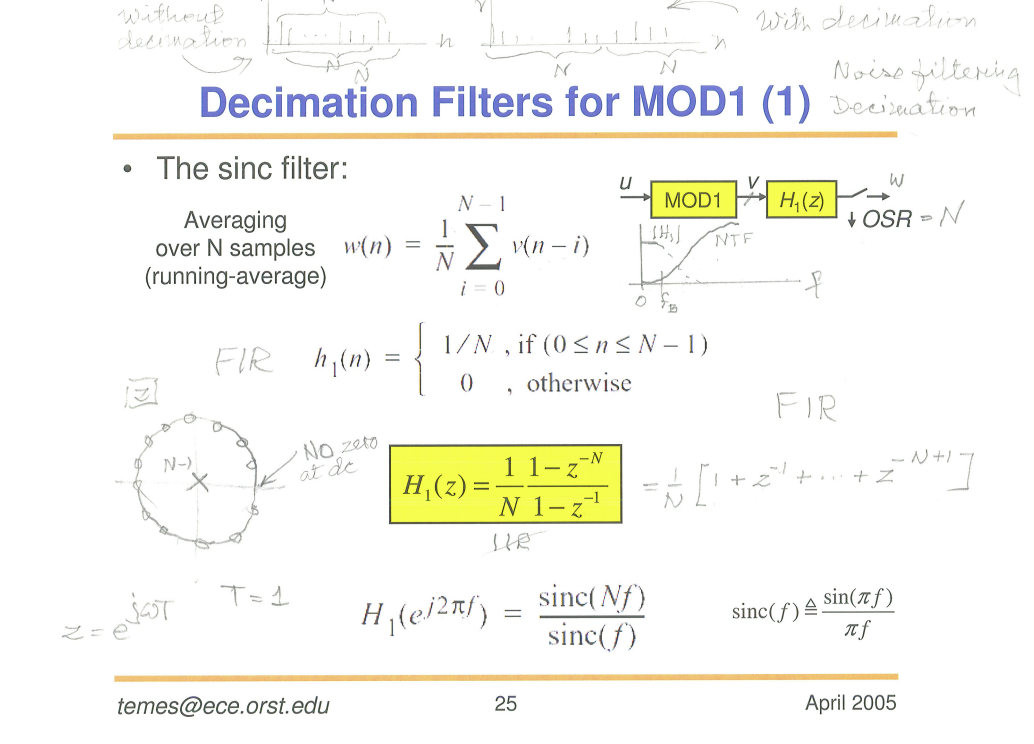
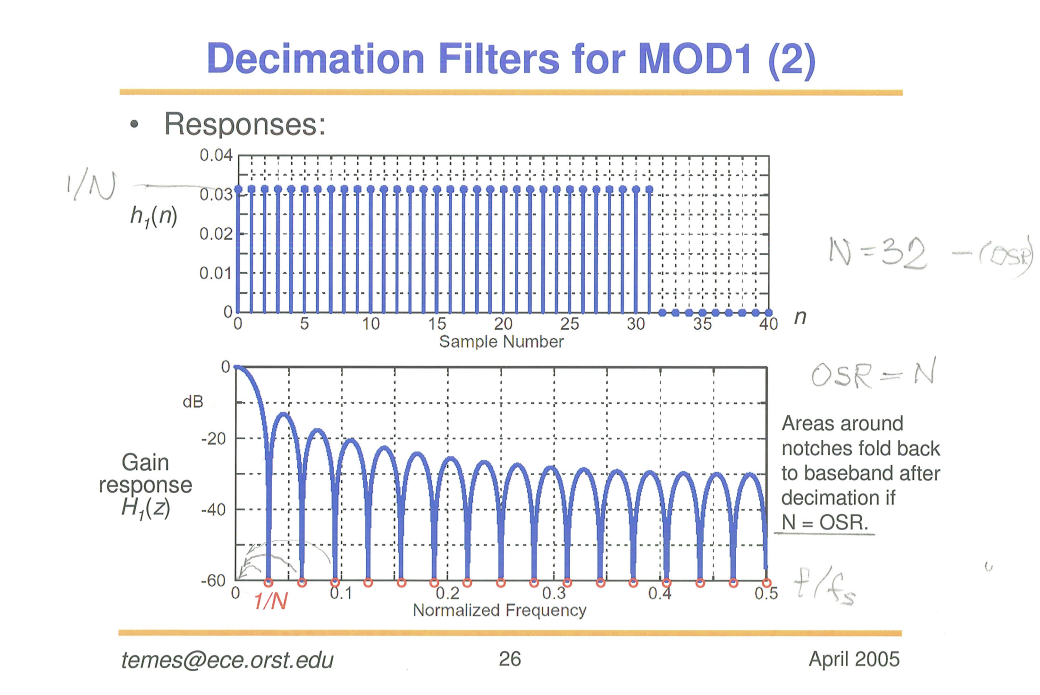
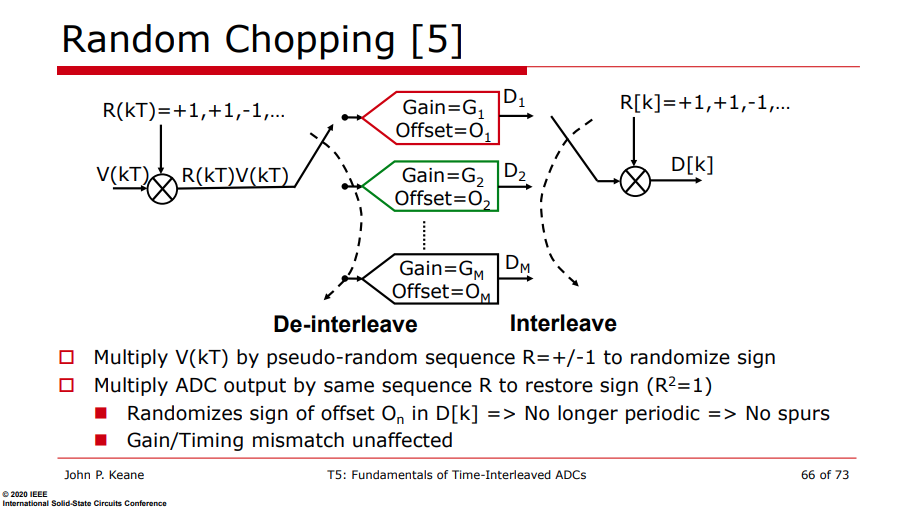
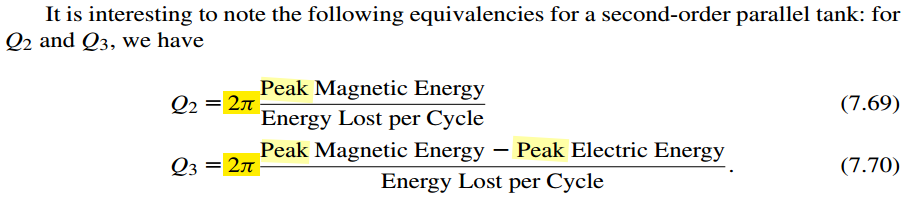LC Tank

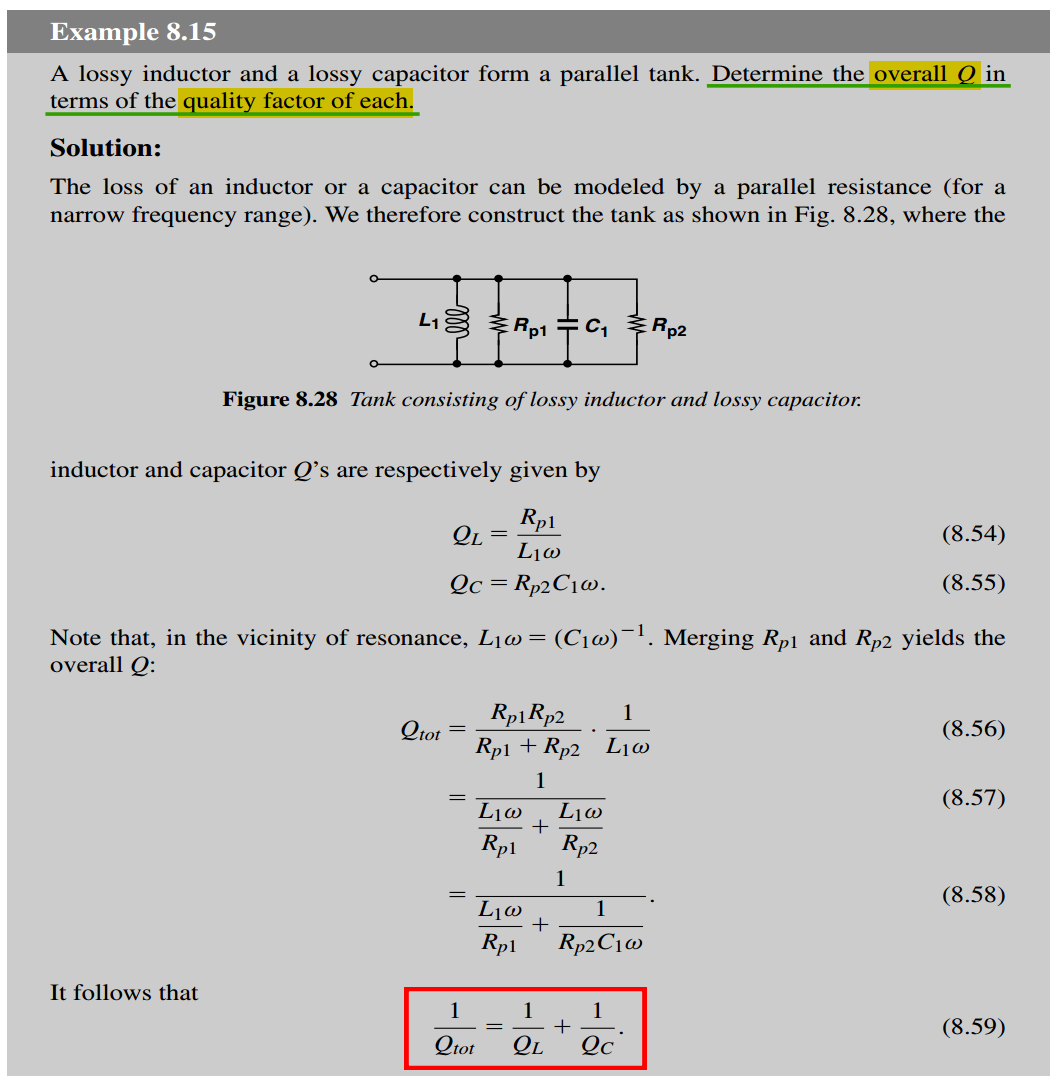
Definitions of Q

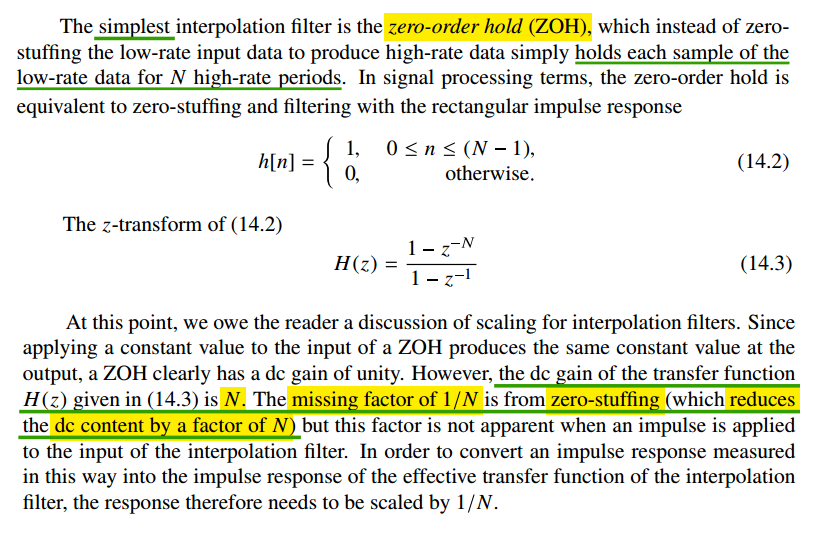
Assuming RLC oscillator waveform is \(V_0\sin\omega_0 t\) and \(\omega_0 = \frac{1}{\sqrt{LC}}\) is
resonance frequency. suppose the current through \(R\) is cancelled out by additional \(-R\)
Energy stored \[
E_t = \frac{1}{2}LI_0^2 =
\frac{1}{2}L(C\omega_0V_0)^2=\frac{1}{2}LC^2\omega_0^2 V_0^2 =
\frac{1}{2}CV_0^2
\] Energy Dissipated per Cycle \[
E_d = \frac{V_0^2}{2R}\frac{2\pi}{\omega_0}
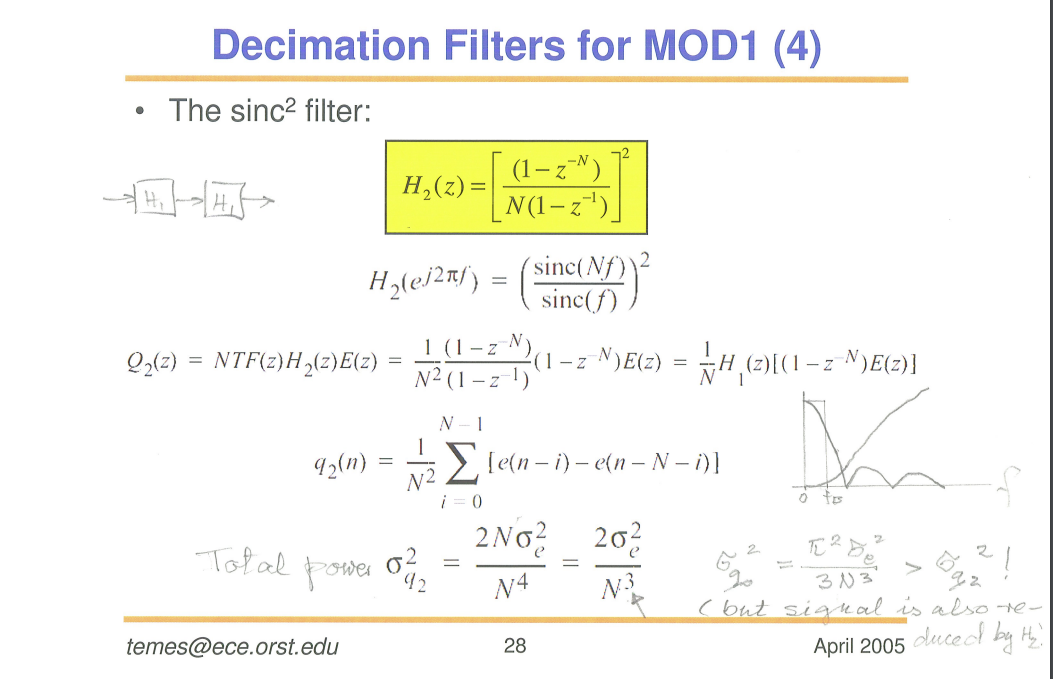
\] For \(Q_4\) \[
Q_4 = 2\pi\frac{E_s}{E_d} = R\omega_0C = \frac{R}{\omega_0L}
\]
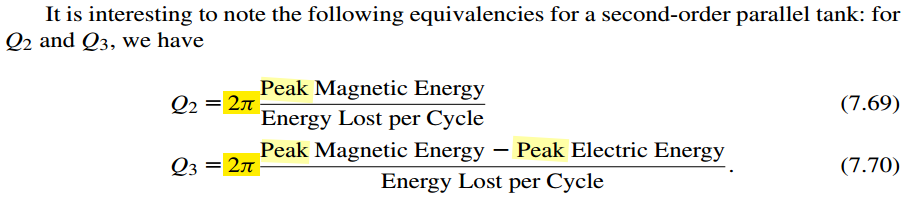
For \(Q_3\), suppose RLC tank is
driven by \(V_o\cos \omega t\) voltage
source, then
Peak Magnetic Energy \[
E_{pL} = \frac{1}{2}LI_0^2 =
\frac{1}{2}L\left(\frac{V_0}{L\omega}\right)^2
\] Peak Electric Energy \[
E_{pC} = \frac{1}{2}CV_0^2
\] with Energy Lost per Cycle \(E_d =
\frac{V_0^2}{2R}\frac{2\pi}{\omega_0}\), we have \[
Q_3 = \frac{E_{pL} - E_{pC}}{E_d} =
\left(\frac{1}{L\omega^2}-C\right)R\omega=\frac{R}{L\omega}\left(1 -
\frac{\omega^2}{\omega^2_{SR}}\right)
\]
Makarov, Sergey & Ludwig, Reinhold & Bitar, Joyce. (2016).
Practical Electrical Engineering. 10.1007/978-3-319-21173-2. [pdf]
TODO 📅
Capacitor Bank
B. Sadhu and R. Harjani, "Capacitor bank design for wide tuning range
LC VCOs: 850MHz-7.1GHz (157%)," Proceedings of 2010 IEEE International
Symposium on Circuits and Systems, Paris, France, 2010 [https://sci-hub.st/10.1109/ISCAS.2010.5537040]
TODO 📅
Non ideal capacitor &
inductor
Tank Circuits/Impedances [https://stanford.edu/class/ee133/handouts/lecturenotes/lecture5_tank.pdf]
Resonant Circuits [https://web.ece.ucsb.edu/~long/ece145b/Resonators.pdf]
Series & Parallel Impedance Parameters and Equivalent Circuits
[https://assets.testequity.com/te1/Documents/pdf/series-parallel-impedance-parameters-an.pdf]
ES Lecture 35: Non ideal capacitor, Capacitor Q and series RC to
parallel RC conversion [https://youtu.be/CJ_2U5pEB4o?si=4j4CWsLSapeu-hBo]
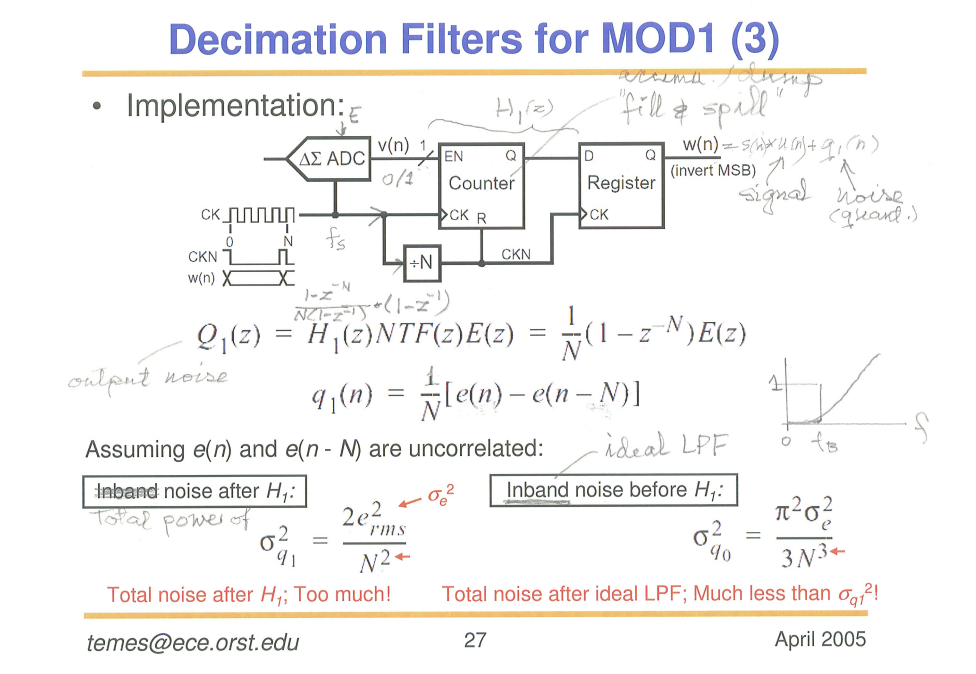
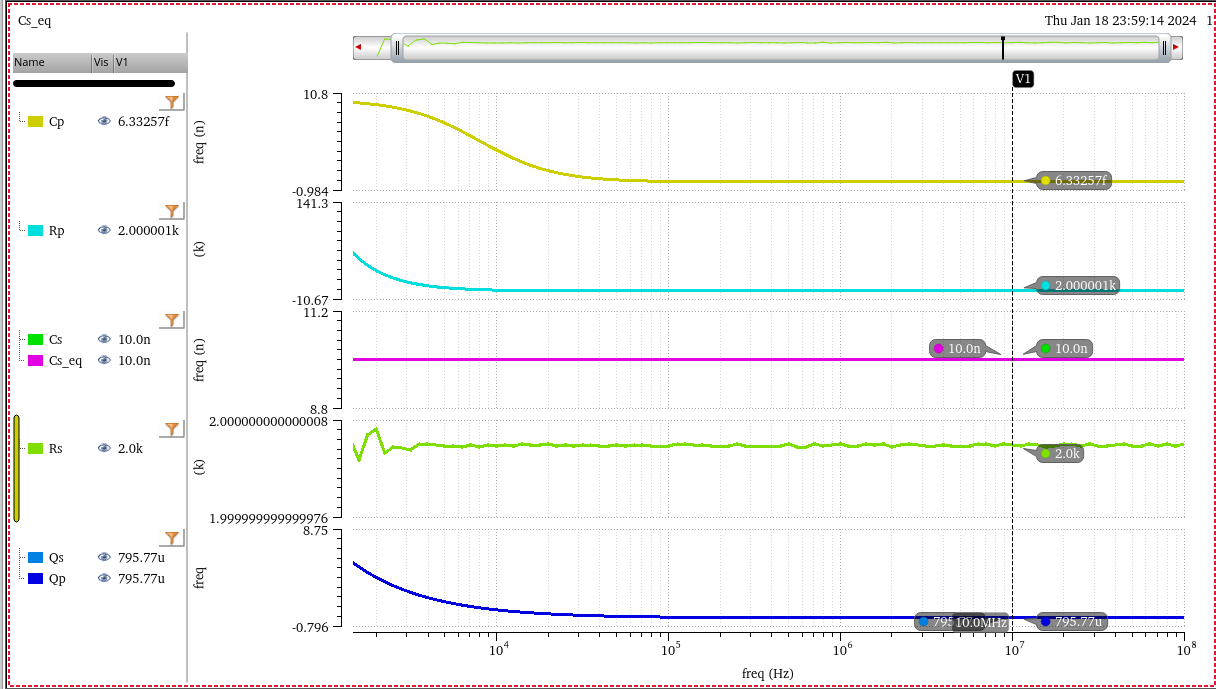
Capacitor
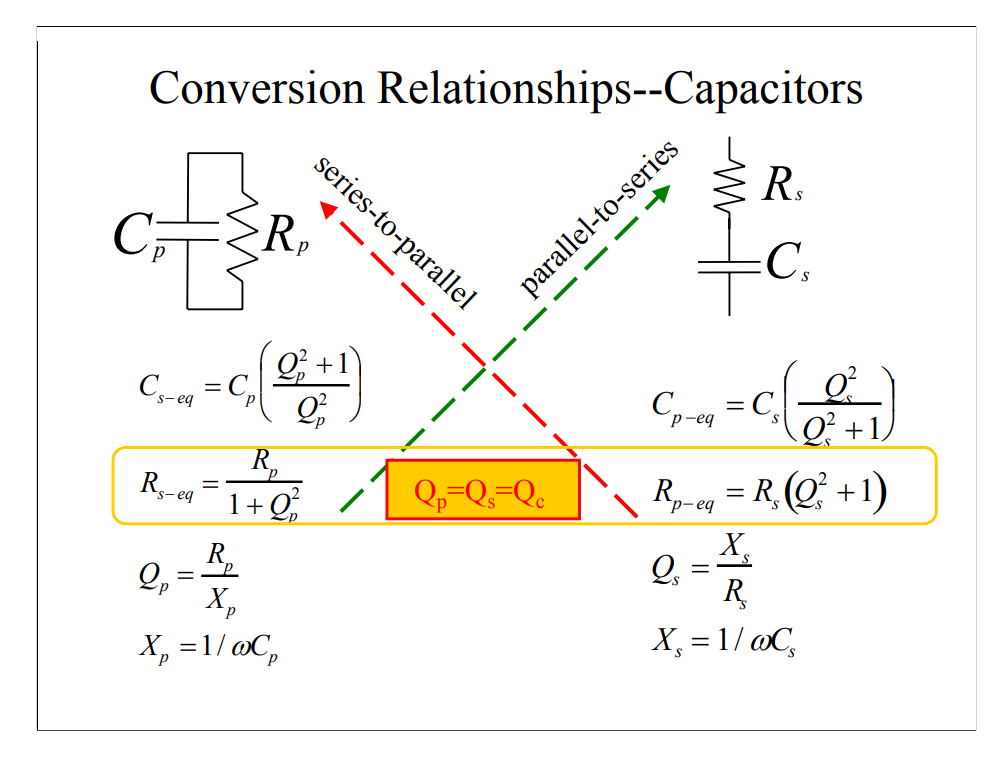
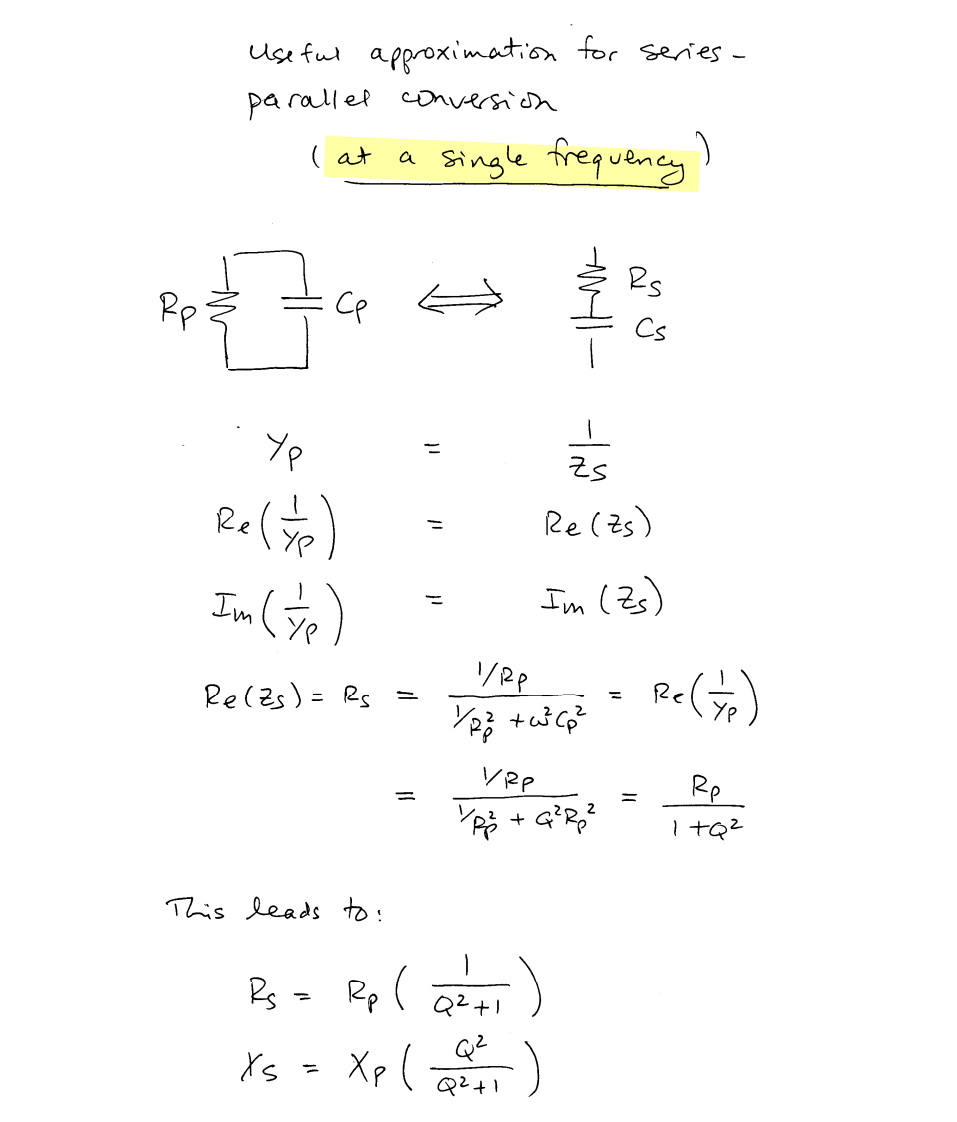

\[
Q_s = \frac{X_s}{R_s} = X_p\frac{Q_p^2}{Q_p^2+1}\cdot
\frac{Q_p^2+1}{R_p} =\frac{Q_p^2}{R_p/X_p}=Q_p
\]
So long as \(Q_s\gg 1\) \[\begin{align}
R_p &\approx Q_s^2R_s \\
C_p &\approx C_s
\end{align}\]
Inductor

So long as \(Q_s\gg 1\) \[\begin{align}
R_p &\approx Q_s^2R_s \\
L_p &\approx L_s
\end{align}\]
Tail filter
D. Murphy, H. Darabi and H. Wu, "Implicit Common-Mode Resonance in LC
Oscillators," in IEEE Journal of Solid-State Circuits, vol. 52, no. 3,
pp. 812-821, March 2017, [https://sci-hub.st/10.1109/JSSC.2016.2642207]
M. Shahmohammadi, M. Babaie and R. B. Staszewski, "A 1/f Noise
Upconversion Reduction Technique for Voltage-Biased RF CMOS
Oscillators," in IEEE Journal of Solid-State Circuits, vol. 51, no. 11,
pp. 2610-2624, Nov. 2016 [pdf]
M. Babaie, M. Shahmohammadi, R. B. Staszewski, (2019) "RF CMOS
Oscillators for Modern Wireless Applications" River Publishers [https://www.riverpublishers.com/pdf/ebook/RP_E9788793609488.pdf]
TODO 📅

P. Liu et al., "A 128Gb/s ADC/DAC Based PAM-4 Transceiver with
>45dB Reach in 3nm FinFET," 2025 Symposium on VLSI Technology and
Circuits (VLSI Technology and Circuits), Kyoto, Japan, 2025
E. Hegazi, H. Sjoland and A. Abidi, "A filtering technique to lower
oscillator phase noise," 2001 IEEE International Solid-State
Circuits Conference. Digest of Technical Papers. ISSCC (Cat.
No.01CH37177), San Francisco, CA, USA, 2001 [paper,
slides]
—, "A filtering technique to lower LC oscillator phase noise," in
IEEE Journal of Solid-State Circuits, vol. 36, no. 12, pp.
1921-1930, Dec. 2001 [https://people.engr.tamu.edu/spalermo/ecen620/filtering_tech_lc_osc_hegazi_jssc_2001.pdf]
—, "25.3 A VCO with implicit common-mode resonance," 2015 IEEE
International Solid-State Circuits Conference - (ISSCC) Digest of
Technical Papers, San Francisco, CA, USA, 2015 [https://sci-hub.st/10.1109/ISSCC.2015.7063116]
Lecture 16: VCO Phase Noise [https://people.engr.tamu.edu/spalermo/ecen620/lecture16_ee620_vco_pn.pdf]
reference
P. Andreani and A. Bevilacqua, "Harmonic Oscillators in CMOS—A
Tutorial Overview," in IEEE Open Journal of the Solid-State Circuits
Society, vol. 1, pp. 2-17, 2021 [pdf]
A. A. Abidi and D. Murphy, "How to Design a Differential CMOS LC
Oscillator," in IEEE Open Journal of the Solid-State Circuits Society,
vol. 5, pp. 45-59, 2025 [https://ieeexplore.ieee.org/stamp/stamp.jsp?arnumber=10818782]
Pietro Andreani. ISSCC 2011 T1: Integrated LC oscillators [slides,transcript]
—. ISSCC 2017 F2: Integrated Harmonic Oscillators
—. SSCS Distinguished Lecture: RF Harmonic Oscillators Integrated in
Silicon Technologies [https://www.ieeetoronto.ca/wp-content/uploads/2020/06/DL-Toronto.pdf]
—. ESSCIRC 2019 Tutorials: RF Harmonic Oscillators Integrated in
Silicon Technologies [https://youtu.be/k1I9nP9eEHE?si=fns9mf3aHjMJobPH]
—. "Harmonic Oscillators in CMOS—A Tutorial Overview," in IEEE Open
Journal of the Solid-State Circuits Society, vol. 1, pp. 2-17, 2021 [pdf]
C. Samori, "Tutorial: Understanding Phase Noise in LC VCOs," 2016
IEEE International Solid-State Circuits Conference (ISSCC), San
Francisco, CA, USA, 2016
—, "Understanding Phase Noise in LC VCOs: A Key Problem in RF
Integrated Circuits," in IEEE Solid-State Circuits Magazine,
vol. 8, no. 4, pp. 81-91, Fall 2016 [https://sci-hub.se/10.1109/MSSC.2016.2573979]
—, Phase Noise in LC Oscillators: From Basic Concepts to Advanced
Topologies [https://www.ieeetoronto.ca/wp-content/uploads/2020/06/DL-VCO-short.pdf]
Jun Yin. ISSCC 2025 T10: mm-Wave Oscillator Design
Razavi, Behzad. RF Microelectronics. 2nd ed. Prentice Hall, 2012. [pdf]